A small loop of area of cross -section is lying concentrically and coplanar inside a bigger loop of radius . A current of is passed in the bigger loop. The smaller loop is rotated about its diameter with an angular velocity . The magnetic flux linked with the smaller loop will be
The correct Answer is:D
Step by step video, text & image solution for A small loop of area of cross -section 10^(-4)m^(2) is lying concentrically and coplanar inside a bigger loop of radius 0.628m. A current of 10A is passed in the bigger loop. The smaller loop is rotated about its diameter with an angular velocity omega. The magnetic flux linked with the smaller loop will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITIVE THINKING
158 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EVALUATION TEST
8 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EVALUATION TEST
8 Videos
- ELASTICITYBOOK - TARGET PUBLICATIONCHAPTER - ELASTICITYEXERCISE - Evaluation test
14 Videos
- ELECTROSTATICSBOOK - TARGET PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - EVALUATION TEST
14 Videos
Similar Questions
A very small circular loop of area 5×I0−4m2 , resistance 2Ω and negligible inductance is initially coplanar and concentric with a much larger fixed circular loop of radius 0.Im . A constant current of IA is passed in the bigger loop and the smaller loop is rotated with angular velocity ωrad/sec about a diameter. Calculate (a) the flux limked with the smaller loop, (b) indced emf (c) induced current in the smaller loop, as a function of time.
View SolutionA very small circular loop of radius r is initially coplanar and concentric with a much larger circular loop of radius R(>>r) . A constant current i is passed in the larger loop which is kept fixed in space and the small loop is rotated with angular velocity ω about a diameter. The resistance of the small loop is R0 and the inductance is negligible.
(a) Find the current in the small loop as a function of time.
(b) Calculate how much troque must be exerted on the small loop to rotate it.
View Solution
Knowledge Check
A circular loop is kept perpendicular to a uniform magnetic field. Loop is rotated about its diameter with constant angular velocity.
AMagnitude of emf induced will be minimum when magnitude of flux is maximumBMagnitude of emf is maximum when magnitude of flux is minimumCPhase difference between emf and flux is π2DNone of theseA very small circular loop of radius a is initially (at t = 0) coplanar and concentric with a much larger fixed circular loop of radius b. A constant current I flows in the larger loop. The smaller loop is rotated with a constant angular speed ω about the common diameter. The emf induced in the smaller loop as a function of time t is
Aπa2μ0I2bωcos(ωt)Bπa2μ0I2bωsin(ω2t2)Cπa2μ0I2bωsin(ωt)Dπa2μ0I2bωsin2(ωt)A very small circular loop of radius a is initially (at t = 0) coplanar and concentric with a much larger fixed circular loop of radius b. A constant current I flows in the larger loop. The smaller loop is rotated with a constant angular speed ω about the common diameter. The emf induced in the smaller loop as a function of time t is
Aπa2μ0I2bωcosωtBπa2μ0I2bωsinω2t2Cπa2μ0I2bωsinωtDπa2μ0I2bωsin2ωt
A very small circular loop of radius a is initially coplanar & concentric with a much larger circular loop of radius b(<<a).A constant current I is passed in the large loop which is kept fixed in space & the small loop is rotated with constant angular velocity ω about a diameter.The resistance of the small loop is R & its inductance is negligible The induced emf in the large loop due to current induced in smaller loop as a function of time is equal to 1x(πa2μ0ωb)2Icos2ωtR.Find out value of x.
View SolutionThe linear loop has an area of 5×10−4m2 and a resistance of 2Ω. The larger circular loop is fixed and has a radius of 0.1m. Both the loops are concentric and co-planner. The smaller loop is rotated with an angular velocity ωrads−1 about its dismeter. The magnetic flux with the smaller loop is

View SolutionA very small circular loop of radius a is initially coplanar & concentric with a much larger circular loop of radius b (>>a). A constant current is passed in the large loop which is kept fixed in space & the small loop is rotated with angular velocity omega about a diameter. The resistance of the small loop is R & its self inductance is negligible. The current in the larger loop is clockwise.
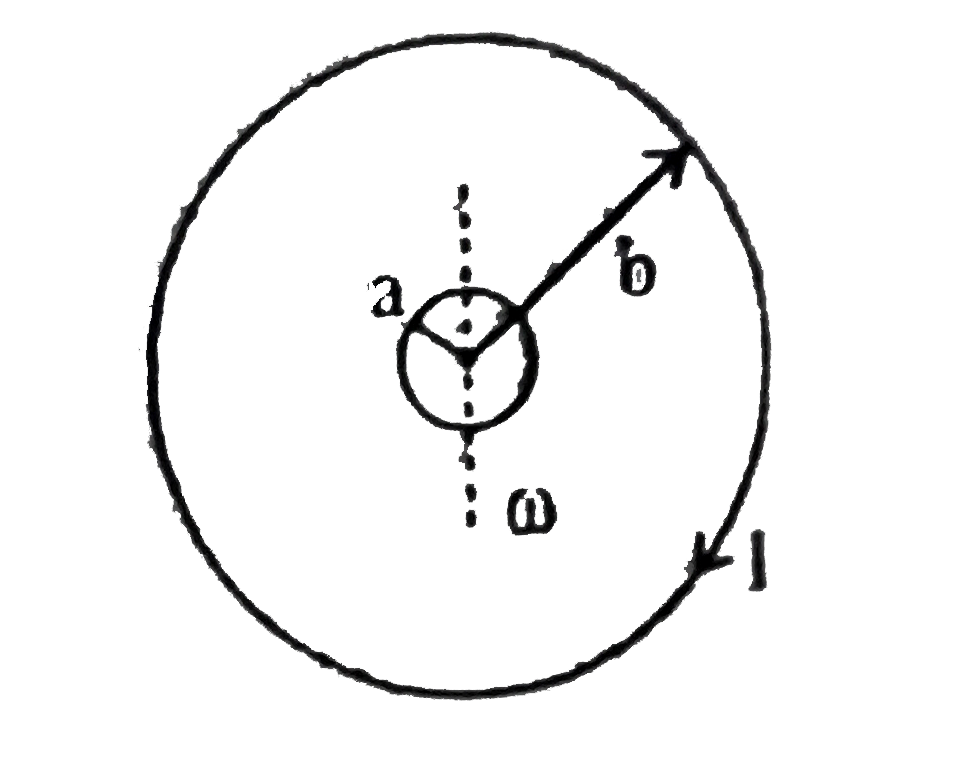
Calculate how much torque must be exerted on the small loop to rotate it.
View SolutionA very small circular loop of radius a is initially coplanar & concentric with a much larger circular loop of radius b (>>a). A constant current is passed in the large loop which is kept fixed in space & the small loop is rotated with angular velocity omega about a diameter. The resistance of the small loop is R & its self inductance is negligible. The current in the larger loop is clockwise.
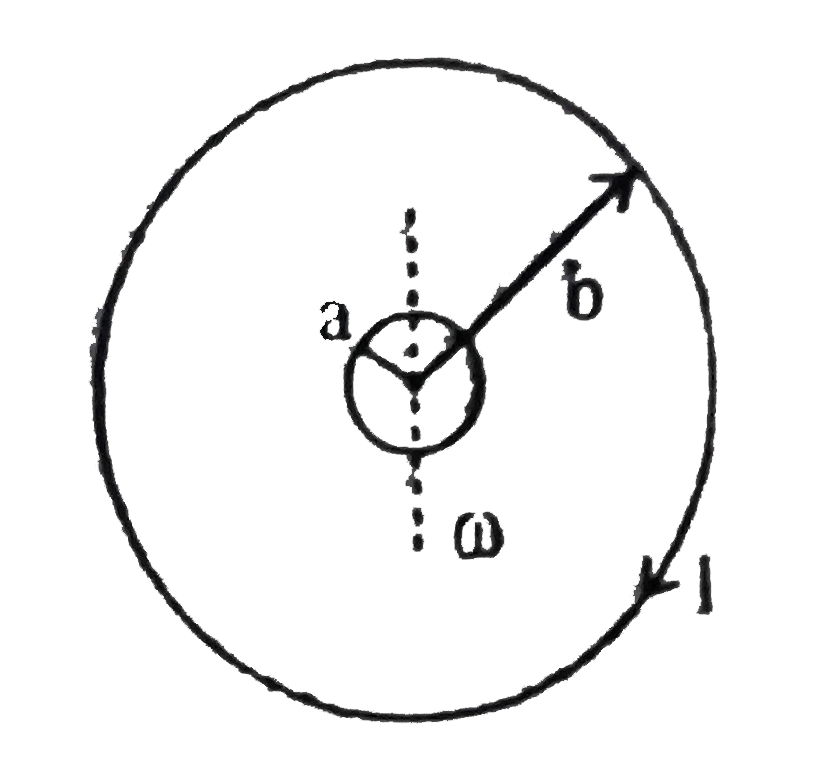
Find the current in the small loop as a function of time.
View SolutionA very small circular loop of radius a is initially coplanar & concentric with a much larger circular loop of radius b (>>a). A constant current is passed in the large loop which is kept fixed in space & the small loop is rotated with angular velocity omega about a diameter. The resistance of the small loop is R & its self inductance is negligible. The current in the larger loop is clockwise.
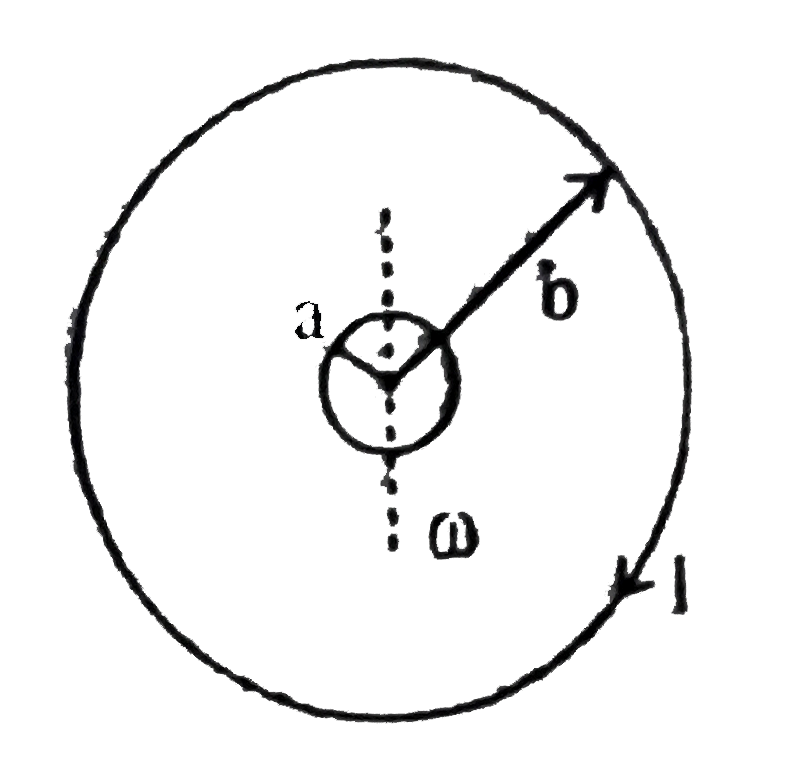
At the moment both the loops are in the same plane
View Solution
