A square loop of side 22cm is converted into circular loop in 0.4s. A uniform magnetic field of 0.2Tdirected normal to the loop, then the induced in the loop is
6.6×10−3V
6.6×10−5V
6.6×10−4V
6.6×10−8V
The correct Answer is:A
To solve the problem step by step, we will calculate the induced electromotive force (emf) in the loop as it changes from a square shape to a circular shape in a uniform magnetic field.
Step 1: Calculate the Area of the Square Loop
The side of the square loop is given as 22cm.
Area of the square loop=side2=(22cm)2=484cm2
Step 2: Convert the Area to Square Meters
To convert the area from square centimeters to square meters, we use the conversion factor 1cm2=10−4m2.
Area in m2=484cm2×10−4=0.0484m2
Step 3: Calculate the Radius of the Circular Loop
Since the square loop is converted into a circular loop, the circumference of the square loop will equal the circumference of the circular loop.
Circumference of the square loop=4×side=4×22cm=88cm
The circumference of the circular loop is given by:
Circumference of the circular loop=2πr
Setting these equal gives:
88cm=2πr
Solving for r:
r=88cm2π=88×10−2m2π≈0.886.2832≈0.140m
Step 4: Calculate the Area of the Circular Loop
Now we can calculate the area of the circular loop using the radius found:
Area of the circular loop=πr2=π(0.140)2≈0.0616m2
Step 5: Calculate the Change in Magnetic Flux
The change in magnetic flux (ΔΦ) is given by:
ΔΦ=B⋅(Area of circular loop−Area of square loop)=0.2T⋅(0.0616m2−0.0484m2)
Calculating the change in area:
ΔΦ=0.2⋅(0.0616−0.0484)=0.2⋅0.0132=0.00264Wb
Step 6: Calculate the Induced EMF
The induced emf (E) can be calculated using Faraday's law of electromagnetic induction:
E=−ΔΦΔt
Given that Δt=0.4s:
E=−0.00264Wb0.4s=−0.0066V=−6.6mV
Thus, the induced emf in the loop is approximately 6.6mV.
Final Answer
The induced emf in the loop is 6.6mV.
---
The side of the square loop is given as 22cm.
To convert the area from square centimeters to square meters, we use the conversion factor 1cm2=10−4m2.
Since the square loop is converted into a circular loop, the circumference of the square loop will equal the circumference of the circular loop.
Now we can calculate the area of the circular loop using the radius found:
The change in magnetic flux (ΔΦ) is given by:
The induced emf (E) can be calculated using Faraday's law of electromagnetic induction:
The induced emf in the loop is 6.6mV.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITIVE THINKING
158 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EVALUATION TEST
8 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EVALUATION TEST
8 Videos
- ELASTICITYBOOK - TARGET PUBLICATIONCHAPTER - ELASTICITYEXERCISE - Evaluation test
14 Videos
- ELECTROSTATICSBOOK - TARGET PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - EVALUATION TEST
14 Videos
Similar Questions
A rectangular loop of sides 8 cm and 2 cm with a small cut is stationary in a uniform magnetic field directed normal to the loop. The magneic field is reduced form its initial value of 0.3 T at the rate of 0.2 T s−1. If the cut is joined and the loop has a resistance of 1.6Ω, how much power is dissipated by the loop as heat ?
View SolutionA rectangular wire loop of sides 8 cm and 2 cm with a small cut is moving out of a region of uniform magnetic field of magnitude 0.3 T directed normal to the loop. What is the emf developed across the cut if the velocity of the loop is 1 cm s1 in a direction normal to the (a) longer side?
(b) shorter side of the loop ? For how long does the induced voltage last in each case ?
View Solution
Knowledge Check
A rectangular loop sides 10 cm and 3 cm moving out of a region of uniform magnetic field of 0.5T directed normal to the loop. If we want to move loop with a constant velocity 1cm/sec. Then required mechanical force is (Resistance of loop =1mΩ):-
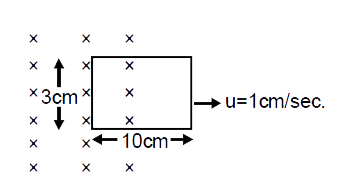 A2.25×10−3NB4.5×10−3NC9×10−3ND1.25×10−3N
A2.25×10−3NB4.5×10−3NC9×10−3ND1.25×10−3NA rectangular loop of sides 6 cm and 2 cm with a small cut is moving out of a region of uniform magnetic field of magnitude 0.4 T direction normal to the loop. The voltage developed across the cut if velocity of loop is 2cm−1 in a direction normal to the longer side is
A3.8×10−4VB4.8×10−4VC2.2×10−2VD3.2×10−2VA square loop of side 22cm is changed to a circle in time 0.4sec with its plane normal to a magnetic field 0.2T. The emf induced is
A+6.6mvB−6.6mvC+13.2mvD−13.2mv
A square loop of sides 10cm carries a current of 10A.A uniform magnetic field of magnitude 0.20T exists parallel to one of the side of the loop.(a)What is the force acting on the loop? (b)What is the torque acting on the loop?
View SolutionA rectangular loop of sides 20 cm and 10 cm carries a current of 5.0 A. A uniform magnetic field of magnitude 0.20 T exists parallel to the longer side of the loop (a) What is the force acting on the loop? (b) what is the torque acting on the loop?
View SolutionA rectangular loop of sides 8 cm and 2 cm with a small cut is moving out of a region of uniform megnetic field of magnitude 0.3 tesla directed normal to the loop. What is the voltage developed acorss the cut if velocity of the loop is 1cms−1 in a direction normal to the (i) longer side (ii) shorter side of the loop ? For how long does the induced voltage last in each case?
View SolutionA conducting square loop of side 10 cm is placed in a region of uniform magnetic field 0.5 T as shown in the figure. Calculate the work done in slowly moving the loop out of the field in 2 seconds with a speed of 0.05ms−1. The resistance of loop is 10 Ω.

View SolutionA rectangular loop of sides 20cm and 10cm carries a current of 5.0A. A uniform magnetic field of magnitude 2T exists parallel to the longer side of the loop. The torque acting on the loop is
View Solution
