In a quadrilateral ABCD, AC is the bisector of the (AB, AD) which is , , then is equal to
A
B
C
D
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for In a quadrilateral ABCD, AC is the bisector of the (AB, AD) which is (2pi)/(3), 15|AC|=3|AB|=5|AD|, then cos(BA, CD) is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PRODUCT OF VECTORSBook:ARIHANT MATHSChapter:PRODUCT OF VECTORSExercise:Exercise (More Than One Correct Option Type Questions)
Explore 36 Videos
- PRODUCT OF VECTORSBook:ARIHANT MATHSChapter:PRODUCT OF VECTORSExercise:Exercise (Statement I And Ii Type Questions)
Explore 12 Videos
- PRODUCT OF VECTORSBook:ARIHANT MATHSChapter:PRODUCT OF VECTORSExercise:Exercise For Session 4
Explore 10 Videos
- PROBABILITYBook:ARIHANT MATHSChapter:PROBABILITYExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 55 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES Book:ARIHANT MATHSChapter:PROPERTIES AND SOLUTION OF TRIANGLES Exercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 21 Videos
Similar Questions
Knowledge Check
In a quadrilateral ABCD, ¯¯¯¯¯¯AC⊥¯¯¯¯¯¯BD and AB=AD. ABCD is a ________
ATrapeziumBRhombusCRectangleDKiteIn a quadrilateral ABCD. −−→AC is the bisector of −−→AB and −−→AD, angle between −−→AB and −−→AD is 2π/3 . 15∣∣∣−−→AC∣∣∣=3∣∣∣−−→AB∣∣∣=5∣∣∣−−→AD∣∣∣. Then the angle between −−→BA and −−→CD is.
Acos−1(√14)7√2Bcos−1(√21)7√3Ccos−1(2)√7Dcos−1(2√7)14In a quadrilateral ABCD, ¯¯¯¯¯¯AC⊥¯¯¯¯¯¯BD and AB=AD. ABCD is a ________
AtrapeziumBrhombusCrectangleDKite
In cyclic quadrilateral ABCD, AD∣∣BC. Prove that AB = CD.
View SolutionIn Figure, if AD is the bisector of LA , show that: AB>BD (ii) AC>CD
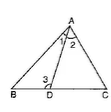
View SolutionShow that in a quadrilateral ABCD AB+BC+CD+DA>AC+BD
View SolutionIn Figure, AC>AB and AD is the bisector of LA . Show that ADC>∠ADB .
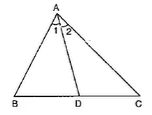
View SolutionIn quadrilateral ABCD, AB = AD and BC = CD. Show that ∠ABC=∠ADC.
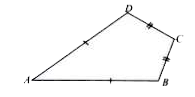
View Solution