An illuminant shaped as a plane horizontal disc S=100cm2 in area is suspended over the centre of a round table of radius R=1.0m. Its luminance does not depend on direction and is equal illuminant be suspended to provide maximum illuminance at the circumference of the table ? How great will that illuminance be? The liuminant is assumed to be a point source.
The equivalent luminous intensity in the direction OP is
LScosθ
and the illuminance at P is
LScosθ(R2+h2)cosθ=LSh2(R2+h2)2=LS(R2h+h)2=LS[(R√h−√h)2+2R]2

This is maximum when R=h
and the maximum illuminance is
LS4R2=1.6×1024=40 lux
Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Interference Of Light
33 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Diffraction Of Light
60 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
An illuminant shaped as a plane horizontal disc of raiud R=25cm is suspended over a table at a hight h=75cm. The illuminance of the table below the cnetre of the illuminant is equal to E0=70Ix. Assuming the source to obey Lambert's law, find its luminosity.
View SolutionAt what height should a lamp be hung above the centre of a round table of radius R to otain the maximum illumination at its edges?
View Solution
Knowledge Check
A lamp rated at 100 cd hangs over the middle of a round table with diameter 3 m at a height of 2 m . It is replaced by a lamp of 25 cd and the distance to the table is changed so that the illumination at the centre of the table remains as before. The illumination at edge of the table becomes X times the original. Then X is
A13B1627C14D19
A vertical shaft of light from a projector forms a light spot S=100cm2 in area on the celling of a round room of radius R=2.0m. The illuminance of the spot is equal to E=10001x. The reflection coeffiecient of the celling is equal to ρ=0.80. find the maximum illuminance of the well produced by the light reflected from the celling. the reflection is assumed to obey Lambert's law.
View SolutionA point source is suspended at a hight h=1.0m over the centre of a round table of radius R=1.0m. The luminous intensity I of the source depends on direction so that illuminance at all points of the table is the same. Find the function I(θ), where θ is the angle between the radiation direction and the vertical, as well as the luminous flux reaching the table if I(0)=I0=100cd.
View SolutionA point source is suspended at a height h over the centre of a round. Table The lumious intensity. P of the source depends on the direction θ with the normal function Pθ in terms of θ, assuming thatP(0)=P0
View SolutionA luminous done shaped as a hemisphere rests on a horizontal plane. Its luminosity is uniform. Determine the illuminance at the centre of that plane if its luminance equals L and is independent of direction.
View SolutionA student is studying a book placed near the edge of a circular table of radius R. A point source of light is suspended directly above the centre of the table. What should be the height of the source above the table so as to produce maximum illuminance at the position of the book ?
View SolutionA point source emitting uniformly in all directions is placed above a table top at a distance of 0.50 m from it. The luminous flux of the source is 1570 lumen. Find the illuminance at a small surface area of the table to a.directly below the source and b. at as distance of 0.80 m from the source.
View Solution(i) A spinning disk has a hole at its centre. The surface of the disk is horizontal and a small block A of mass m = 1 kg is placed on it.
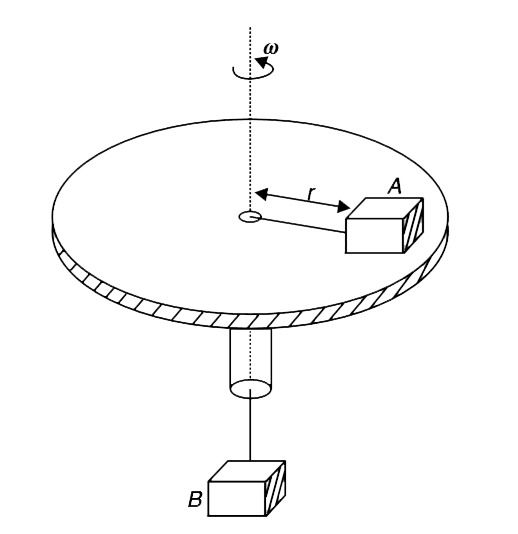
Block A is tied to a light inextensible string, other end of which passes through the hole and supports another block B of mass M = 2 kg. The coefficient of friction between A and the disk surface is 0.5. It was observed that the disk is spinning with block A remaining at rest relative to the disk. Block B was found to be stationary. It was estimated that length of horizontal segment of the string (r) was anywhere between 1.0 m to 1.5 m. With this data what estimate can be made about the angular speed (ω) of the disk. [g=10m/s2] (ii) A spring has force constant equal to k=100Nm–1. Ends of the spring are joined to give it a circular shape of radius R = 20 cm. Now the spring is rotated about its symmetry axis (perpendicular to its plane) such that the circumference of the circle increases by 1%. Find the angular speed (ω). Mass of one meter length of the spring is λ=0.126gm–1.
View Solution
