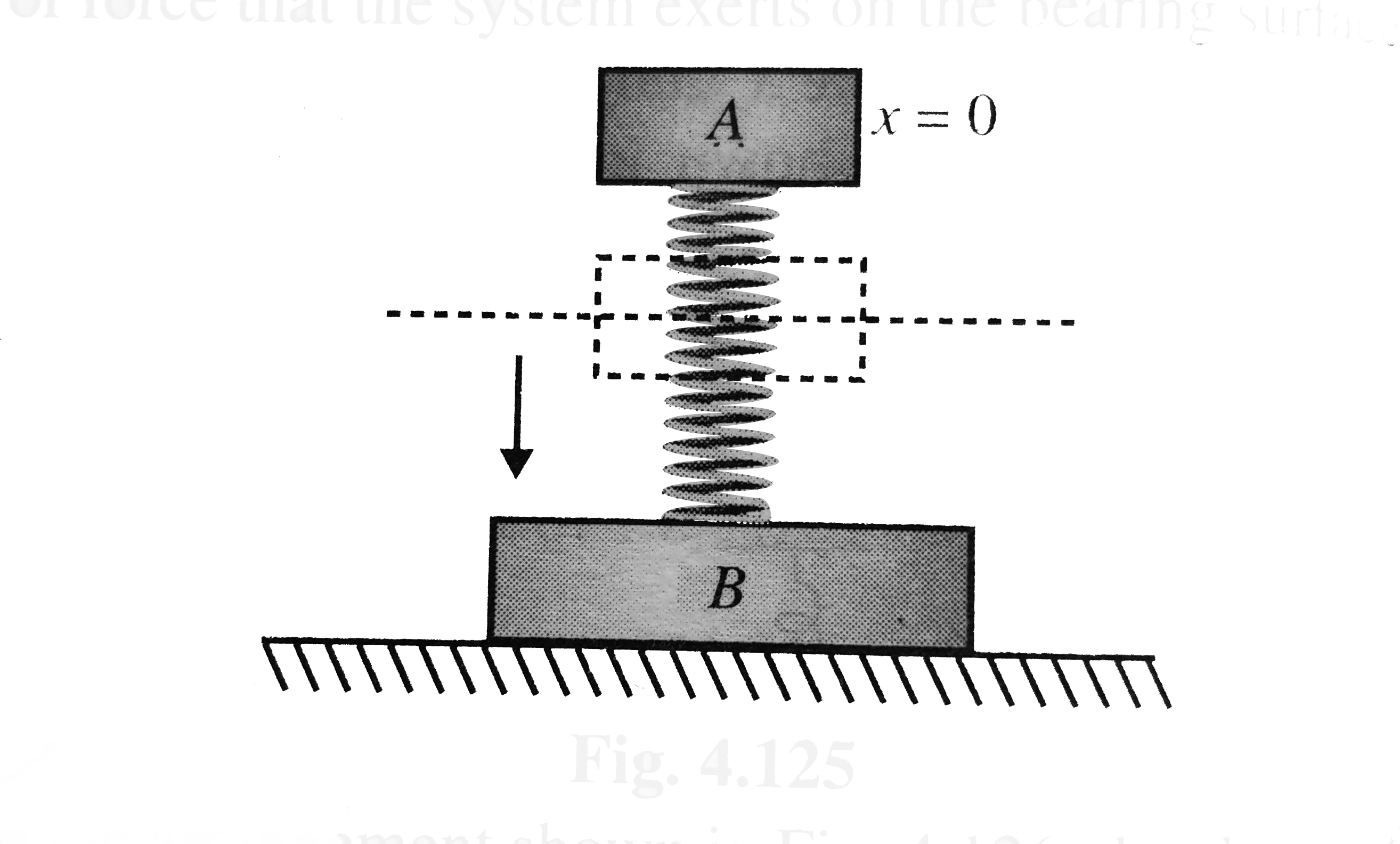
A body $A$ of mass $m_{1} = 1 k g$ and a body $B$ of mass $m_{2} = 4 k g$ are attached to the ends of a spring. The body $A$ performs vertical simple harmonic oscillations of amplitude $a = 1.6 c m$ and angular frequency $ω = 25 r a \frac{d}{s}$ . Neglecting the mass of the spring determine the maximum and minimum values of force the system exerts on the surface on which in rests.
$[Take g = 10 m / s^{2}]$

Video Solution

Text Solution

Verified by Experts

While the body $A$ is at its upper extreme position, the spring is obviously elongated by the amount
$(a - \frac{m_{1} g}{k})$
If we indicate $y -$ axis in vertically downward direction, Newton's second law of motion in projection form i.e. $F_{y} = m w_{y}$ for body $A$ gives :
$m_{1} g + k (a - \frac{m_{1} g}{k}) = m_{1} ω^{2} a$ or, $k (a - \frac{m_{1} g}{k}) = m_{1} (ω^{2} a - g) (1)$
(Because at any extreme position the magnitude of acceleration of an oscillating body equals $ω^{2} a$ and is restoring in nature.)
If $N$ be teh normal force exerted by the floor on the body $B$ , while the body $A$ is at its upper extreme position, from Newton's second lw for body $B$
$N + k (a - \frac{m_{1} g}{k}) = m_{2} g$
or, $N = m_{2} g - k (a - \frac{m_{1} g}{k}) = m_{2} g - m_{1} (ω^{2} a - g)$ ( using Eqn.1)
Hence $N = (m_{1} + m_{2}) g - m_{1} ω^{2} a$
When the body $A$ is at its lower extreme position, the spring is compressed by the distance $(a + \frac{m_{1} g}{k})$ .
From Newton's second law in projection from i.e. $F_{y} = m w_{y}$ for body $A$ at this state $:$
$m_{1} g = k (a + \frac{m_{1} g}{k}) = m_{1} (- ω^{2} a)$ or, $k (a + \frac{m_{1} g}{k}) = m_{1} (g + ω^{2} a) ... . . (3)$
In this case if $N^{'}$ be the normal force exerted by the floor on the body $B$ , From Newton's second law
for body $B$ we get : $N^{'} = k (a + \frac{m_{1} g}{k}) + m_{2} g = m_{1} (g + ω^{2} a) + m_{2} g$ (using En. 3)
Hence $N^{'} = (m_{1} + m_{2}) g + m_{1} ω^{2} a$
From Newton's third law the magnitude of sought forces are $N^{'}$ and $N$ , respectively.

Updated on:21/07/2023

A body $A$ of mass $m_{1}$ and body $B$ of mass $m_{2}$ are interconnected by a massless spring as shown. The body $A$ performs free vertical harmonic oscillations with the amplitude $A$ and frequency $f$ . The maximum value of $f$ such that body $B$ does not leave the surface is

A $\frac{1}{2 π} \sqrt{(\frac{m_{1} + m_{2}}{m_{2}}) \frac{g}{A}}$
B $\frac{1}{2 π} \sqrt{(\frac{m_{1} + m_{2}}{m_{1}}) \frac{g}{A}}$
C $\frac{1}{2 π} \sqrt{\frac{g}{A}}$
D $\frac{1}{2 π} \sqrt{\frac{m_{2}}{m_{1}} . \frac{g}{A}}$
A ball hung from a vertical spring oscillates in simple harmonic motion with an angular frequency of 2.6 rad/s and an amplitude of 0.075m. What is the maximum acceleration of the ball?
A $0.13 m / s^{2}$
B $0.20 m / s^{2}$
C $0.51 m / s^{2}$
D $2.6 m / s^{2}$
Two bodies of masses 1 kg and 4 kg are connected to a vertical spring, as shown in the figure. The smaller mass executes simple harmonic motion of angular frequency ${25 rad s}^{- 1}$ , and amplitude 1.6 cm while the bigger mass remains stationary on the ground. The maximum force exerted by the system on the floor is $(take g = 10 m s^{- 2})$ .

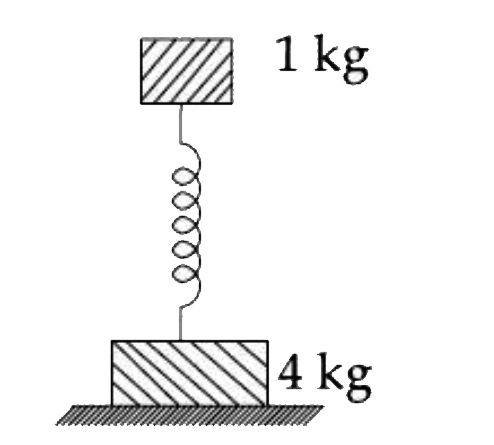A20 N
B60 N
C40 N
D10 N