A plane electromagentic wave E=Emcos(ωt−kr) whereEmEmey,k=kex,ey are the unit vectors of the x,y axes, propagates in vacuum. Find the vector H at the point with radius vector r=xex at the moment (a)t=0,(b)t=t0. Consider the case when Em=160V/m,k=0.51m−1,x=7.7m, and t0=33ns.
As in the previous problem
→H=→k×→Emμ0cos(ωt−→k.→r)=Emμ0cˆexcos(kx−ωt)
=√ε0μ0Emˆexcos(kx−ωt)
Thus
(a) at t=0,→H=√ε0μ0Emˆexcoskx
(b) at t=t0,→H=√ε0μ0Emˆexcos(kx−ωt0)
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
A plane electromagentic wave E=Emcos(ωt−kr) propagates in vacuum. Assuming the vectors Em and k to be known, find the vector H as a function of time t at the point with radius vector r=0 .
View SolutionA standing electromagnetic wave E=Emcoskx.Cosωt is sustained along the x axis in vacuum. Find the projection of the Poyntind vector on the x axis Sx(x,t) and the mean value of that projection averaged over an oscillation period.
View Solution
Knowledge Check
A plane electromagnetic wave Ez=100cos(6×108t+4x) V/m propagating in medium of dielectric constant is
A1.5B2C2.4D4Equations of the tangent and normal to the curve x=etsint,y=etcost at the point t=0 on it are respectively
Ay=x+1,x+y=1Bx+y+1=0,x−y=1Cx−y=1,x+y=2Dx−y=1,x+y+2=0The following travelling electromagnetic wave Ex=0,Ey=E0sin(kx+ωt),Ez=–2E0sin(kx+ωt) is-
Aelliptically polarizedBcircularly polarizedClinearly polarizedDunpolarized
A plane electromagnetic wave E=Emcos(ωt−kx) propagating in vacuum induces the emf Eind, in a square frame with side l. The orientation of the frame is shown in figure. Find the amplitude value εind , if Em=0.50mV/m, the frquency v=5.0MHz and l=50cm.
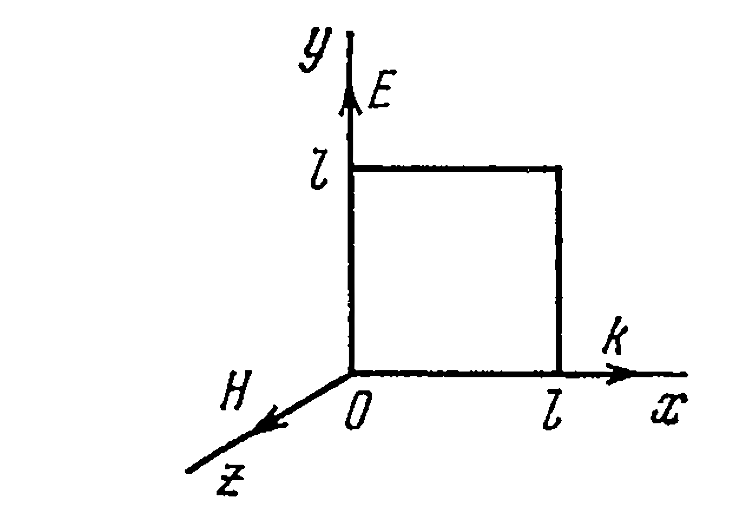
View SolutionFind the mean Plynting vector (:S:) of a plane electromagnetice wave E=Emcos(ωt−kr) if the wave propagates in vacuum.
View SolutionA standing electromagnetic wave with electric component E=Emcoskx.Cosωt is sustained along the x axis in vacuum. Find the magnetic component of the wave B(x,t). Draw the approximate distribution pattern of the wave's electric and magnetic components (E and B) at the moments t=0 and t=T/4, where T is the oscillation period.
View SolutionWhat kind of polarizations has a plane electromagetic wave if the projections of the vector E on the x and y axes are perpendicular to the propagation direction and are defind by the following equations:
(a) Ex=Ecos(ωt−kz),Ey=Esin(ωt−kz),
(b) Ex=Ecos(ωt−kz),Ey=Ecos(ωt−kz+π/4)
(c) Ex=Ecos(ωt−kz),Ey=Ecos(ωt−kz+π)?
View SolutionA plane wave of frquency ω propagates so that a certain phase of oscillation moves along the x,y,z axes with velocities υ1,υ2,υ3. Respectively. Find the wave vectork, assuming the unit vetors ex,ey,ez of the coordinate axes to be assigned.
View Solution
