A long straight solenoid has n turns per unit length. An alternating current I=Imsinωt flows throught it. Find the displacement current density as a function of the distnace r from the solenoid equals R.
Inside the solenoid, there is a magnetic field,
B=μ0nImsinωt.
since this varies in time there is an associated electric field. This is obtained by using,
∮C→E.d→r=−ddt∫S→B.D→S
For r<R,2πrE=−.B.πr2, or, E=−.Br2
Forr>R,E==−.BR22r
The associated displacement current density is
j4=ε0∂E∂t=[−ε0..Br/2−ε0..BR2/2r
The answer given in the book, is dimensionally incorrect without the factor ε0
Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field
36 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics
69 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
In a long staright solenoid with cross-sectional radius a and number of turns per unit length n a current varies with a constant velocity.IA/s. Find the magntidue of the eddy current field strength as a function of the distance r from the solenoid axis. Draw the appoximate plot of this function.
View SolutionConsider a long solenoid of radius R which has n turns per unit length. A time dependent current I=I0sinωt flows solenoid, magnitude of electric field at a perpendicular distance r<R, from the axis of symmetry of solenoid is found to be E=α4ωμ0nI0rcosωt, where α is pure number and μ0 is permability of free space. Find α
View Solution
Knowledge Check
The magnetic field B within the solenoid having n turns per unit length and carrying a current I is given by
Aμ0nIB4πμ0nICnIDμ0nIlA long solenoid has n turns per metre and current IA is flowing through it. The magnetic field induciton at the ends of the solenoid is
AμonI2BμonICZeroD2μonIA long solenoid has 800 turns per metre length of solenoid. A current of 1.6 A flows through it. The magnetic induction at the end of the solenoid on its axis is
A16×10−4TB8×10−4TC32×10−4TD4×10−4T
A very long straight solenoid has a cross section radis R and n turns per unit lenghth. A direct current Iflows throguh the solenoid. Suppose that x is the distance from the end of the the solenoid, measured along its axis. Find:
(a) the magnetic induction B on the axis as a funciton of x, draw an approximate plot of B vs ratio x/R,
(b) the distance x0 to the point on the axis at which the value of B differs by η=1% from that in the middle section of the solnoid.
View SolutionA long solenoid has n turns per unit length and carries a current i=i0sinωt. A coil of N turns and area A is mounted inside the solenoid and is free to rotate about its diameter that is perpendicular to the axis of the solenoid. The coil rotates with angular speed ω and at time t = 0 the axis of the coil coincides with the axis of the solenoid as shown in figure. Write emf induced in the coil as function of time.
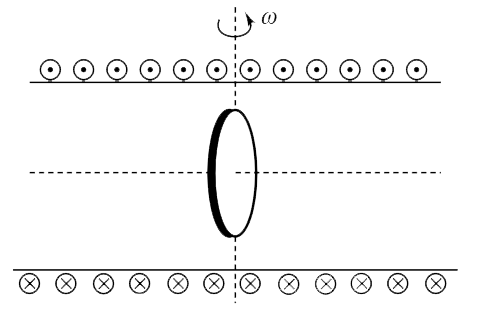
View SolutionA long solenoid has 800 turns per metre length of solenoid A current of 1.6 A flow through it. The magnetic induction at the end of the solenoid on its axis is
View SolutionThe magnetic field on the axis of a long solenoid having n turns per unit length and carrying a current is
View SolutionConsider a long solenoid of n terms per unit length and carrying a current J. The magnetic field in the interior of the solenoid is given by
View Solution
