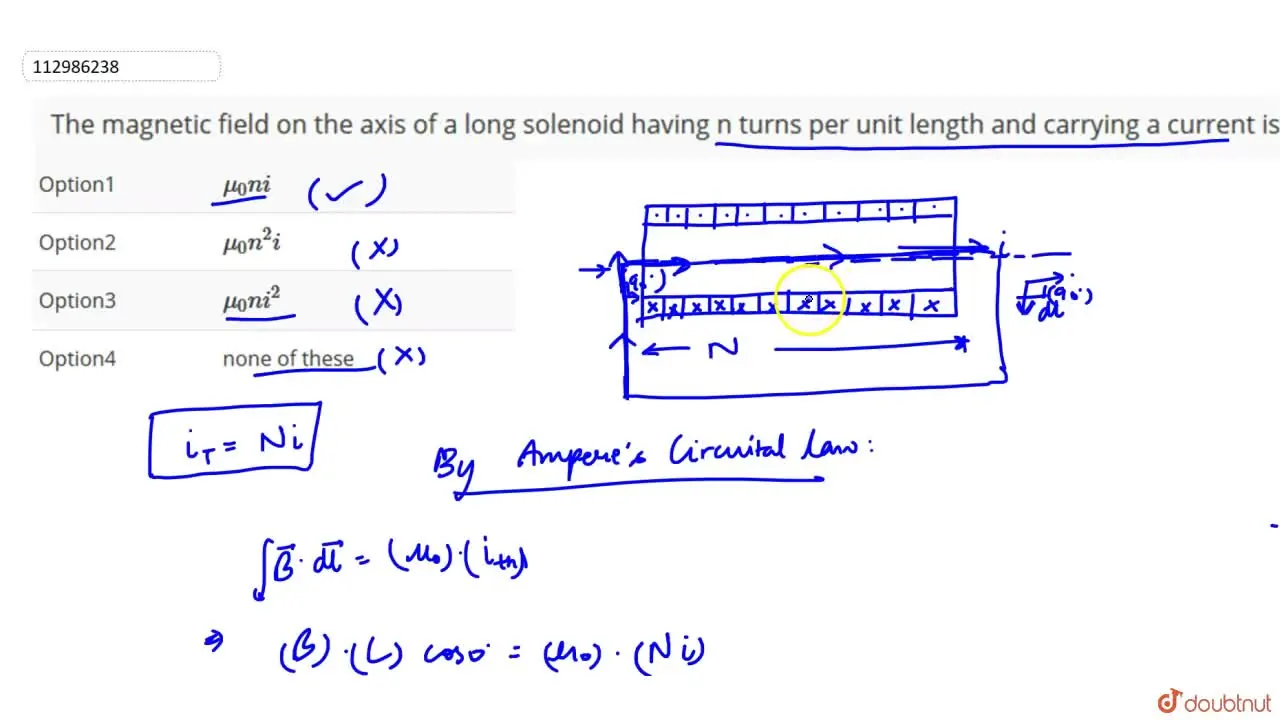
The magnetic field on the axis of a long solenoid having n turns per unit length and carrying a current is
μ0ni
μ0n2i
μ0ni2
none of these
The correct Answer is:A
If the solenoid is of infinite length and the point is well inside the solenoid Bin=μ0ni
Related Playlists
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENTEXERCISE - MHT CET CORNER20videos
MAGNETIC EFFECT OF ELECTRIC CURRENT
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENTEXERCISE - Exercise 136videos
MAGNETIC EFFECT OF ELECTRIC CURRENT
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - KINETIC THEORY OF GASES ANDRADIATIONEXERCISE - MHT CET Corner40videos
KINETIC THEORY OF GASES ANDRADIATION
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENT -IEXERCISE - EXERCISE 2 (MISCELLANEOUS PROBLEMS)20videos
MAGNETIC EFFECT OF ELECTRIC CURRENT -I
Similar Questions
Find the magnetic intensity H at the centre of a long solenoid having n turns per unit length and carrying a current i (a) when no material is kept in it and (b) when a long copper rod is inserted in the solenoid.
View SolutionThe magnetic field (B) inside a long solenoid having n turns per unit length and carrying current / when iron core is kept in it is (μo = permeability of vacuum, χ = magnetic susceptibility)
View SolutionThe magnetic field B within the solenoid having n turns per unit length and carrying a current I is given by
View SolutionThe magnetic field B with in the solenoid having n turns per metre length and carrying a current of i ampere is given by
View SolutionThe magnetic field at a point on the axis of a long solenoid having 5 turns per cm length when a current of 0.8 A flows through it is
View SolutionChoose the correct option:
The magnetic field inside a long straight solenoid carrying current:View SolutionThe magnetic field inside a long straight solenoid carrying current
View Solution(a) State Ampere's cicuital law. Use this law to obtain the expression for the magnetic field inside an air cored toriod of average radius 'R',having 'n' truns per unit length and carrying a steady current I.
(b) An observer to the left of a solenoid of N turns each of cross section area 'A' observes that a steady current I in it flows in the clockwise direction. Depict the magnetic field lines due to the solenoid specifying its polarity and show that it acts as a bar magnet of magnetic moment m=NIA.View SolutionState Ampere's circuital law. Use this law to obtain the expression for the magnetic field inside an air cored toroid of average radius r having n-turns per unit length and carrying a steady current I.
View SolutionThe magnetic field inside a long straight solenoid-carrying current.
View Solution