A cylindrical layer of dielectric with permittivity ε is inserted into a cylindrical capacitor to fill up all the space between the electrodes. The mean radius of the electrodes equals R, the gap between them is equal to d, with d<<R. The constant voltage V is applied across the electrodes of the capacitor. Find the magnitude of the electric force pulling the dielectric into the capacitor.
We known that energy of a capacitor , U=q22C
Hence, from Fx=∂U∂x∣∣∣q=const, We have, Fx=q22∂c∂x/C2 ....(1)
Now, since d<<R, the capacitance of the given capacitor can be calculated by the formula of a parallel plate capacitor. Therefore, if the dielectric is intoduced upto a depth x and the length of the capacitor is l, we have,
C=2πε0εRxd+2πRε0(l−x)d ....(2)
From (1) and (2) we get,
Fx=ε0(ε−1)πRV2d
Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Current
72 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics
69 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Conductors And Dielectrics In An Electric Field
47 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
A dielectric slab is inserted between the plates of a capacitor. The charge on the capacitor is Q and the magnitude of the induced charge on each surface of the dielectric is Q'.
View SolutionUnder standard conditions helium fills up the space between two long coaxial cylinders. The mean radius of the cylinders is equal to R, the gap between them is equal to ΔR, with ΔR<<R. The outer cylinder rotates with a fairly low angular velocity ω about the stationary inner cylinder. Down to what magnitude should the helium pressure be lowered (keeping the temperature constant) to decrease the sought moment of friction forces n=10 times if ΔR=6mm ?
View Solution
Knowledge Check
Voltage applied between the electrodes fo discharge tube is of the nature
A220 V alternating voltageB220 V DC voltageCvariable voltageDvltage obtained by induction coilA parallel plate capacitor was lowered into water in a horizontal position, with water filling up the gap between the plates. The distance between the plates is d. Then a constant voltage V was applied to the capacitor. Find the water pressure increment in the gap. Dielectric constant of water is k.
AΔp=ε0(k−1)V22d2BΔp=ε0k(k−1)V22d2CΔp=ε0(k−1)V24d2DΔp=2ε0(k−1)V2d2When dielectric is inserted in the space between plates of a capacitor, then
Amagnitude of charge increases.Bmagnitude of charge decreases.Ccharge remains the same.Dcharge becomes zero.
A capacitor consists of two stationary plates shaped as a semi-circle of radius R adn a movable plate made of dielectric with permittivity ε and capacble of rotoning about an axis O between the stationary plates (fig). The thickness of the movables plate is equal to d which is practically the separation between teh stationary plates. A potential difference V is applied to the capacitor. FInd the magnitude of the moment of forces relative to the axis O acting on teh movable plate in the position shown in the figure.

View SolutionA parallel-plate capacitor was lowered into water in a horizontal position, with water filling up the gap between the plates d=1.0mm wide. Then a constant voltage V=500V was applied to the capacitor. Find the water pressure increment in the gap.
View SolutionA capacitor consists of two stationary plates shaped as a semi-circle of radius R and a movable plate made of dielectric with permittivity K and capable ofrotating about an axis O between the stationary plates. The thickness of movable plate is equal to d which is practically the separation between the stationary plates. A potential difference V is applied to the capacitor. Find the magnitude of the moment of forces relative to the axis O acting on the movable plate in the position shown in figure.
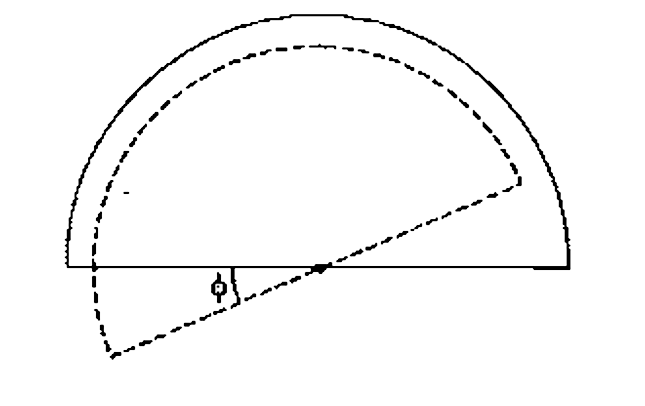
View SolutionThe gap between the plates of a parallel-plate capacitor is filled with istropic dielectrc whose permittlvity ε varies linearly from esπlon1 to ε2(ε2>ε1) in the direction perpendicular to the plates. The area of each plate equals S, the separation between the plates is equal to d. Find :
(a) the capacitance of the capacitor,
(b) the space density of the bound chagres as a function of a if the charge of the capacitor is q and E in it is directed toward the growing ε values.
View SolutionConsider a disconnected. Plate capacitor of capacity 10μ with air filled in the gap between the plates. Now one-half of the space between the plates is filled with a dielectric of dielectric constant 4 as shown in .The capacity of the capacitor changes to
 .
.
View Solution
