A spherical shell is uniformly charged with the surface density $σ$ . Using the energy conservation law, find the magnitude of the electric force acting on a unit area of the shell.

Video Solution

Text Solution

Verified by Experts

Energy of the chagred sphere of radius $r$ , from the equation
$U = \frac{1}{2} q φ = \frac{1}{2} q \frac{q}{4 π ε_{0} r} = \frac{q^{2}}{8 π ε_{0} r}$
If the radius of the shell changes by $d r$ then work done is
$4 π r^{2} F_{u} d r = - d U = q^{2} / 8 π ε_{0} r^{2}$
Thus sought force per unit area,
$F_{u} = \frac{q^{2}}{4 π r^{2} (8 π ε_{0} r^{2})} = \frac{{(4 π r^{2} σ)}^{2}}{4 π r^{2} \times 8 π ε_{0} r^{2}} = \frac{σ^{2}}{2 ε_{0}}$

Updated on:21/07/2023

A charge $q$ is placed at the some distance along the axis of a uniformly charged disc of surface charge density $σ$ . The flux due to the charge $q$ through the disc is $ϕ$ . The electric force on charge $q$ exerted by the disc is
A $σ ϕ$
B $\frac{σ ϕ}{4 π}$
C $\frac{σ ϕ}{2 π}$
D $\frac{σ ϕ}{3 π}$
A spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
Ainside is zero and varies as $x^{- 1}$ outside it
Binside is non zero constant and varies as $x^{2}$ outside it
Cinside is zero and varies as $x^{- 2}$ outside it
Dinside is constant and varies as $x^{- 1}$ outside it
A small section of area $Δ A$ is removed from a uniform spherical shell with surface mass density $σ$ and radius R as shown in the figure . Find the magnitude of gravitational field intensity at point P due to the remaining mass .

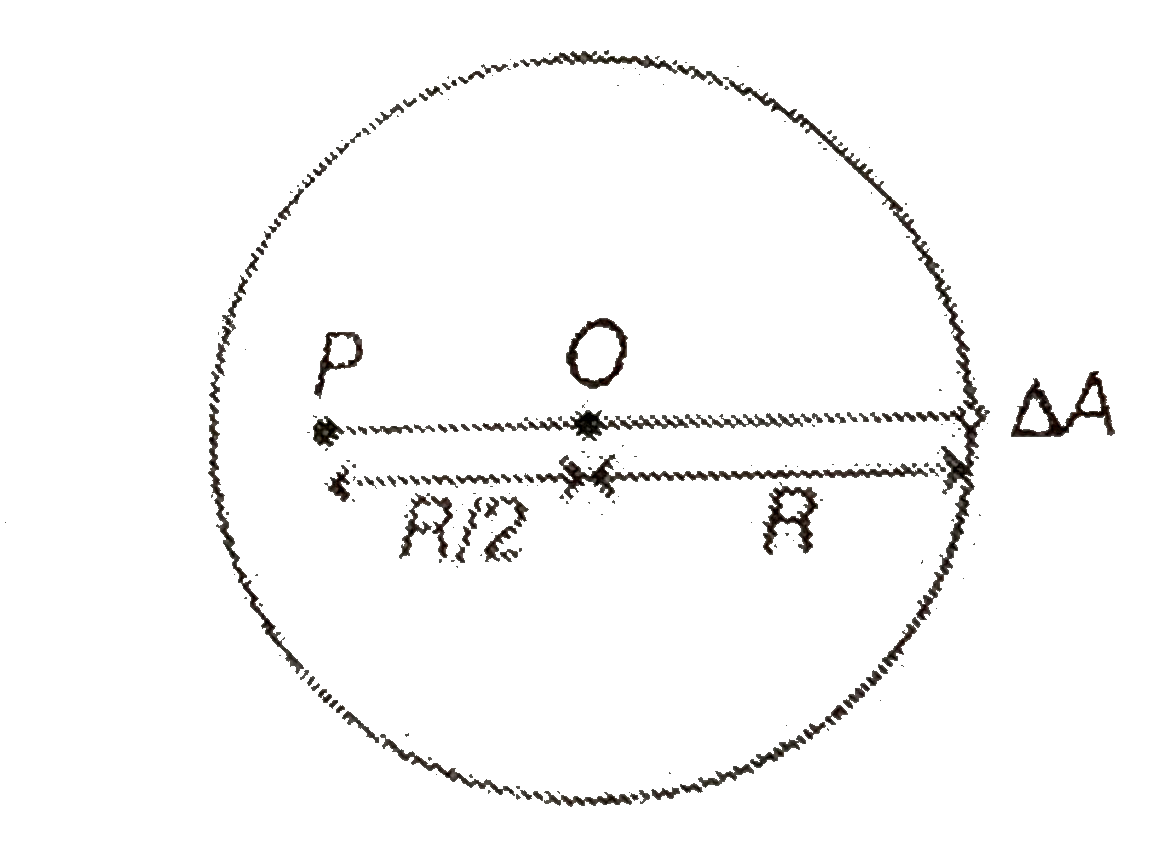A $\frac{4 σ Δ A G}{9 R^{2}}$
B $\frac{4 σ Δ A G}{R^{2}}$
C $\frac{σ Δ A G}{R^{2}}$
Dzero