A very long straight uniformly charged thread carries a charge λ per unit length. Find the magnitude and direaction of the electric field strength at point which is at a distance y from the thread and lies on the perpendicular passing through one of the thread's ends.
The problem is reduced to finding Ex and Ey viz, the projections of →E in fig. where is assumed that λ>0.
Let us start with Ex. The contribution to E from the charge element of the segment dx is
dEx=14πε0λdxr2sinα (1)
Let us expression to the form convenlent for intergation. In our case, dx=rdα/cosα,r=y/cosα. Then
dEx=λ4πε0ysinαdα
Intergating this expressions over α between 0 and π/2, we find
Ex=λ/4πε0y.
In order to find the projection Ey is is sufficient to recall that dEy differs from dEx in that sin α in (1) in simply replaced by cos aplha.
This gives
dEy=(λcosαdα)/4πε0y and Ey=λ/4πε0y.
We have obtained an intersting result :
Ex=Ey independentaly of y.
i.e.,→E is oriented at the angle of 45∘ to the rod. The modulus of →E is
E=√E2s+E2y=λ√2/4πε0y.

Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Conductors And Dielectrics In An Electric Field
47 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Capacitance - Energy Of An Electric Field
46 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
find the electric field strength due to a point charge of 5μC at a distance of 80 cm from the charge.
View SolutionFigure 22-24 shows a plastic rod having a uniformly distributed charge. The charge per unit length of the rod is λ. Find the electric field due to the rod at a point P which is at a distance r from the rod.
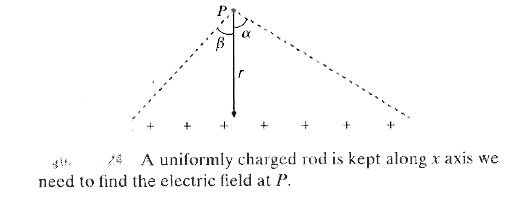
View Solution
Knowledge Check
A 10 cm long rod carries a charge of +150μC distributed uniformly along its length. Find the magnitude of the electric field at a point 10 cm from both the ends of the rod.
A15.6×107N/CB15.6×105N/CC1.56×107N/CD1.56×105N/CA long strikes with a charge of λ per unit length passes through an imaginary cube of edge a. The maximum flux of the electric field through the cube will be
Aλaε0B√λaε0C6λa2ε0D√3λaε0A ring of radius a contains a charge q distributed uniformly over its length. Find the electric field at a point on the axis of the ring at a distance x from the centre.
Akq2x(a2+x2)32B2kq2x(a2+x2)32Ckq2x(a2+x2)32Dkq22x(a2+x2)32
A thin straight rod of length 2a carrying a uniformly distributed charge q is located in vacumm. Find the magnitude of the electric field strength as a function of the distatance r from the rod's centre along the streaight line
(a) perpendicular to the rod and passing through its centre ,
(b) coinciding with the rod's direction (at the point lying outside the rod).
View SolutionA rod of length 20 cm carries a charge of +150 μC that is uniformly distributed along its length. Find the electric field intensity at a point, which is 20 cm away from both the ends of the rod.
View SolutionA charge q is uniformly distributed along an insulating straight wire of length 21 as shown in Fig. Find an expression for the electric potential at a point located a distance d from the distribution along its perpendicular bisector.
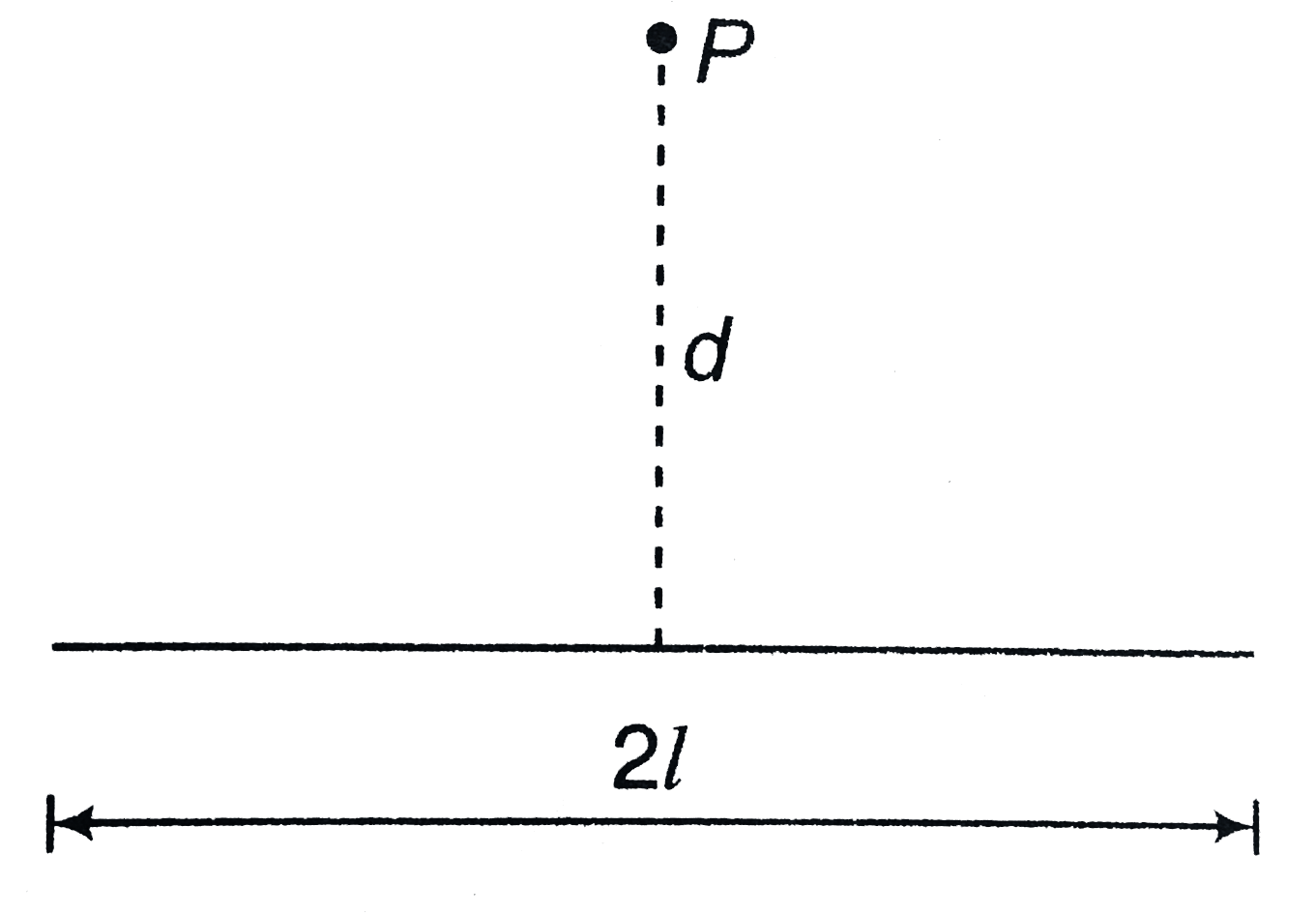
View SolutionEach of the two long parallel threads carries a uniform charge λ per unit length. The threads are separated by a distance l. Find the maximum magnitude of the electric firld strength in the symmetry plane of theis system located between the threads.
View SolutionA very long uniformly charged thin wire whose one end is at point (0,a) extends along positive y-axis . The charge per unit length of wire is λ . The electric field at origin is
View Solution
