A thin nonconducting ring of radius R has a linear charge density λ=λ0cosφ, where λ0 is a constant , ϕ is the azimutahl angle. Find the magnitude of the electric field strength
(a) at the centre of the ring ,
(b) on the axis of the ring as a function of the distance x from its centre. Investegation the obtained function at x>>R.
To solve the problem, we will break it down into two parts:
Part (a): Electric Field at the Center of the Ring
1. Understanding the Charge Distribution: The linear charge density is given by λ(φ)=λ0cosφ. This means that the charge is distributed non-uniformly along the ring.
2. Consider a Small Element of Charge: Take a small element of the ring dφ at an angle φ. The charge dq on this element is:
dq=λ(φ)Rdφ=λ0Rcosφdφ
3. Electric Field Contribution: The electric field dE due to this charge at the center of the ring will have both x and y components. However, due to symmetry, the y-components will cancel out when integrating over the entire ring.
4. Calculating the x-component: The x-component of the electric field due to dq at the center is:
dEx=dEcosφ
where dE=kdqR2=kλ0RcosφdφR2=kλ0cosφRdφ.
5. Integrating Over the Ring: The total electric field at the center is:
Ex=∫2π0dEx=∫2π0kλ0cosφRdφ
The integral of cosφ over a complete cycle (0 to 2π) is zero:
Ex=0
6. Conclusion for Part (a): Therefore, the electric field at the center of the ring is:
E=0
Part (b): Electric Field on the Axis of the Ring
1. Setting Up the Geometry: Consider a point on the axis of the ring at a distance x from the center. The electric field due to a small charge element dq at this point will have a component along the axis.
2. Electric Field Contribution: The distance from the charge element to the point on the axis is given by:
r=√R2+x2
The electric field due to dq at this point is:
dE=kdqr2=kλ0RcosφdφR2+x2
3. Finding the z-component: The z-component of this electric field is:
dEz=dE⋅xr=kλ0RcosφdφR2+x2⋅x√R2+x2
4. Integrating Over the Ring: The total electric field along the z-axis is:
Ez=∫2π0dEz=∫2π0kλ0Rcosφx(R2+x2)3/2dφ
Again, the integral of cosφ over a complete cycle is zero:
Ez=0
5. Conclusion for Part (b): Therefore, the electric field on the axis of the ring is also:
E=0
Investigating the Function at x≫R
1. Behavior of the Electric Field: Since both components of the electric field are zero regardless of the distance x, the electric field remains zero even as x becomes much larger than R.
Summary of Results
- (a) The electric field at the center of the ring is E=0.
- (b) The electric field on the axis of the ring is also E=0, and this holds true for x≫R.
dq=λ(φ)Rdφ=λ0Rcosφdφ
dEx=dEcosφ
where dE=kdqR2=kλ0RcosφdφR2=kλ0cosφRdφ.
Ex=∫2π0dEx=∫2π0kλ0cosφRdφ
The integral of cosφ over a complete cycle (0 to 2π) is zero:
Ex=0
E=0
r=√R2+x2
The electric field due to dq at this point is:
dE=kdqr2=kλ0RcosφdφR2+x2
dEz=dE⋅xr=kλ0RcosφdφR2+x2⋅x√R2+x2
Ez=∫2π0dEz=∫2π0kλ0Rcosφx(R2+x2)3/2dφ
Again, the integral of cosφ over a complete cycle is zero:
Ez=0
E=0
- (a) The electric field at the center of the ring is E=0.
- (b) The electric field on the axis of the ring is also E=0, and this holds true for x≫R.
Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Conductors And Dielectrics In An Electric Field
47 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Capacitance - Energy Of An Electric Field
46 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
A thin nonconducting ring of radius r has a linear charge density q=q0cosθ, where q0 is a constant and θ is the angle at the centre from the diameter of maximum charge density in the anticlockwise direction. Find the electric field at the centre of the ring.
View SolutionA point charge q is located at the centre fo a thin ring of radius R with uniformly distributed charge −q, find the magnitude of the electric field strength vectro at the point lying on the axis of the ring at a distance x from its centre, if x>>R.
View Solution
Knowledge Check
A thin non-conducting ring of radius R has a linear charge density λ=λ0cosθ, where θ is measured as shown. The total electric dipole moment of the charge distribution is
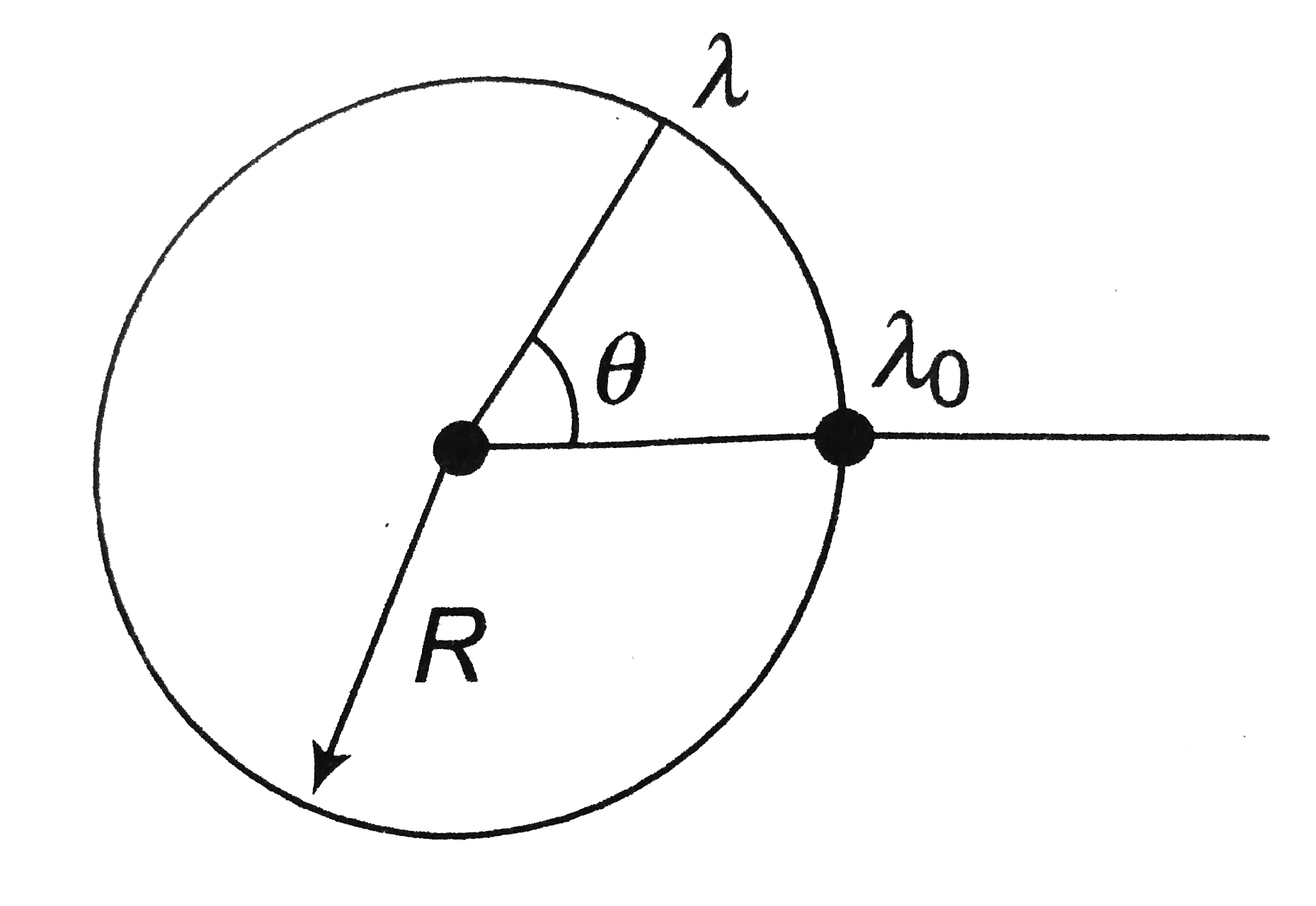 AR2λ0B2πR2λ0CπR2λ02DπR2λ0
AR2λ0B2πR2λ0CπR2λ02DπR2λ0A half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
Aλ4εoln0Bλ4π2ε0rCλ4πε0rDλ4πε0r2The linear charge density on a ring of radius R is λ=λ0sin(θ) where λ0 is a constant and θ is angle of radius vector of any point on the ring with x-axis. The electric potential at centre of ring is
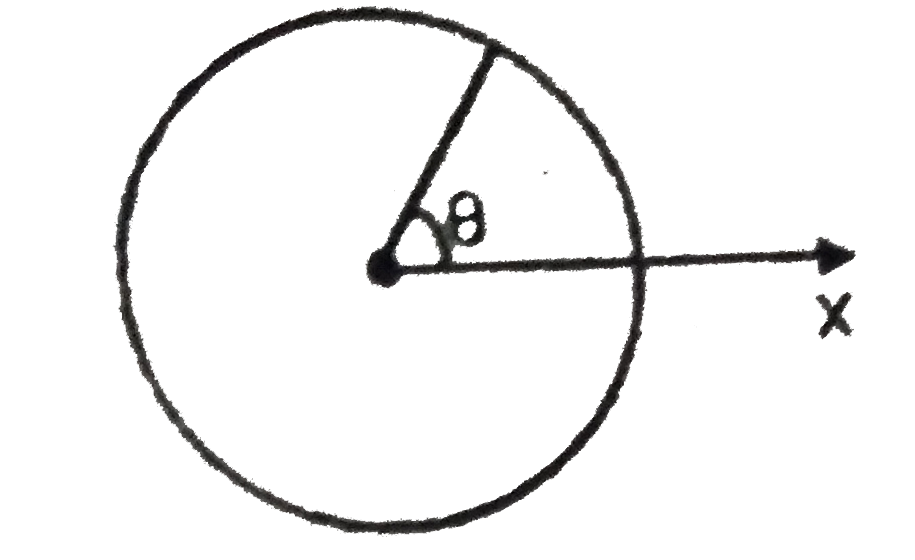 A0Bλ0∈0Cλ0π∈0Dλ02π∈0
A0Bλ0∈0Cλ0π∈0Dλ02π∈0
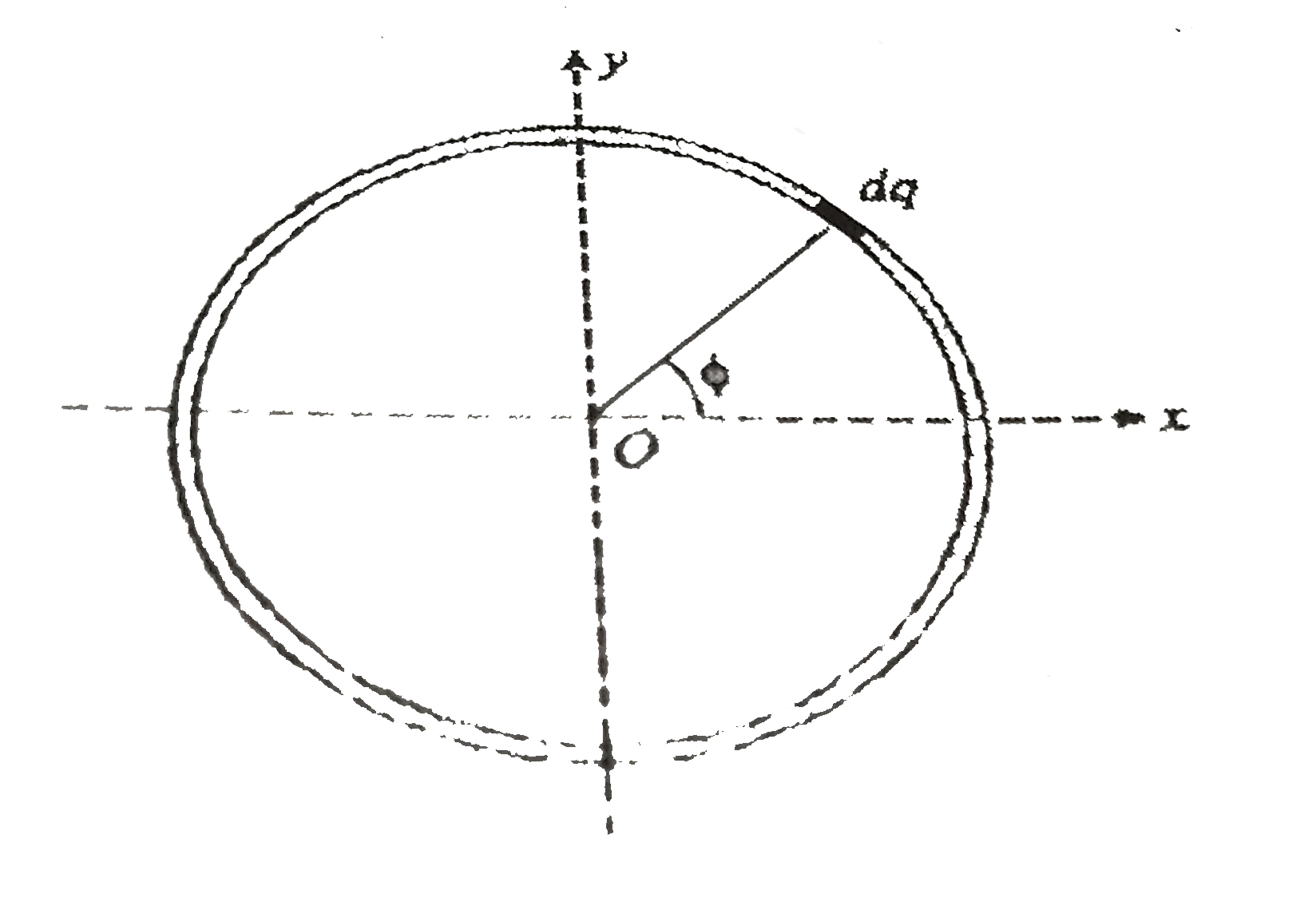
A thin non-conducting ring or radius a has a linear charge density λ=λ0sinϕ. A uniform electric field E0ˆi+E0ˆj exist in the region . .Net torque acting on ring is given as :
View SolutionA thin half- ring of radiusb 20 cm is uniformly charged with a total charge of 0.7nC. Find the magnitude of the electric field at the centre of the centre of the half-ring.
View SolutionA ring has radius 30cm and a charge of 2nC .The electric potential on the axis of the ring at a distance of 40cm from its centre will be
View SolutionA quarter ring of radius R is having uniform charge density λ. Find the electric field and potential at the centre of the ring.
View SolutionThe linear charge density on a dielectric ring of radius R vanes with θ as λ=λ0cosθ/2, where λ0 is constant. Find the potential at the center O of the ring [in volt].
View Solution
