A certain system of particles possesses a total momentum p and an angular momentum M relative to a point O. Find its angular momentum M′ relative to a point O′ whose position with respect to the point O is determined by the radius vector r0. Find out when the angular momentum of the system of particles does not depend on the choice of the point O.
Let position vectors of the particles of the system be →ri and →'ri with respect to the points O and O′ respectively. Then we have,
→ri=→'ri+→r0 (1)
where →r0 is the radius vector of O′ with respect to O.
Now, the angular momentum of the system relative to the point O can be written as follows:
→M=∑(→ri×→pi)=∑(→'ri×→pi)+∑(→r0×pi) [using (1)]
or, →M=→'M+(→r0×→p), where, →p=∑→pi
From (2), if the total linear momentum of the system, →p=0, then its angular momentum does not depend on the choice of the point O.
Note that in the C.M. frame, the system of particles, as a whole is at rest.
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation
34 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Dynamics Of A Solid Body
56 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - The Fundamental Equation Of Dynamics
59 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
Knowledge Check
Angular momentum of the system of particles changes when
Aforce acts on a bodyBtorque acts on a bodyCdirection of velocity changesDnone of theseA particle is moving in a horizontal uniform circular motion. The angular momentum of the particle is conserved about the point :
ACentre of the circleBOutside the circleCInside the circleDPoint on circumferenceA particle has a linear momentum p and position vector r. the angular momentum of this particle about the origin will not be zero under the conditions
Ap=0Bp is perpendicular to r and does not cross rCp is anti-parallel to rDthe particle passes through the origin.
The z component of the angular momentum of a particle whose position vector is
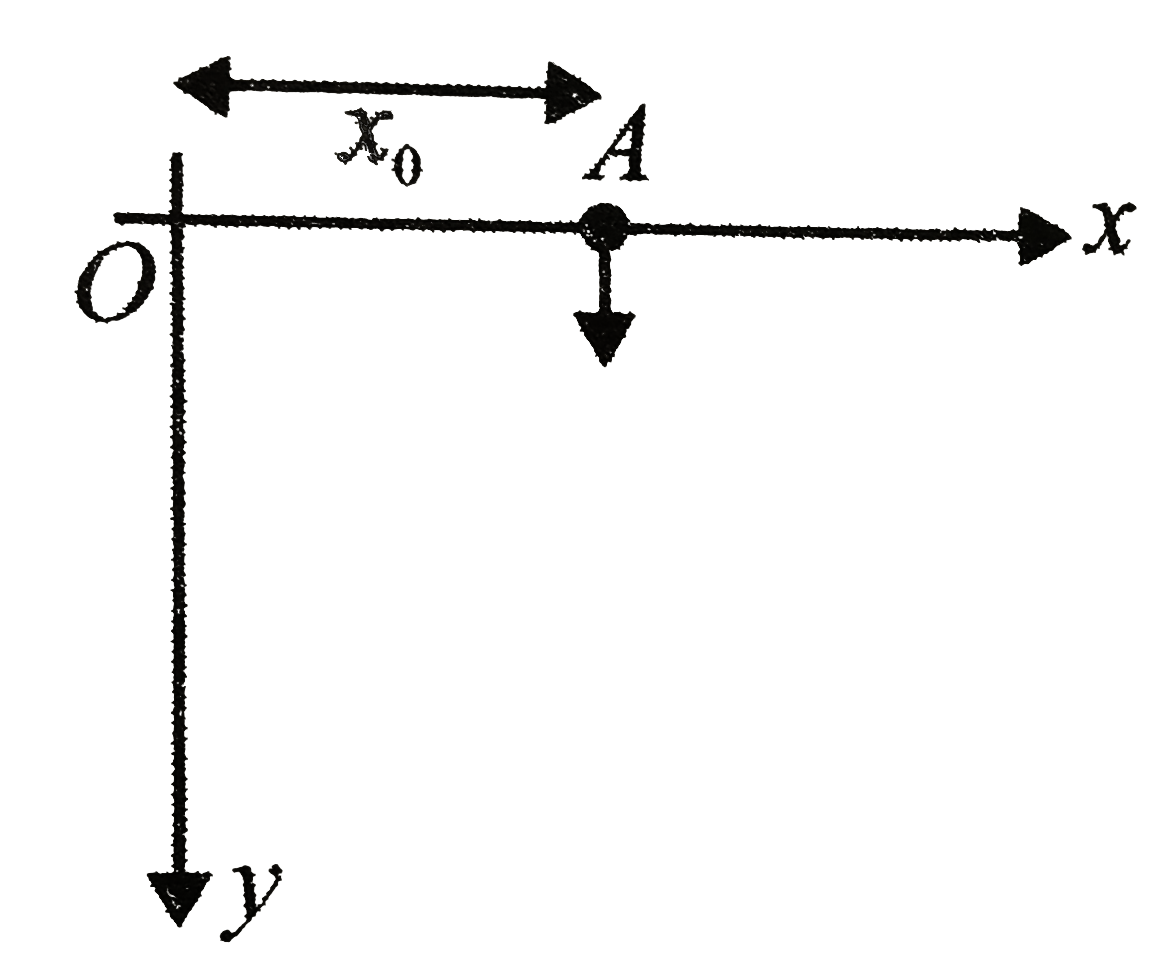
View SolutionA particle of mass m is released from rest at point A in Fig., falling parallel to the (vertical) y-axis. Find the angular momentum of the particle at any time t with respect to the same origin O.
View SolutionA particle of mass m is projected with velocity v at an angle θ with the horizontal. Find its angular momentum about the point of projection when it is at the highest point of its trajectory.
View SolutionA particle of mass m is projected with a speed u at an angle θ with the horizontal. Find angular momentum of particle after time t about point of projection.
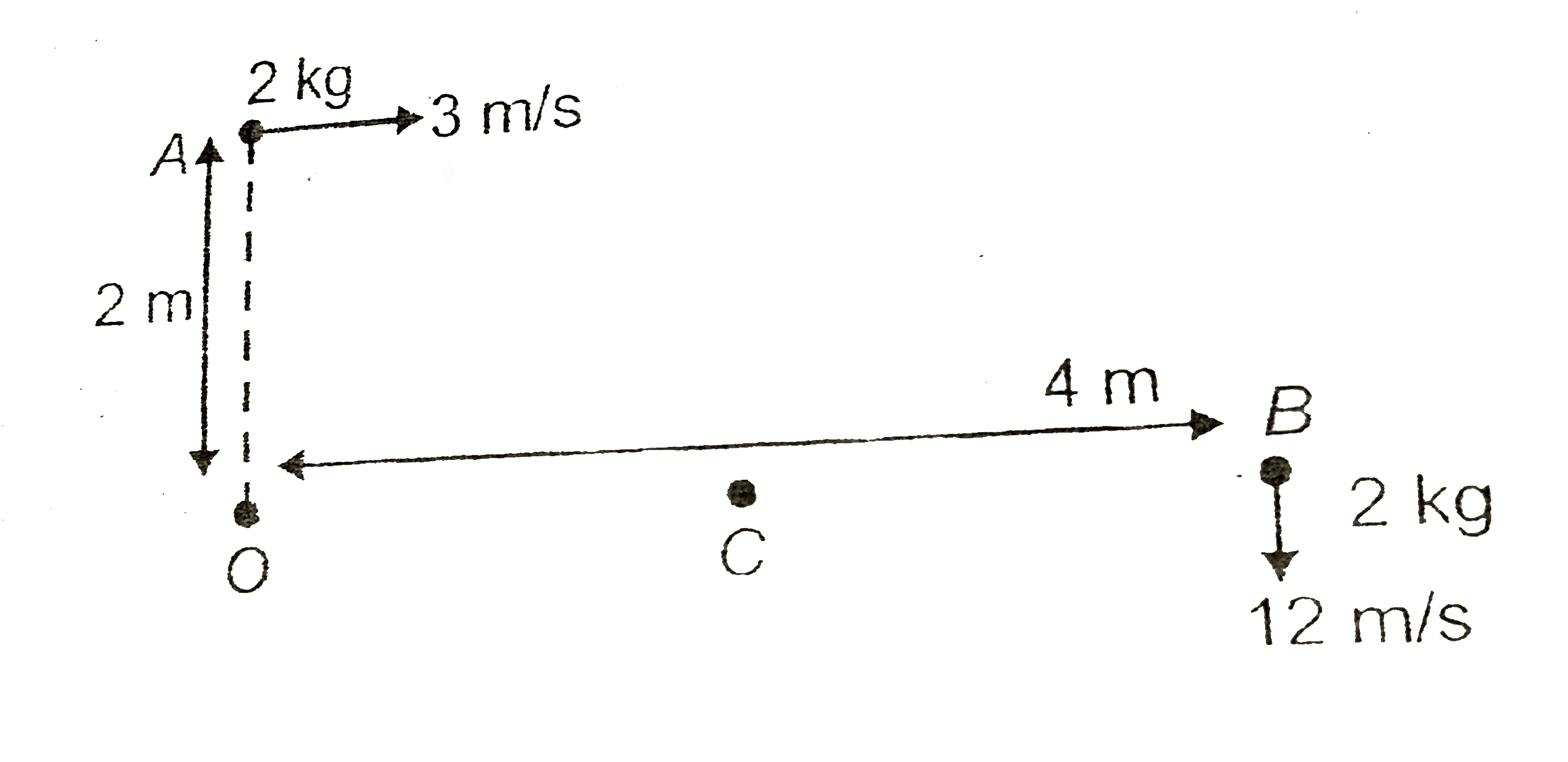
View SolutionThree particles are moving as shown in the figure, Calculate the angular momentum of the third particle C, so that the angular momentum of system about point O becomes zero.
View Solution
