A student connects a cell of emf $ε_{2}$ and internal resistance $r_{2}$ , with a cell of emf $ε_{1}$ such that their combinationhas a net internal resistance less then $r_{1}$ . This combination in the connected across a resistance $R$ . Draw a circuit of the 'set up' and obtain an external for the current flowing through the resistance $R$

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $\frac{ε_{1} r_{2} + ε_{2} r_{1}}{(r_{1} \times r_{2}) + R (r_{2} + r_{1})}$

As the effective internal resistance of two cells is less than the internal resistance $r_{1}$ of one cells so the cell are connected in parallel between the two point $R_{1} and R_{2}$ . The circuit is be the potential difference between point $R_{1} and R_{2}$ . The potential difference between the termianl of first cell is

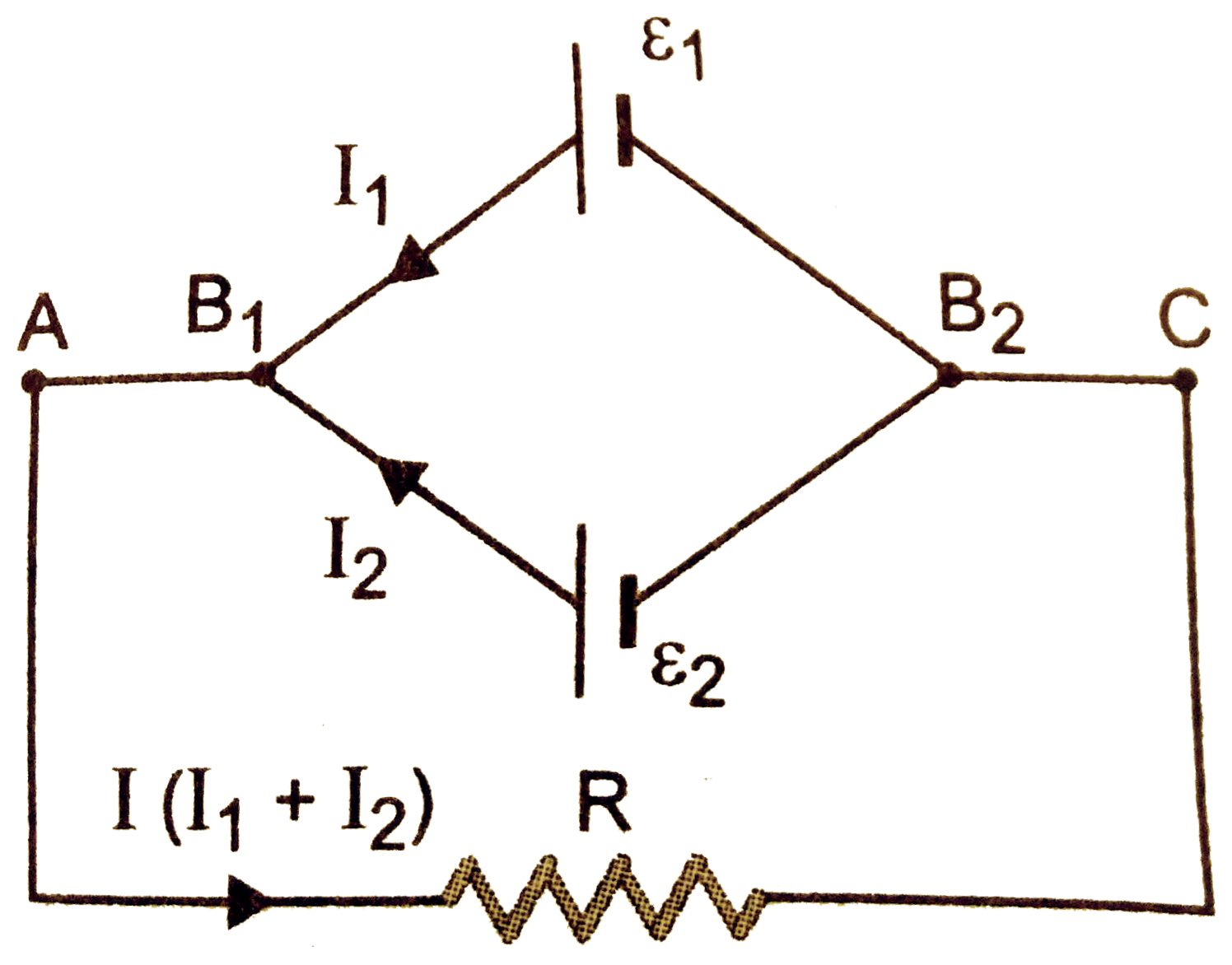
$V = V_{B_{1}} - V_{B_{2}} = ε_{1} - I_{1} r_{1} or I_{1} = \frac{ε_{1} - V}{r_{1}}$
Potential difference between the terminal of second cell is
$V = V_{B_{1}} - V_{B_{2}} = ε_{2} - I_{2} r_{2} or I_{2} = \frac{ε_{2} - V}{r_{2}}$
Hence current in external resistance
$I = I_{1} + I_{2} = \frac{ε_{1} - V}{r_{1}} + \frac{ε_{2} - V}{r_{2}}$
$= (\frac{ε_{1}}{r_{1}} + \frac{ε_{2}}{r_{2}}) - V (\frac{1}{r_{1}} + \frac{1}{r_{2}})$
or $I = (\frac{ε_{1}}{r_{1}} + \frac{ε_{2}}{r_{2}}) - I R (\frac{1}{r_{1}} + \frac{1}{r_{2}}) [∵ V = I R]$
or $I [1 + R (\frac{1}{r_{1}} + \frac{1}{r_{2}})] = (\frac{ε_{1}}{r_{1}} + \frac{ε_{2}}{r_{2}})$
or $I [\frac{r_{1} r_{2} + R (r_{2} r_{1})}{r_{1} r_{2}}] = \frac{ε_{1} r_{2} + ε_{2} r_{1}}{r_{1} r_{2}}$
or $I = \frac{ε_{1} r_{2} + ε_{2} r_{1}}{(r_{1} \times r_{2}) + R (r_{2} + r_{1})}$

|

Updated on:21/07/2023

Class 12 PHYSICS CURRENT ELECTRICITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A cell of emf $ε$ and internal resistance r is charged by a current I, then
Athe cell stores chemical energy at the rate of $ε i$
Bthe cell stores chemical energy at the rate of $i^{2} r$
Cthe cell stores chemical energy at the rate of $ε I - i^{2} r$
Dthe storage of chemical energy rate cannot be calculated
Question 2 - Select One
In a connection of 10 dry cells each of emf E and internal resistance r in series, two cell is connected opposite. Net emf and net internal resistance will be:
A $10 E, 10 r$
B $8 E, 8 r$
C $8 E, 10 r$
D $8 E, \frac{r}{10}$
Question 3 - Select One
Four identical cells of emf $ε$ and internal resistance r are to be connected in series. Suppose, if one of the cell is connected wrongly, then the equivalent emf and effective internal resistance of the combination is
A2E and 4r
B4E and 4r
C2E and 2r
D4E and 2r

PRADEEP-CURRENT ELECTRICITY-Exercise

Home
Ask Doubt
Profile