You are given several identical resistors each of value 10Ω and each capable of carrying a maximum current of 1 A. It is required to make a suitable combination of these to resistances to produce a resistance of 5Ω which can carry a current of 4 A. The minimum number of resistors required for this job is
Video Solution
Text Solution
The correct Answer is:8
Since each part can carry current of one ampere, therefore to pass x A current we need four paths in parallel .Let r be the resistance of each path .The equivalent resistance of a parallel paths will be r/4 According to given problem
r/4=5orr=5×4=20Ω
In order to have 20Ω resistance in each path two resistance each of resistance 10Ω be connected in series. Since there are four paths the total number of resistances required =2×4=8
|
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Jee (main and advance)
4 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Interger Type
2 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Value based
2 Videos
- COMMUNICATION SYSTEMSBOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - MODEL TEST PAPER-2
9 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
191 Videos
Similar Questions
Knowledge Check
IF a person have five resistors each of value 15Ω then the maximum resistance he can obtained by connecting them is
A1ΩB5ΩC10ΩD25ΩIf a person has five resistors each of value 15Ω, then the maximum resistence he can obtain by connecting them is
A1ΩB5ΩC10ΩD25ΩA man has five resistors, each of value (15)Ω. The value of maximum resistance obtained by connecting them will be _____
A12ΩB25ΩC5ΩD1Ω
A parallel combination of three resistors takes a current of 5 A from a 20 V supply. If the resistors are of 10Ωand8Ω find the value of the third resistor.
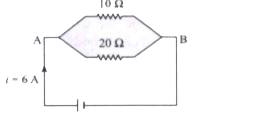
View SolutionTwo resistors of resistance 10Ω and 20Ω are connected in parallel. A battery supplies 6 A of current to the combination. Calculate the current in each resistor.
View SolutionA series combination of two resistors 1Ω each is connected to a 12 V battery of internal resistance 0.4Ω. The current flowing through it will be
View SolutionThe maximum resistance which can be made using four resistors each of resistance 12Ω is
View SolutionA current l=3+8 sin 100t is passing through a resistor of resistance 10 Ω.The effective value of current is
View Solution
