How many lamps each of 50Wand 100Wcan be connected in parallel across a 120V battery of internal resistance 10Ω so the each glows to full power ?
2
4
6
8
The correct Answer is:b
To solve the problem of how many lamps of 50W and 100W can be connected in parallel across a 120V battery with an internal resistance of 10Ω so that each glows at full power, we can follow these steps:
Step 1: Calculate the resistance of each lamp
The resistance R of each lamp can be calculated using the formula:
R=V2P
where V is the voltage across the lamp and P is the power rating of the lamp.
For the 50W lamp:
R50W=120250=1440050=288Ω
For the 100W lamp:
R100W=1202100=14400100=144Ω
Step 2: Calculate the current required by each lamp
The current I through each lamp can be calculated using the formula:
I=PV
For the 50W lamp:
I50W=50120=512A
For the 100W lamp:
I100W=100120=56A
Step 3: Determine the total current required for N lamps
If N lamps are connected in parallel, the total current required for the 50W lamps is:
Itotal,50W=N×I50W=N×512A
For the 100W lamps:
Itotal,100W=N×I100W=N×56A
Step 4: Calculate the total resistance of the circuit
The total resistance Rtotal of the circuit when N lamps are connected in parallel is given by:
Rtotal=Rinternal+Rlamp
Where Rinternal is the internal resistance of the battery (10Ω) and Rlamp is the equivalent resistance of N lamps in parallel.
For N lamps of 50W:
Rlamp,50W=R50WN=288N
Thus,
Rtotal,50W=10+288N
For N lamps of 100W:
Rlamp,100W=R100WN=144N
Thus,
Rtotal,100W=10+144N
Step 5: Apply Ohm's law to find the maximum N
Using Ohm's law, we can set up the equation for the total current from the battery:
I=VRtotal
For the 50W lamps:
N×5121=12010+288N
Cross-multiplying gives:
N×512(10+288N)=120
Solving this equation will yield the maximum number of 50W lamps.
For the 100W lamps:
N×561=12010+144N
Cross-multiplying gives:
N×56(10+144N)=120
Solving this equation will yield the maximum number of 100W lamps.
Final Calculation
Solving the equations for both types of lamps will give us the maximum number of lamps that can be connected in parallel while glowing at full power.
The resistance R of each lamp can be calculated using the formula:
R=V2P
where V is the voltage across the lamp and P is the power rating of the lamp.
R50W=120250=1440050=288Ω
R100W=1202100=14400100=144Ω
The current I through each lamp can be calculated using the formula:
I=PV
I50W=50120=512A
I100W=100120=56A
If N lamps are connected in parallel, the total current required for the 50W lamps is:
Itotal,50W=N×I50W=N×512A
Itotal,100W=N×I100W=N×56A
The total resistance Rtotal of the circuit when N lamps are connected in parallel is given by:
Rtotal=Rinternal+Rlamp
Where Rinternal is the internal resistance of the battery (10Ω) and Rlamp is the equivalent resistance of N lamps in parallel.
Rlamp,50W=R50WN=288N
Thus,
Rtotal,50W=10+288N
Rlamp,100W=R100WN=144N
Thus,
Rtotal,100W=10+144N
Using Ohm's law, we can set up the equation for the total current from the battery:
I=VRtotal
For the 50W lamps:
N×5121=12010+288N
Cross-multiplying gives:
N×512(10+288N)=120
Solving this equation will yield the maximum number of 50W lamps.
N×561=12010+144N
Cross-multiplying gives:
N×56(10+144N)=120
Solving this equation will yield the maximum number of 100W lamps.
Solving the equations for both types of lamps will give us the maximum number of lamps that can be connected in parallel while glowing at full power.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Jee (main and advance)
4 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Interger Type
2 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Value based
2 Videos
- COMMUNICATION SYSTEMSBOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - MODEL TEST PAPER-2
9 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
191 Videos
Similar Questions
A 10 V cell of negligible internal resistance is connected in parallel across a battery of emf 200 V and internal resistance 38 Ω as shown in the Fig. Find the value of current in the circuit.
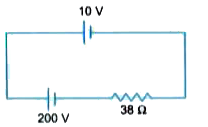
View SolutionA 10 V cell of neglible internal resitsance is connected in parallel across a battery of emf 200 V and internal resistance 38Ω as shown in the figure. Find the value of current in the circuit.

View Solution
Knowledge Check
Two heating coils of resistance 10Ωand20Ω are connected in parallel and connected to a battery of emf 12V and internal resistance 1Ω. The power consumed by them is in the ratio
A1:4B1:3C2:1D4:1two heading coils of resistances 10Ωand20Ω are connected in parallel and connected to a battery of emf 12 V and internal resistance 1Ω Thele power consumed by the n are in the ratio
A1:4B1:3C2:1D4:1Two bulbs 100W,250V and 200W,250V are connected in parallel across a 500V line. Then-
A100W bulb with fusedB200 W bulb will fusedCBoth bulbs will be fusedDNo bulb will fused
An inductor coil of resistance 10Ω and inductance 120 mH is connected across a battery of emf 6 V and internal resistance 2Ω. Find the charge which flows through the inductor in (a) 10 ms, (b) 20ms and (c ) 100ms after the connections are made.
View SolutionHow will you connect ( series and parallel ) 24cells each of internal resistance 1Ω to get maximum power output across a load of 10Ω?
View SolutionA series combination of two resistors 1Ω each is connected to a 12 V battery of internal resistance 0.4Ω. The current flowing through it will be
View SolutionA 25 W , 220 V bulb and a 100 W , 220 V bulb are connected in parallel across a 440 V line
View SolutionTwo electric bulbs rated 50 W and 100 W are glowing at full powr, when used in parallel with a battery of emf 120 V and internal resistance 10 Ω. The maximum number of bulbs that can be connected in the circuit when glowing at full power, is
View Solution
