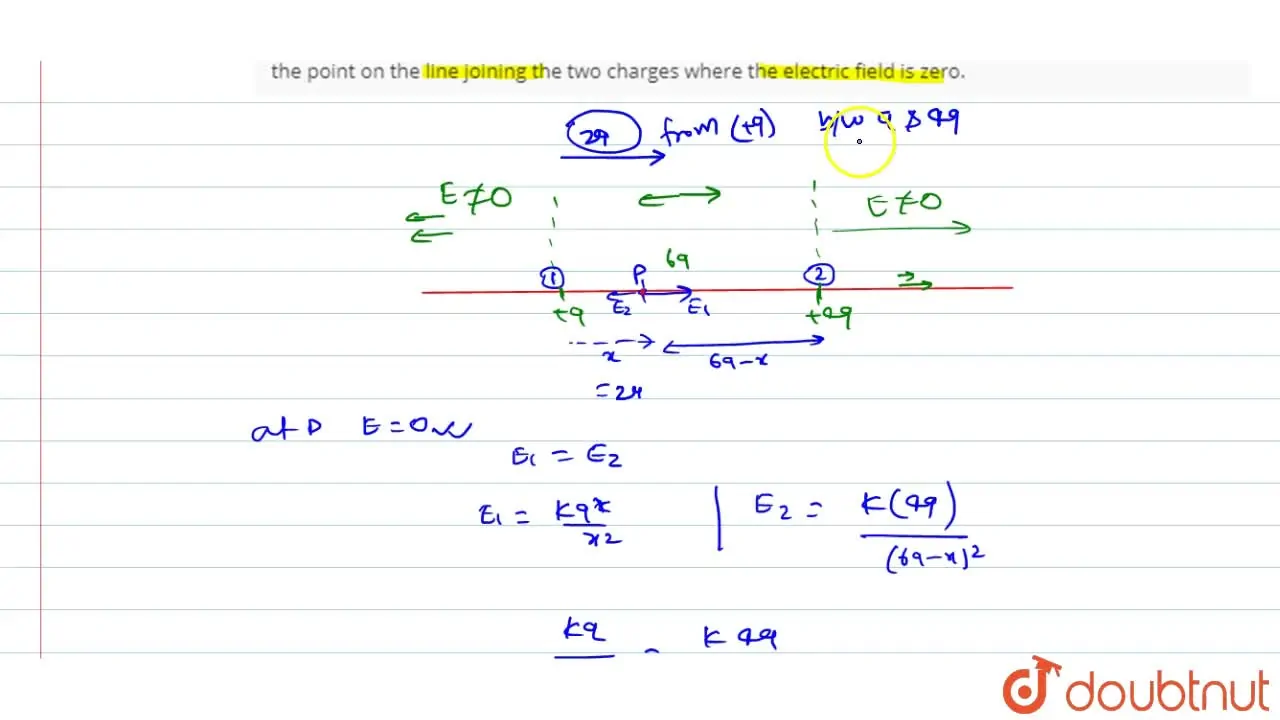
Two electric +qand+4q are placed at a distance 6a apart on a horizontal plane. Find the position of the point on the line joining the two charges where the electric field is zero.
The correct Answer is:2a from +q
Let electric field be zero at P, where AP=x
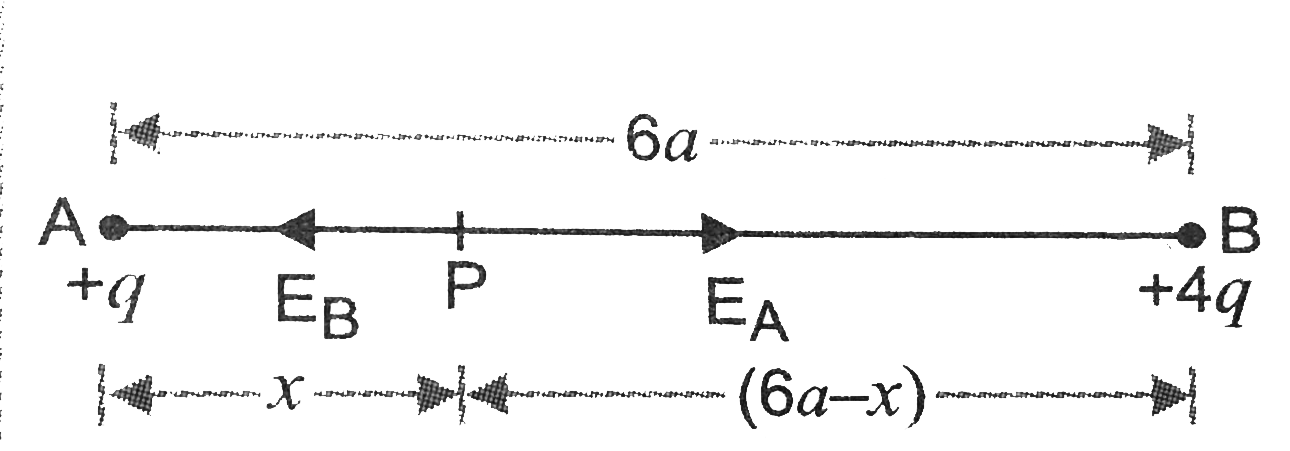
For this EA=EB
kqx2=K(4q)(6a−x)2
(6a−x)2x2=4
x=2a from +q
Related Playlists
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - FILL IN THE BLANKS8videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - PROBLEMS FOR PRACTICE8videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - VALUE BASED QUESTIONS7videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTRONIC DEVICESEXERCISE - Fill in the Blanks1videos
ELECTRONIC DEVICES
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)2videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
Similar Questions
Two electric charges qand−2q are placed at a distance 6 m apart on a horizontal plane. Find the locus of point on this plane where the potential has a value zero.
 .View Solution
.View SolutionTwo electric charges q1=qandq2=−2q are placed at a distance l = 6a apart. Find the locus of points in the plane of the charges where the field potential is zero
View SolutionTwo point charges+ q and-q are placed at distanced apart. What are the points at which the resultant field is parallel to the line joining the two charges ?
View SolutionTwo point objects carry positive charges of magnitude 5e and 20e . Separation between the charges is 1 cm. Find the location of points on the line joining the two charges where the electric field intensity is zero.
View SolutionTwo point charges +4μCand+1μC are separated by a distance of 2 m in air. Find the point on the line joining the two charges at which the net electric field of the system is zero.
View SolutionTwo charge _q and -3q are placed at a distance of 1 m apart.Find out the points on the line joing two charges, where electric potential is zero.
View SolutionTwo charges of 9 and 16 μC are placed 0.7 m apart. Find the position of the null point (A point where the net field is zero) on the line joining the two charges.
View SolutionThe two point charges 4μC and 1μC are separated by a distance of 2 m in air. Find the point on the line joining the charges at which net electric field of the system is zero.
View SolutionTwo point charges +4q and +q are placed 30cm apart. At what point on the line joining them is the electric field zero
View SolutionTwo positive point charges q1=16μC and q2=4μC are separated in vacuum by a distance of 3.0m. Find the point on the line between the charges where the net electric field is zero.-
View Solution