Two charges of 9 and 16 μC are placed 0.7 m apart. Find the position of the null point (A point where the net field is zero) on the line joining the two charges.
To find the position of the null point between two charges of 9 μC and 16 μC that are 0.7 m apart, we can follow these steps:
1. Identify the Charges and Distance:
- Let Q1=9μC=9×10−6C
- Let Q2=16μC=16×10−6C
- Distance between the charges R=0.7m
2. Set Up the Null Point Condition:
- Let R1 be the distance from Q1 (9 μC) to the null point.
- Therefore, the distance from Q2 (16 μC) to the null point will be R2=R−R1=0.7−R1.
3. Electric Field Equation:
- The electric field due to a charge is given by E=k⋅Qr2, where k is Coulomb's constant.
- At the null point, the electric fields due to both charges must be equal in magnitude:
E1=E2
- Thus, we can write:
k⋅Q1R21=k⋅Q2R22
- Since k cancels out, we have:
Q1R21=Q2R22
4. Substituting the Values:
- Substitute Q1 and Q2:
9×10−6R21=16×10−6(0.7−R1)2
5. Cross Multiplying:
- Cross-multiply to eliminate the fractions:
9×10−6⋅(0.7−R1)2=16×10−6⋅R21
6. Dividing by 10−6:
- Simplifying gives:
9(0.7−R1)2=16R21
7. Expanding the Equation:
- Expand the left side:
9(0.49−1.4R1+R21)=16R21
- This simplifies to:
4.41−12.6R1+9R21=16R21
8. Rearranging the Equation:
- Rearranging gives:
0=7R21+12.6R1−4.41
9. Using the Quadratic Formula:
- Apply the quadratic formula R1=−b±√b2−4ac2a:
- Here, a=7,b=12.6,c=−4.41.
- Calculate the discriminant:
b2−4ac=(12.6)2−4⋅7⋅(−4.41)
=158.76+123.48=282.24
- Now substitute into the formula:
R1=−12.6±√282.2414
R1=−12.6±16.8114
10. Calculating the Values:
- Calculate the two possible values for R1:
- R1=4.2114≈0.301m
- R1=−29.4114 (not valid as distance cannot be negative)
11. Conclusion:
- The position of the null point A is approximately R1≈0.301m from the charge of 9 μC.
- Let Q1=9μC=9×10−6C
- Let Q2=16μC=16×10−6C
- Distance between the charges R=0.7m
- Let R1 be the distance from Q1 (9 μC) to the null point.
- Therefore, the distance from Q2 (16 μC) to the null point will be R2=R−R1=0.7−R1.
- The electric field due to a charge is given by E=k⋅Qr2, where k is Coulomb's constant.
- At the null point, the electric fields due to both charges must be equal in magnitude:
E1=E2
- Thus, we can write:
k⋅Q1R21=k⋅Q2R22
- Since k cancels out, we have:
Q1R21=Q2R22
- Substitute Q1 and Q2:
9×10−6R21=16×10−6(0.7−R1)2
- Cross-multiply to eliminate the fractions:
9×10−6⋅(0.7−R1)2=16×10−6⋅R21
- Simplifying gives:
9(0.7−R1)2=16R21
- Expand the left side:
9(0.49−1.4R1+R21)=16R21
- This simplifies to:
4.41−12.6R1+9R21=16R21
- Rearranging gives:
0=7R21+12.6R1−4.41
- Apply the quadratic formula R1=−b±√b2−4ac2a:
- Here, a=7,b=12.6,c=−4.41.
- Calculate the discriminant:
b2−4ac=(12.6)2−4⋅7⋅(−4.41)
=158.76+123.48=282.24
- Now substitute into the formula:
R1=−12.6±√282.2414
R1=−12.6±16.8114
- Calculate the two possible values for R1:
- R1=4.2114≈0.301m
- R1=−29.4114 (not valid as distance cannot be negative)
- The position of the null point A is approximately R1≈0.301m from the charge of 9 μC.
Topper's Solved these Questions
- STATIC ELECTRICITYBOOK - CENGAGE PHYSICSCHAPTER - STATIC ELECTRICITYEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
10 Videos
- STATIC ELECTRICITYBOOK - CENGAGE PHYSICSCHAPTER - STATIC ELECTRICITYEXERCISE - CONSOLIDATED EXERCISE (Multiple Choice Questions with One or More Than One Correct Answer
5 Videos
- SOURCES OF ELECTRIC CURRENTBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF ELECTRIC CURRENTEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
10 Videos
- TRANSFER OF HEATBOOK - CENGAGE PHYSICSCHAPTER - TRANSFER OF HEATEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
10 Videos
Similar Questions
Knowledge Check
Two point charges +4q and +q are placed 30cm apart. At what point on the line joining them is the electric field zero
A15 cm from charge qB20 cm from charge 4 qC7.5 cm from charge qD5cm from charge qThe positions of two point charges q1 and q2 are →r1 and →r2 , respectively. Find the position of the point where the net field is zero due to thses charges.
A→r1√q1+→r2√q2√q1+√q2B→r1√q2+→r2√q1√q1+√q2C→r1√q2+→r2√q1√q1+q2D→r1√q1+→r2√q2√q1+q2Two charges e and 3e are placed at a distance r. The distance of the point where the electric field intensity will be zero is
Ar(1+√3) from 3e chargeBr(1+√3) from e chargeCr(1−√3) from 3e chargeDr1+√13 from e charge
Two point charges2μC and 8μC areplaced 12 cm apart. Find the position of the point where the electric field intensity will be zero.
View SolutionTwo electric charges 9μ c and −3μ c are placed 0.16 m apart in air. There will be a point p at which electric potential is zero on the line joining the two charges and is between them.The distance of p from "9 mu c is .....
View SolutionTwo point charges+ q and-q are placed at distanced apart. What are the points at which the resultant field is parallel to the line joining the two charges ?
View SolutionTwo point charges of +10μC and +20μC are placed in free space 2 cm apart. Find the electric potential at the middle point of the line joining the two charges.
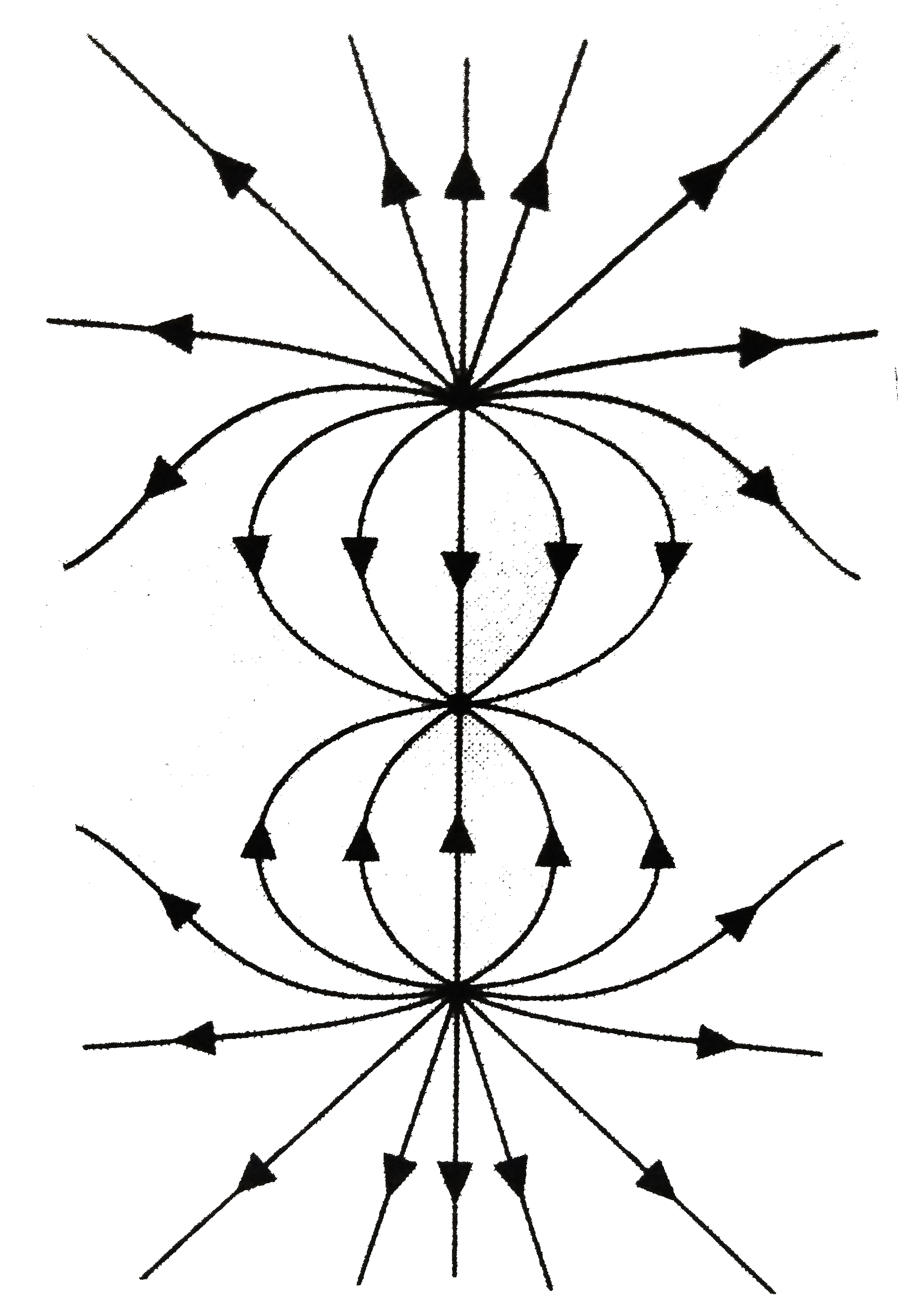
View SolutionFig. shows some of the electric field lines due to three point charges arranged along the vertical axis. All three charges have the same magnitude.
(a) What are the signs of each of the three charges? Explain your reasoning.
(b) At what points(s) is hte magnitude of hte electric field the smallest? Explain your reasoning. Explain how the fields produed by each individual point charge combine to give a small net field at this point or points.
(c ) Two point charges q and -q are placed at a distance d apart. What are the points at which the resultant electric field is parallel to the line joining the two charge?
 .
.
View Solution