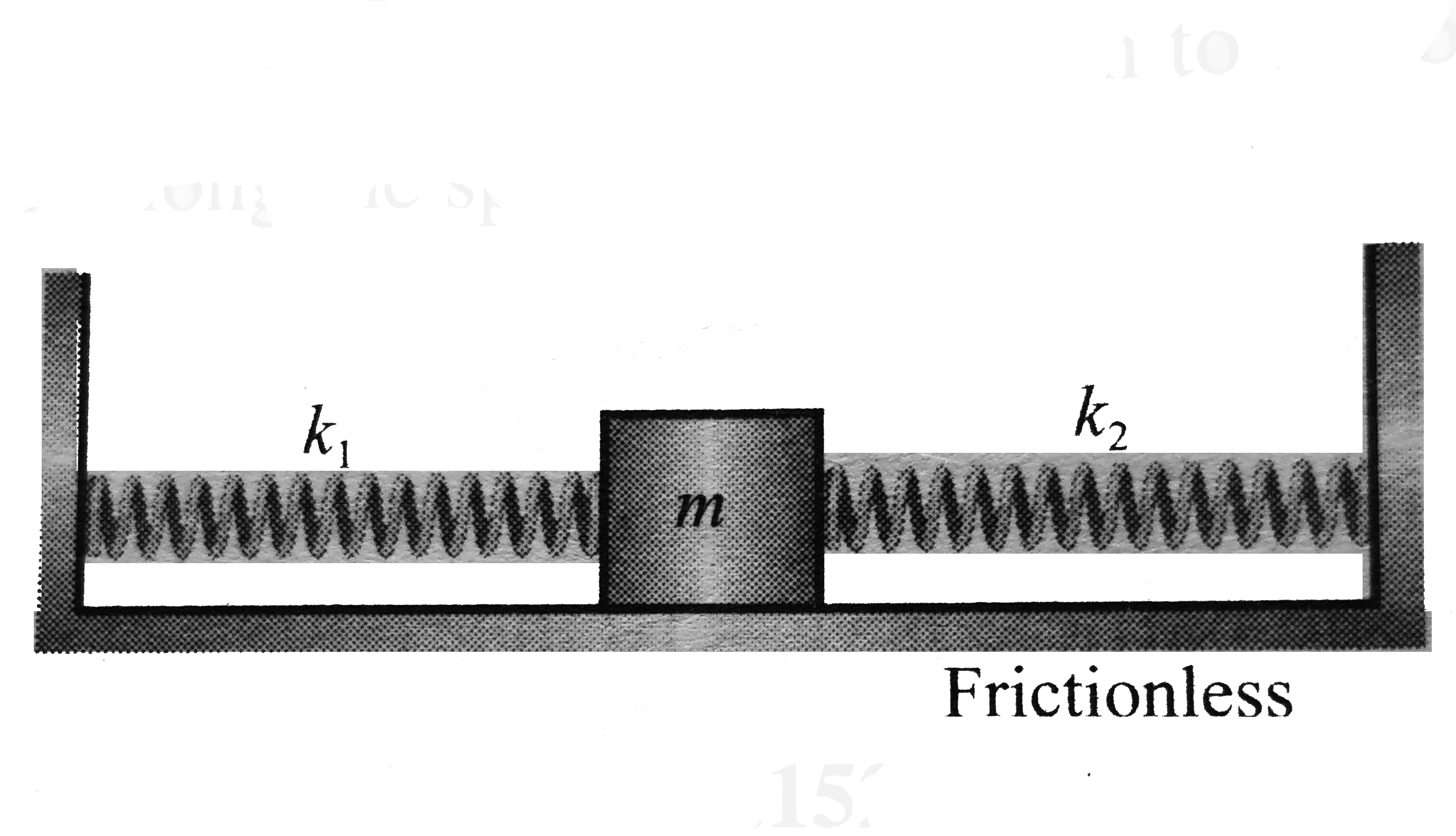
Two springs with negligible masses and force constants k1=200Nm and K2=160Nm are attached to the block of mass m=10kg as shown in the fig. Initially the block is at rest, at the equilibrium position which both springs are neither stretched nor compressed. At time t=0, sharp impulse of 50Ns is given to the block with a hammer along the spring.
Period of oscillations for the mass m is π3s.
Maximum velocity of the mass m during its oscillation is 5ms−1.
Data are insufficient to determine maximum velocity.
Amplitude of oscillation is 0.42m.
The correct Answer is:A, B
Figure
Similar Questions
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
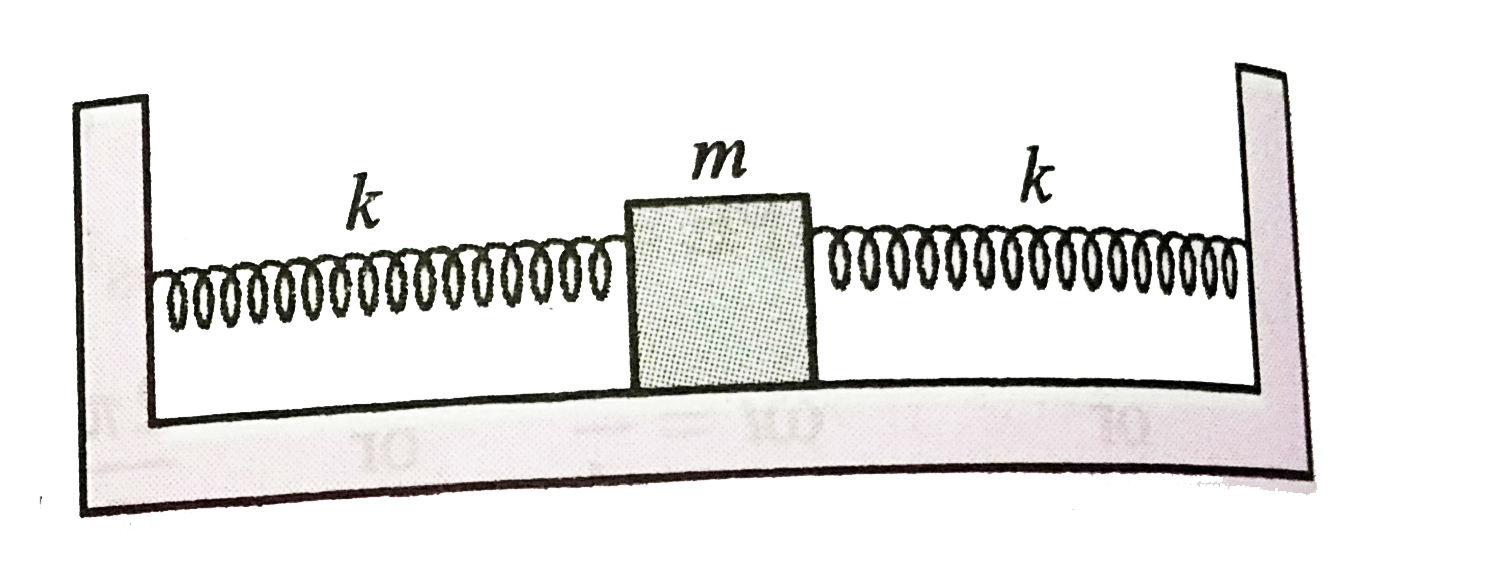
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View Solution
Knowledge Check
Two springs with negligible massess and force constant of k1=200Nm−1 and k2=160Nm−1 are attached to the block of mass m = 10kg as shown in the figure. Initially the block is at rest at the equilibrium position the block is at rest at the equilibrium position nor compressed. At time t=0, sharp impulse of 50 N-s is given to the block.
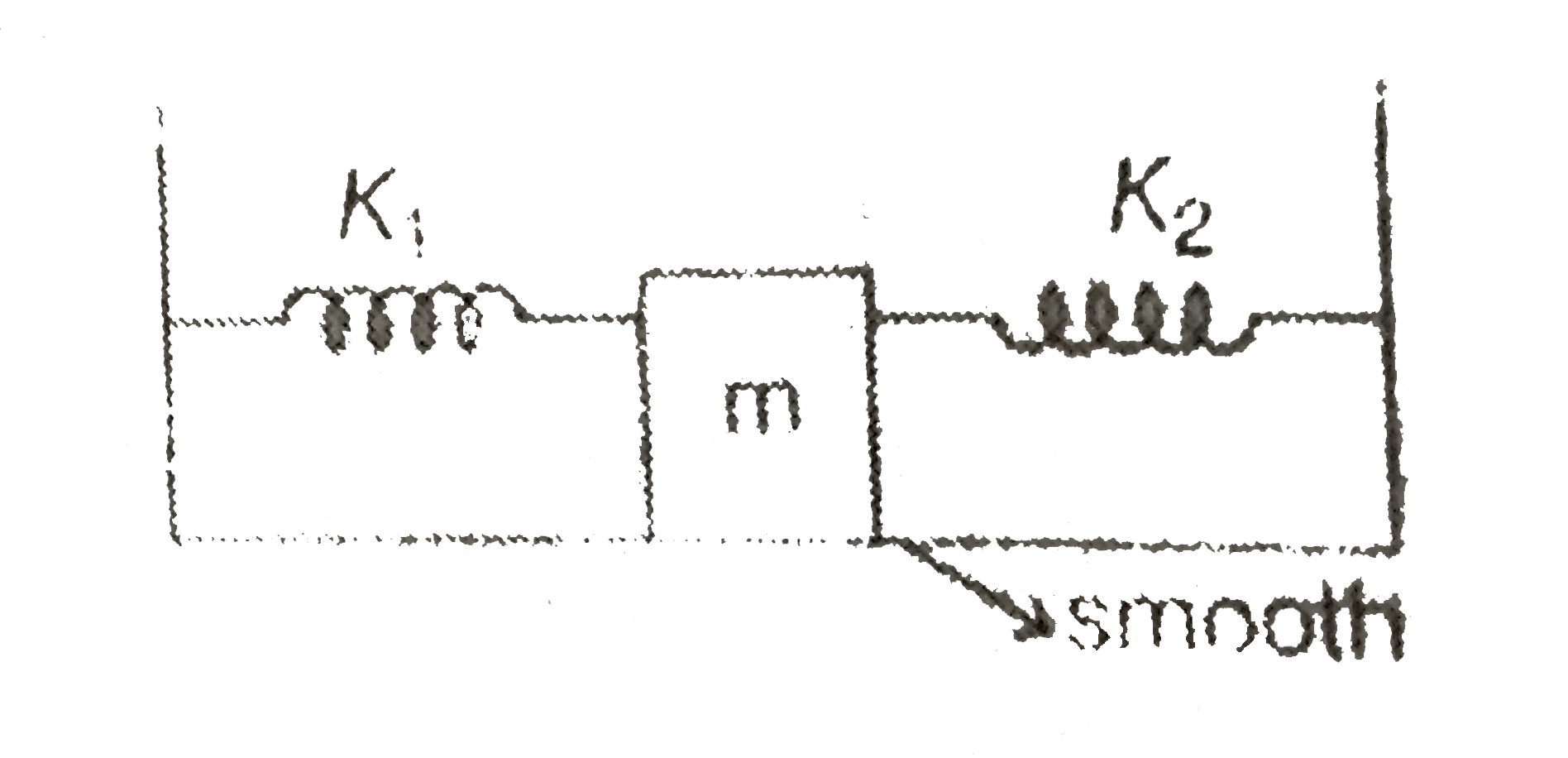 APeriod of oscillations for the mass m is π3 sBmaximum velocity of the mass m during its oscillations is 5ms−1CData are insufficient to determine maximum velocityDAmplitude of oscillation is 0.42 m
APeriod of oscillations for the mass m is π3 sBmaximum velocity of the mass m during its oscillations is 5ms−1CData are insufficient to determine maximum velocityDAmplitude of oscillation is 0.42 mTwo springs with negligible massess and force constant of k1=200Nm−1 and k2=160Nm−1 are attached to the block of mass m = 10kg as shown in the figure. Initially the block is at rest at the equilibrium position the block is at rest at the equilibrium position ir. Which both springs are neither stretched nor compressed. At time t = 0, sharp impulse of 50 N-s is given to the block in horizontal direction.
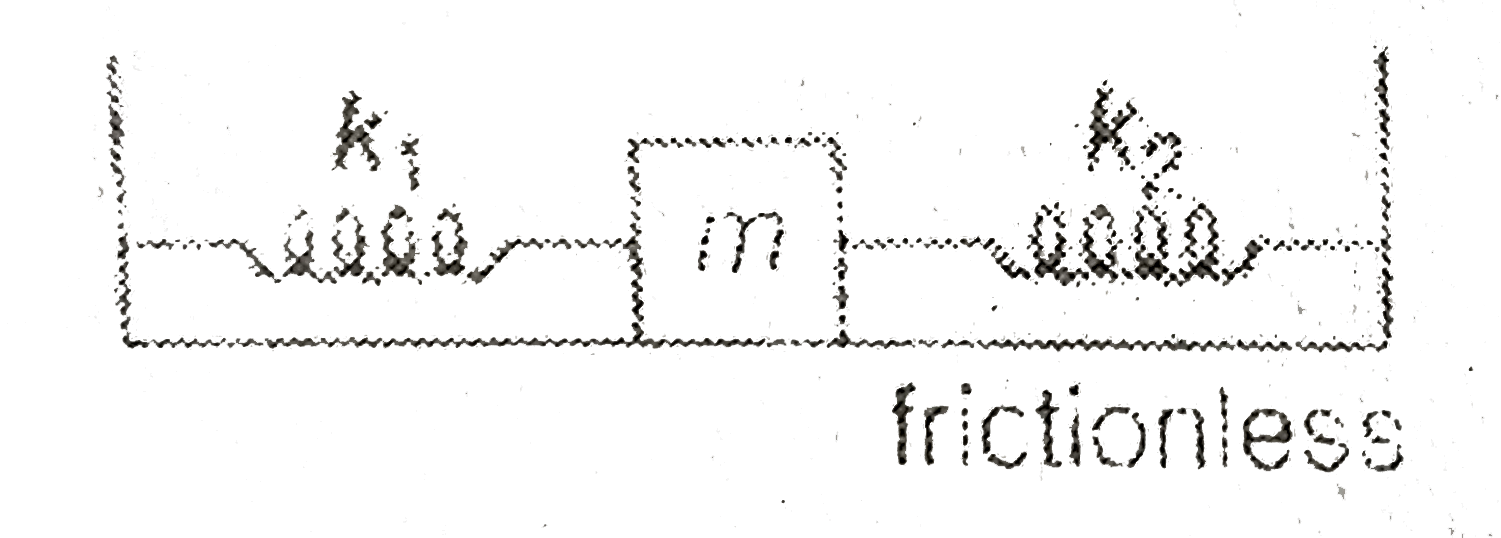 Aperiod of oscialltions for the mass m is (π6) sBmaximum velocity of the mass m during its oscillation is 10ms−1.Cmaximum velocity is 6m/sDamplitude of oscillations is 56m.
Aperiod of oscialltions for the mass m is (π6) sBmaximum velocity of the mass m during its oscillation is 10ms−1.Cmaximum velocity is 6m/sDamplitude of oscillations is 56m.Two springs of spring constant 6N/m and 4N/m are attached to a block of mass 1 kg and to fixed support. Then time period of the given oscillation is
(consider √10=π)A1sB2sC0.5sD4s
Block of mass m=10kg is suspended by two springs vertically as shown in the diagram. The block is at rest initially. Find the acceleration of block just after cutting the right spring.
View SolutionA block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :

View SolutionA block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :

View SolutionA spring is connected between two blocks as shown in the diagram. Initially the blocks are held stationary and suddenly they are released. Find the ratio of maximum kinetic energy with spring compressed the block of mass m to that of mass 2m.
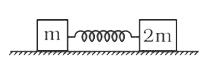
View Solution
A block of mass m initially at rest is dropped from a height h on to a spring of force constant k . the maximum compression in the spring is x then
View Solution