Two particles A to B perform SHM along the same stright line with the same amplitude 'a' same frequency 'f' and same equilbrium position 'O'. The greatest distance between them is found to be 3a/2. At some instant of time they have the same displacement from mean position. what is the displacement?
a/2
a√74
√3a2
3a4
The correct Answer is:B
To solve the problem, we need to find the displacement of two particles A and B performing Simple Harmonic Motion (SHM) along the same straight line, given that they have the same amplitude, frequency, and equilibrium position, and the maximum distance between them is 3a2.
1. Understanding the Problem:
- Both particles A and B have the same amplitude a and frequency f.
- The maximum distance between them is given as 3a2.
- At some instant, both particles have the same displacement from the mean position.
2. Setting Up the Displacement:
- Let the displacement of particle A from the mean position be xA and that of particle B be xB.
- Since they have the same amplitude and frequency, we can express their displacements as:
xA=asin(ωt+ϕA)
xB=asin(ωt+ϕB)
- Here, ϕA and ϕB are the phase angles of particles A and B, respectively.
3. Using the Maximum Distance:
- The greatest distance between the two particles is given by:
|xA−xB|=3a2
- This can be rewritten as:
|asin(ωt+ϕA)−asin(ωt+ϕB)|=3a2
- Dividing both sides by a (assuming a≠0):
|sin(ωt+ϕA)−sin(ωt+ϕB)|=32
4. Using the Sine Difference Identity:
- We can use the sine difference identity:
|sinA−sinB|=2∣∣∣cos(A+B2)sin(A−B2)∣∣∣
- Let A=ωt+ϕA and B=ωt+ϕB.
- Thus:
2∣∣
∣∣cos((ωt+ϕA)+(ωt+ϕB)2)sin((ωt+ϕA)−(ωt+ϕB)2)∣∣
∣∣=32
- Simplifying gives:
∣∣∣cos(2ωt+ϕA+ϕB2)sin(ϕA−ϕB2)∣∣∣=34
5. Finding the Displacement:
- At the instant when both particles have the same displacement, we can set xA=xB.
- This implies:
asin(ωt+ϕA)=asin(ωt+ϕB)
- Therefore, the angles must be equal or differ by π:
ϕA=ϕBorϕA=ϕB+π
6. Calculating the Displacement:
- If ϕA=ϕB, then both particles are at the same position, which contradicts the maximum distance condition.
- If ϕA=ϕB+π, we have:
xA=−xB
- The average displacement when they are at maximum separation can be calculated as:
x=a2 (since they are at opposite extremes)
- Thus, the displacement at that instant when they have the same displacement is:
x=a4√7
Final Answer:
The displacement of the particles when they have the same displacement from the mean position is a4√7.
- Both particles A and B have the same amplitude a and frequency f.
- The maximum distance between them is given as 3a2.
- At some instant, both particles have the same displacement from the mean position.
- Let the displacement of particle A from the mean position be xA and that of particle B be xB.
- Since they have the same amplitude and frequency, we can express their displacements as:
xA=asin(ωt+ϕA)
xB=asin(ωt+ϕB)
- Here, ϕA and ϕB are the phase angles of particles A and B, respectively.
- The greatest distance between the two particles is given by:
|xA−xB|=3a2
- This can be rewritten as:
|asin(ωt+ϕA)−asin(ωt+ϕB)|=3a2
- Dividing both sides by a (assuming a≠0):
|sin(ωt+ϕA)−sin(ωt+ϕB)|=32
- We can use the sine difference identity:
|sinA−sinB|=2∣∣∣cos(A+B2)sin(A−B2)∣∣∣
- Let A=ωt+ϕA and B=ωt+ϕB.
- Thus:
2∣∣
∣∣cos((ωt+ϕA)+(ωt+ϕB)2)sin((ωt+ϕA)−(ωt+ϕB)2)∣∣
∣∣=32
- Simplifying gives:
∣∣∣cos(2ωt+ϕA+ϕB2)sin(ϕA−ϕB2)∣∣∣=34
- At the instant when both particles have the same displacement, we can set xA=xB.
- This implies:
asin(ωt+ϕA)=asin(ωt+ϕB)
- Therefore, the angles must be equal or differ by π:
ϕA=ϕBorϕA=ϕB+π
- If ϕA=ϕB, then both particles are at the same position, which contradicts the maximum distance condition.
- If ϕA=ϕB+π, we have:
xA=−xB
- The average displacement when they are at maximum separation can be calculated as:
x=a2 (since they are at opposite extremes)
- Thus, the displacement at that instant when they have the same displacement is:
x=a4√7
The displacement of the particles when they have the same displacement from the mean position is a4√7.
Similar Questions
Two particle A and B execute SHM along the same line with the same amplitude a, same frequency and same equlibrium position O. If the phase difference between them is ϕ=2sin−1(0.9) then find maxium distance between two.
View SolutionTwo particles P and Q describe S.H.M. of same amplitude a, same frequency f along the same straight line from the same mean position. The maximum distance between the two particles is a √2, If the initial phase difference between the particles is πN then find N :
View Solution
Knowledge Check
Two particles are performing SHM in same phase. It means that
Athe two partcles must have same distance from the mean posotion simultaneouslyBtwo particle may have same distance from the mean position simultaneouslyCthe two particles must have maximum speed simultaneouslyDthe two particles may have maximum speed simultaneouslyTwo particles execute SHM of same amplitude of 20cm with same period along the same line about the same equilibrium position. If phase difference is π/3 then the maximum distance between these two will be
A10cmB20cmC10√2cmD20√2cmTwo particles P and Q describe simple harmonic motions of same period , same amplitude along the same line about the same equilibrium position O. When P and Q are on opposite sides of O at the same distance from O they have the same speed of 1.2m/s in the same direction, when their displacements are the same they have the same speed of 1.6m/s in opposite directions .The maximum velocity in m/s of either particle is
A2.8B2.5C2.4D2
Two particle execute SHM of same amplitude of 20cm with same period along the same line about the same equilibrium position. The maximum distance between the two is 20cm. Their phase difference in radians is
View SolutionTwo particles P and Q describe S.H.M. of same amplitude a, same frequency f along the same straight line. The maximum distance between the two particles is a√2 . The initial phase difference between the particle is –
View SolutionTwo particles P and Q describe S.H.M. of same amplitude a, same frequency f along the same straight line from the same mean position. The maximum distance between the two particles is a √2, If the initial phase difference between the particles is πN then find N :
View SolutionThe figure shows a graph between velocity and displacement (from mean position) of a particle performing SHM:
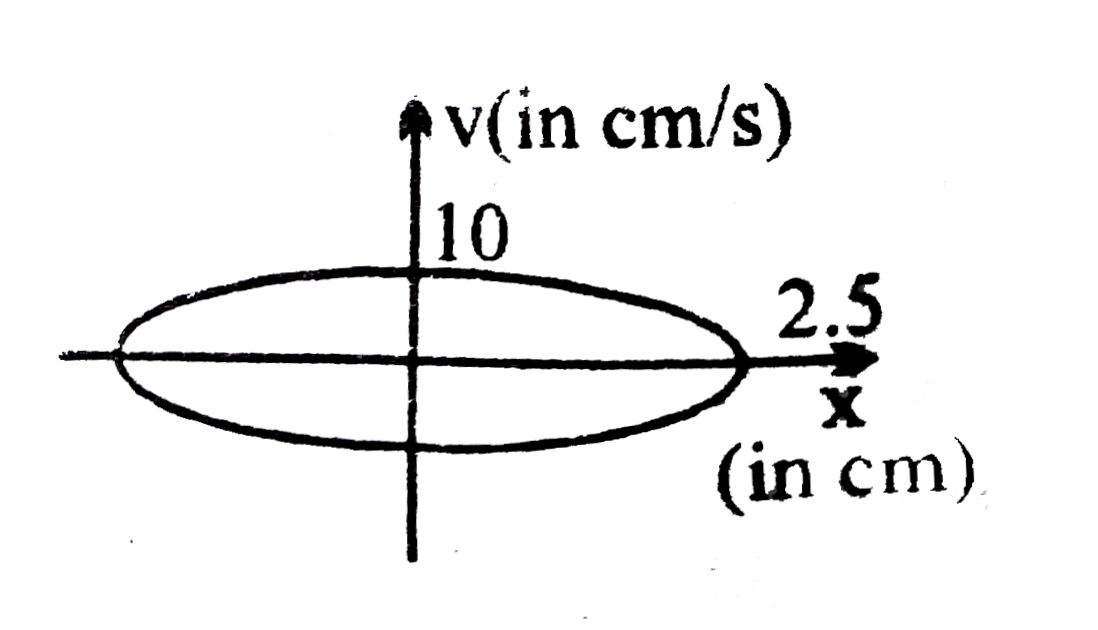
View SolutionThe period of a simple harmonic oscillator is 2 s. If it crosses mean position at an instant. The time after which its displacement from the mean position will be half of the amplitude is.
View Solution
