A mass m is attached to a pulley through a cord as shown in figure. The pulley is a solid disk with radius R. The cord does not slip on the disk. The mass is released from rest at a height h from the ground and at the instant the mass reaches the ground, the disk is rotating with angular velocity ω. Find the mass of the disk.
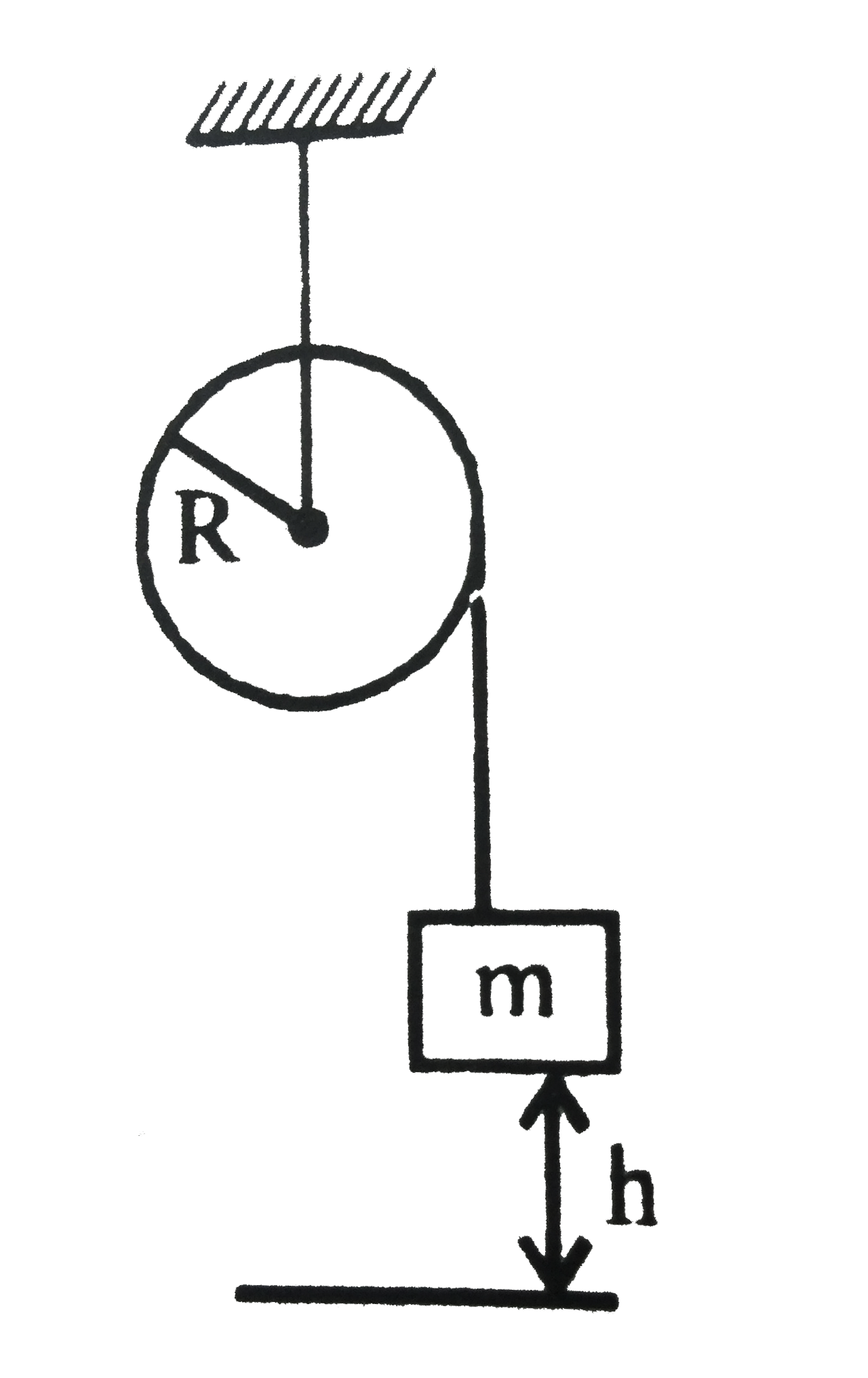 .
.
The correct Answer is:(i) M=2m(2ghR2ω2−1)
By work Energy Theorem
WDg=ΔKE
mgh=12mv2+12ω2
mgh=12m(Rω)2+12×(12MR2)ω2
(M = mass of disc)
mgh=12mR2ω2+14MR2ω2
mgh−12MR2ω2=14MR2ω2
M=4(mgh−12mR2ω2)R2ω2
M=2m(2ghR2ω2−1).
Similar Questions
A cord is wrapped on a pulley (disk) of mass M and radius R as shown in figure. To one end of the cord, a block of mass M is connected as shown and to other end in (a) a force of 2 Mg and in (b) a block of mass 2 M. Let angular acceleration of the disk in (a) and (b) is alpha_(A) and alpha_(B) respectively, then (cord is not slipping on the pulley)
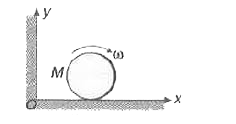
View Solutionद्रव्यमान M तथा त्रिज्या R की एक डिस्क चित्रानुसार कोणी चाल ω से क्षैतिज तल पर लुढृक रही है। मूलबिंदु O के परितः डिस्क के कोणीय संवेग का परिमाण है
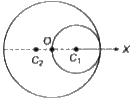
View SolutionR त्रिज्या की एक वृत्ताकार डिस्क को 2R त्रिज्या की एक बड़ी वृत्ताकार डिस्क से इस प्रकार काटा जाता है कि दोनों डिस्क की परिधि संपाती हो। नयी डिस्क के द्रव्यमान केन्द्र की बड़ी डिस्क के द्रव्यमान केन्द्र से दूरी αR है। α का मान है
View SolutionFigure shows a uniform disk, with mass M = 2.5 kg and radius R = 20 cm, mounted on a fixed horizontal axle. A block with mass m = 1.2 kg hangs from a massless cord that is wrapped around the rim of the disk. Find the acceleration of the falling block, the angular acceleration of the disk, and the tension in the cord. The cord does not slip, and there is no friction at the axle.
View SolutionA cord with negligible mass is wrapped around a pulley that is a uniform disk of mass 5.00 kg and radius 0.300 m and that can rotate without friction about its central axis. A 1.0 kg bucket is attached at the free end of the cord hanging down from the pulley and then released at time t = 0. The cord begins to unwrap from the pulley as the bucket descends. At t = 5.00 s, through how many rotations has the pulley turned (the bucket is still descending)?
View Solutionत्रिज्या R की एक समान वृत्ताकार डिस्क से त्रिज्या R/4 की एक समाक्ष छोटी वृत्ताकार डिस्क काट ली गई है। यदि सम्पूर्ण डिस्क का केंद्र O हो तो शेष डिस्क के द्रव्यमान केंद्र की O से दूरी होगी
View Solutionएक पतली वृत्ताकार चकती जिसका द्रव्यमान M तथा त्रिज्या r है, अपने अक्ष के अनुदिश नियत कोणीय वेग ω से घूर्णन कर रही है। दो वस्तुएँ जिनमें प्रत्येक का द्रव्यमान m है, चकती के व्यास के दोनों सिरों से मृदुलता से जुड़ी हैं। अब चकती जिस कोणीय वेग से घूर्णन करेगी वह है
View Solutionm द्रव्यमान डोरी के द्वारा घिरनी से दर्शाए अनुसार संयोजित है। घिरनी R त्रिज्या की एक ठोस चकती है। डोरी (cord) चकती पर फिसलती नहीं है। द्रव्यमान को धरातल से hऊँचाई पर विरामावस्था से मुक्त किया जाता है तथा उस क्षण पर द्रव्यमान धरातल तक पहुँचता है, जब चकती ω कोणीय वेग से घुर्णन कर रही है। चकती का द्रव्यमान ज्ञात कीजिए।

View Solution