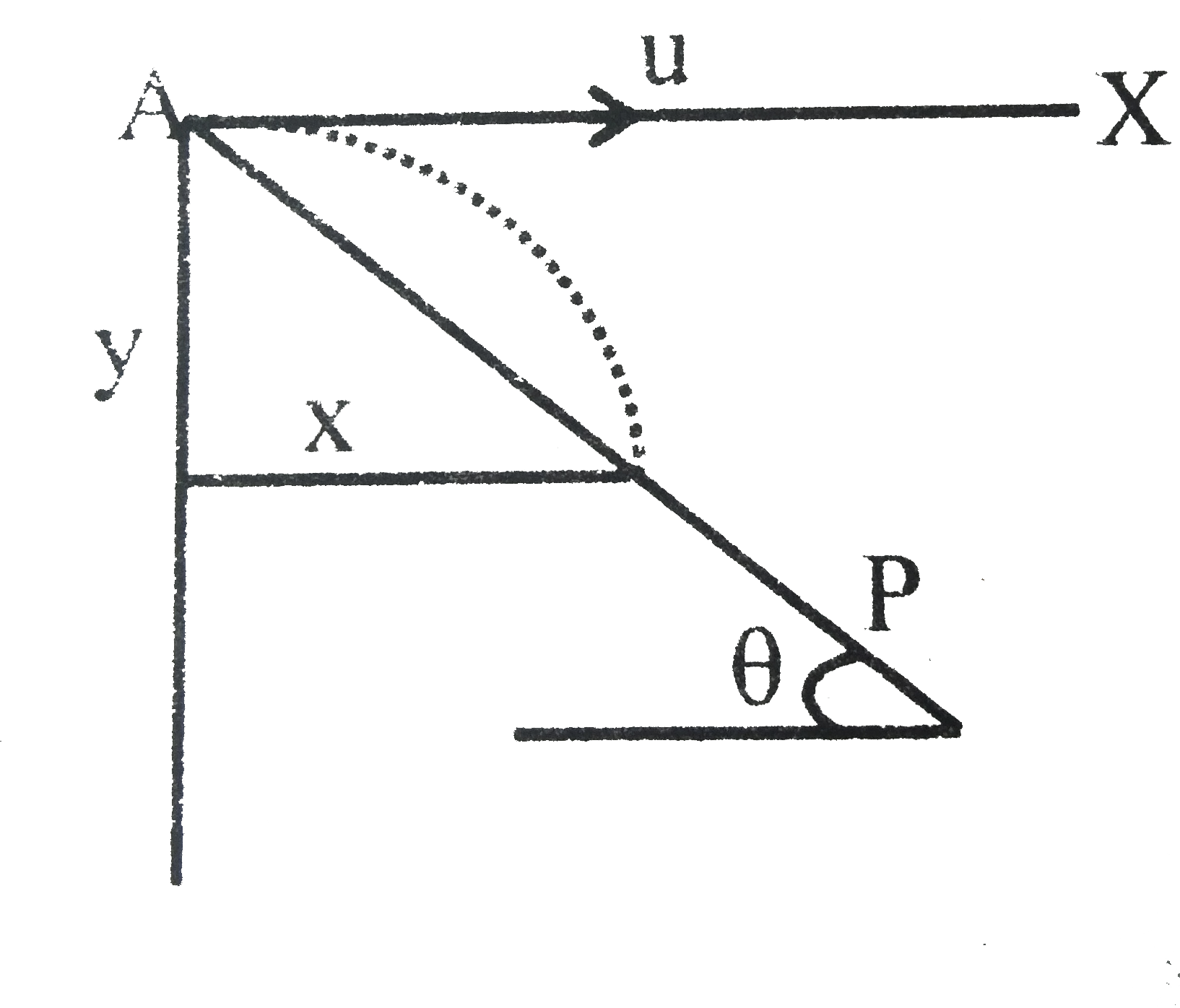
A pariicle is projected horizontally with a speed (u) from top of a plance inclined at anangle θ with the horizontal direction. How far from the point of projection will the particle strike the plane ?
Suppose that the particle strikes the plane at a point P with corrdinates (x,y). Consider the motion between points A and P by resolving them into two directions
Motion in x-direction
Initial velocity =u,
acceleration =0
∴x=ut...(i)
Motion in y-direction
Initial velocity =0,
acceleration =g,
y =(½) "gt"^(2) ...(ii)
Eliminating t from (i) & (ii)
y=12g(x2)u2
Also, y=xtanθ
Therefore gx22u2=xtanθ⇒gx22u2−xtanθ=0
⇒x(gx2u2−tanθ)=0⇒x2u2tanθg...(iii)
Thus, y=xtanθ=2u2tan2θg...(iv)
∴ Distance AP=√x2+y2=2u2gtanθ
=√(1+tan2θ)=2u2gtanθsecθ,
Similar Questions
A particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?

View SolutionA particle is projected horizontal with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane?
View Solution
Knowledge Check
A ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
A2v2gB√2[2v2g]Cv2gD√2v2gA particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
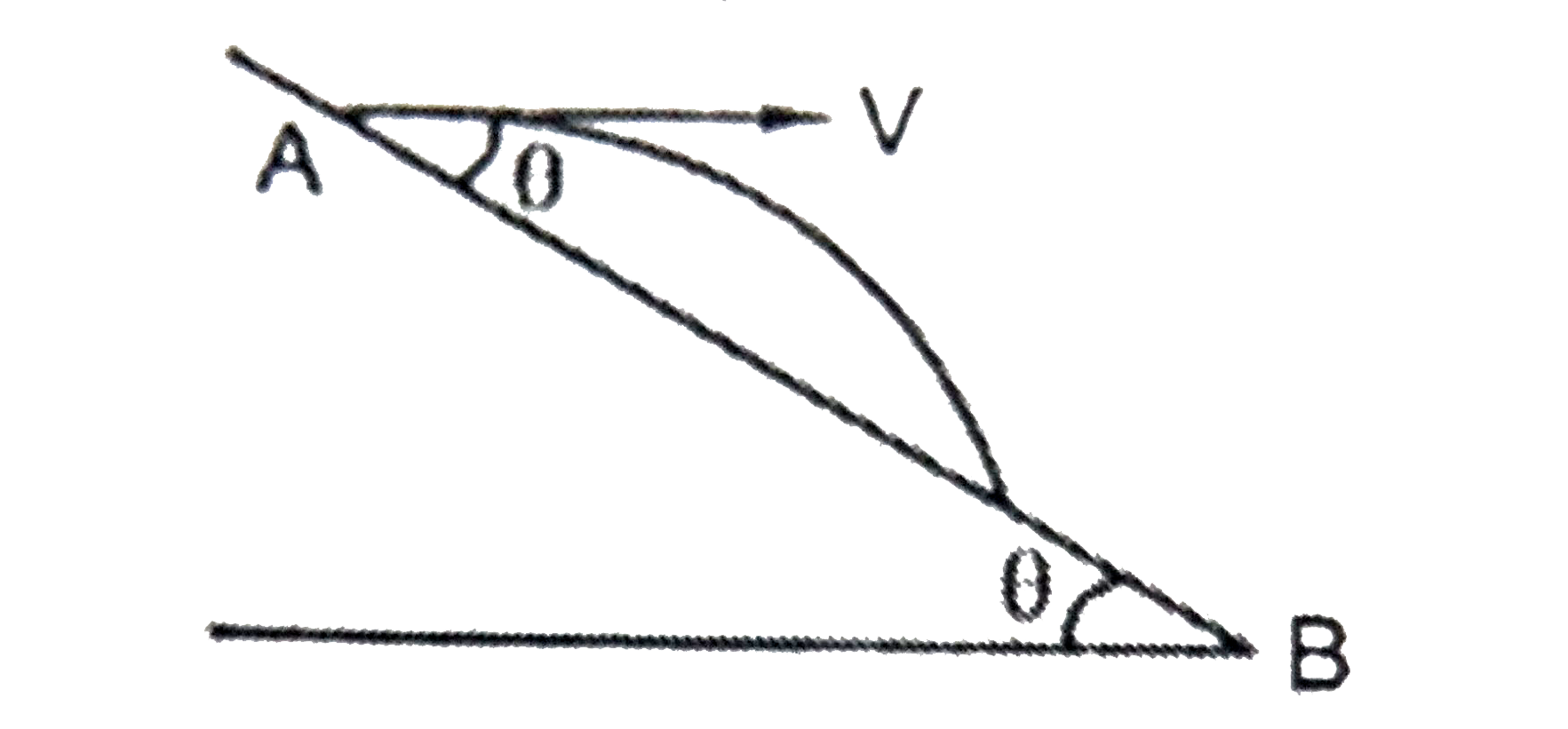
How far from the point of projection will the particle strike the plane?A75mB6516mC7516mD859mA particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
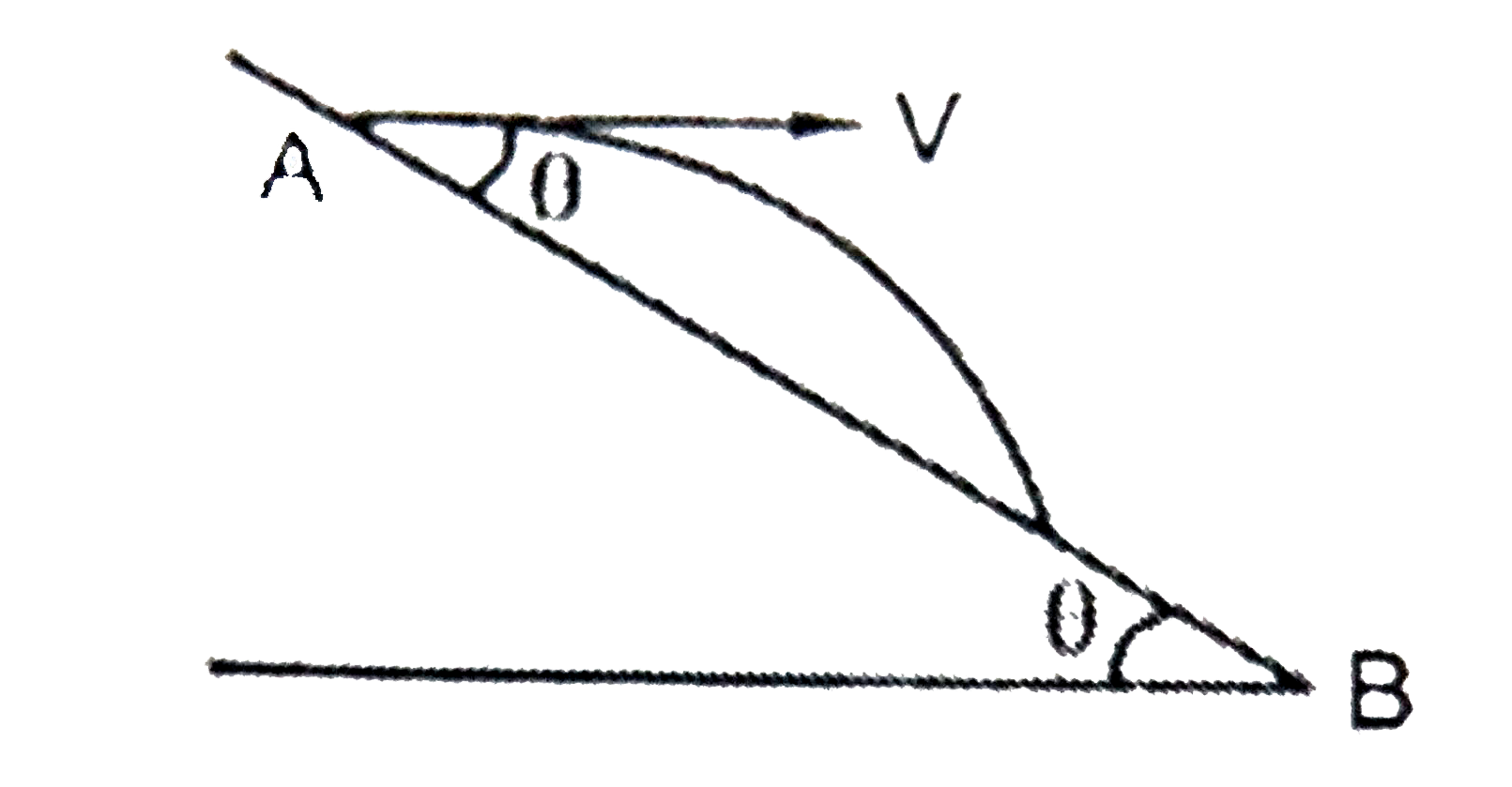
Find the time taken by the particle to hit the plane.A34sB3sC4sD43s
A particles is projected horizontally with a speed v from the top of a plane inclined at an angle tehta to the horizontal as shown in the figure.
(a) Hwo far from the point of projection will the particle strike the plane ?
(b) Find the time taken by the particel to hit the plane.
(c) What is the velocity of particle just before it hits the plane ?
View SolutionA particle is thrown horizontally from the top of an inclined plane , with a speed of 5m/s . If the inclination of the plane is 30∘ with the horizontal , how far from the point of projection will the particle strike the plane ?
View SolutionA ball is projected horizontally with a speed u from the top of inclined plane of inclination α with horizontal. At what distance along the plane, the ball will strike the plane ?
View SolutionA particle of mass m is projecte dwilth speed u at an angle θ with the horizontal. Find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View SolutionA particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
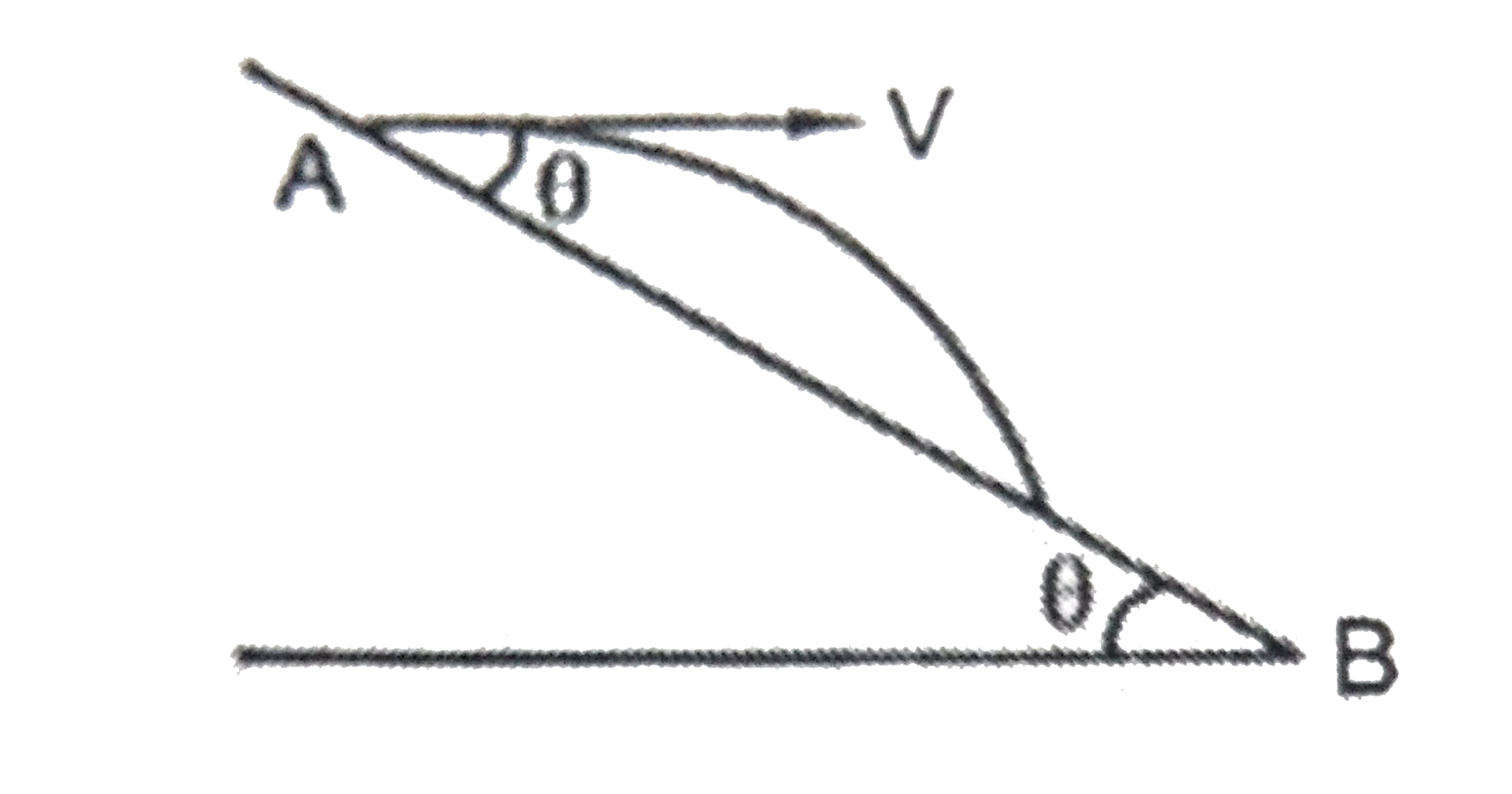
What is the velocity of the particle just before it hits the plane?
View Solution