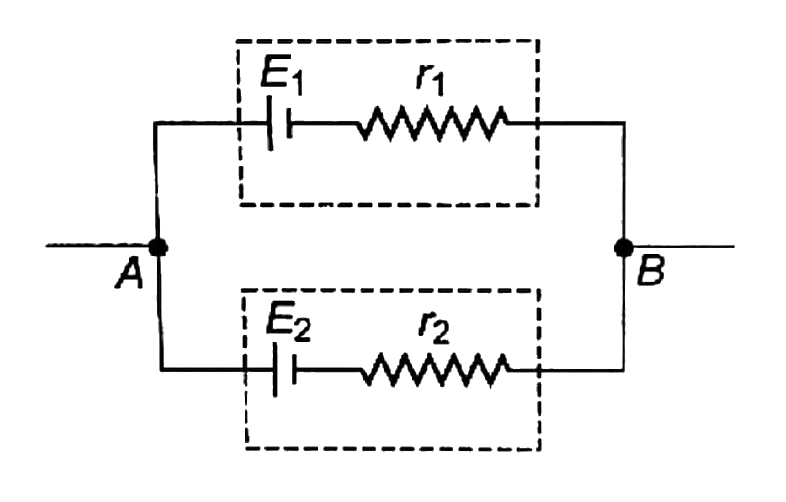
When two cells of emf E1E2 and interval resistance r1 and r2 are connected in parallel between two points A and B, find the values of effective internal resistance of two cells and their effective emf.
Step by step video & image solution for When two cells of emf E_(1) E_(2) and interval resistance r_(1) and r_(2) are connected in parallel between two points A and B, find the values of effective internal resistance of two cells and their effective emf. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - MODEL TEST PAPER-29videos
COMMUNICATION SYSTEMS
- BOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - Multiple Choice Question4videos
COMMUNICATION SYSTEMS
- BOOK - PRADEEPCHAPTER - ATOMS AND NUCLEIEXERCISE - Assertion- Reason type question 121videos
ATOMS AND NUCLEI
- BOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Problems for Practice (B)2videos
CURRENT ELECTRICITY
Similar Questions
Two batteries of e.m.f. E1 and E2 and internal resistance r1 and r2 are connected in parallel. Determine their equivelent e.m.f.
View SolutionTwo cells of emf E1andE2 having internal resistance r1andr2 are connected in parallel. Calculate Eeq and req for the combination.
View SolutionTwo batteris of emf ε1 and ε2 wit respective internal resistance r1 and r2 are connected in parallel. Now
View SolutionTwo cells of emf 1.25V,0.75V and each of internal resistance 1Ω are connected in parallel. The effective emf will be
View SolutionTwo cells of emf 2ε and ε and internal resistance 2r and r respectively, are connected in parallel. Obtain the expressions for the equivalent emf and the internal resistance of the combination.
View SolutionTwo cells of emfs ε1andε2 and internal resistances r1andr2 respectively are connected in parallel . Obtain expressions for the equivalent (i) resistance and , (ii) emf of the combination.
View SolutionTwo cells of emf ε1 and ε2 having internal resistances r1 and r2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the pointsB1 and B2 .
 View Solution
View SolutionTwo cells of equal e.m.f. E b ut of difference internal resistance r1 and r2 are connected in parallel and the combination is connected with an external resistance R to obtain maximum power in R. find the value of R
 View Solution
View SolutionIf n cells each of emf E and internal resistance are connected in parallel, then the total emf and internal resistances will be
View SolutionTwo batteries of emf E1 and E2(E2>E1) and internal resistance r1 and r2 respectively are connected in parallel as shown in the figure. Then, which of the followings statements is correct ?
 View Solution
View Solution


