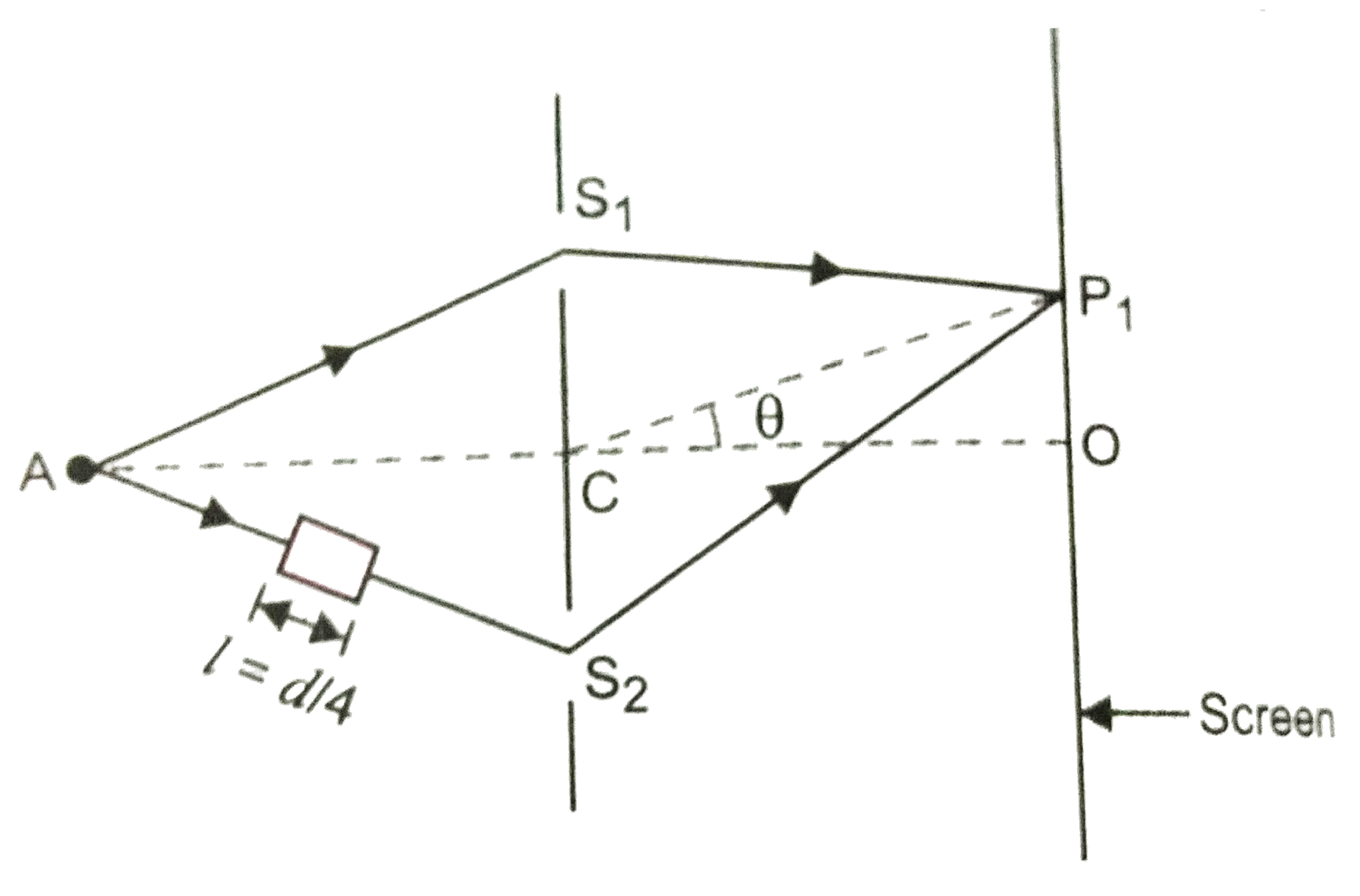
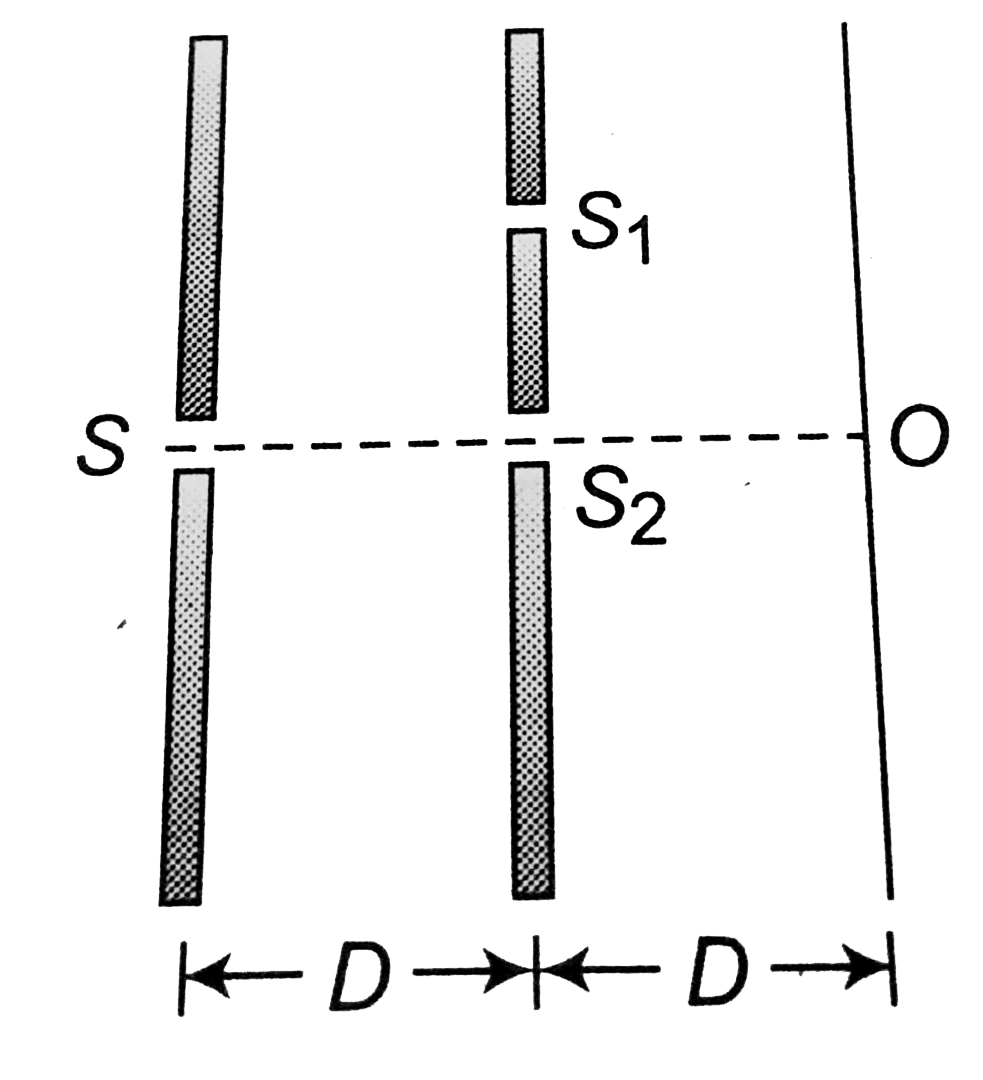
A small transparent slab (μ=1.5) is placed along AS2 as shown.

AC=CO=D,S1C=S2S=d<<D
The distance of princpal maxima front 'O' is
As is clear from Fig. path difference between waves reaching P1 from A is
=2dsinθ+(μ−1)l.
For principal maximum, path diff. =0.
i.e., 2dsinθ+(μ−1)l=0
2d=sinθ+(1.5−1)d4=0,sinθ=−116
∴OP1=(CO)tanθ≅D(−116)
For the first minimum, a angle θ1, say,
path difference =2dsinθ1+0.5l=±λ/2
sinθ1=±λ/2−0.5l2d
As diffraction ocuurs when d=λ,∴sinθ1=±λ/2−λ/82λ=±14−116
on the positive side, sinθ1=+14−116=316 , on the negative side, sinθ'1=−14−116=−516.
The first principal maximum on the positive side is at distance (above O)
=Dthanθ1=Dsinθ1√1−sin2θ1=D.3/16√1−9/256=3D√162−32
On the negative side, distance of first principal maximum (below O) will be
=Dθ'1=Dsinθ'1√1−sin2θ1=D(−5/16)√1−(5/16)2=−5D√162−52
Topper's Solved these Questions
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Very short answer question
5 Videos
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - very short answer questions
1 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)
2 Videos
Similar Questions
A small transparent slab containing material of μ=1.5 is placed along AS2(figure). What will be the distance from O of the principle maxima and of the first minima on either side of the principal maxima obtained in the absence of the glass slab ?
View SolutionMonochromatic light of wavelength λ passes through a very narrow slit S and then strikes a screen placed at a distance D = 1m in which there are two parallel slits S1 and S2 as shown. Slit S1 is directly in line with S while S2 is displaced a distance d to one side. The light is detected at point P on a second screen, equidistant from S1 and S2 When either slit S1 or S2 is open equal light intensities I are measured at point P. When both slits are open, the intensity is three times as large i.e. 3I. If the minimum possible wavelength is Nd2, then find the value of N(d<<D)

View Solution
Knowledge Check
A small transparent slab (μ=1.5) is placed along AS2 as shown.

AC=CO=D,S1C=S2S=d<<D
The distance of first minima above O isA3D√43BD√247C3D√247D5D√247A small transparent slab (μ=1.5) is placed along AS2 as shown.

AC=CO=D,S1C=S2S=d<<D
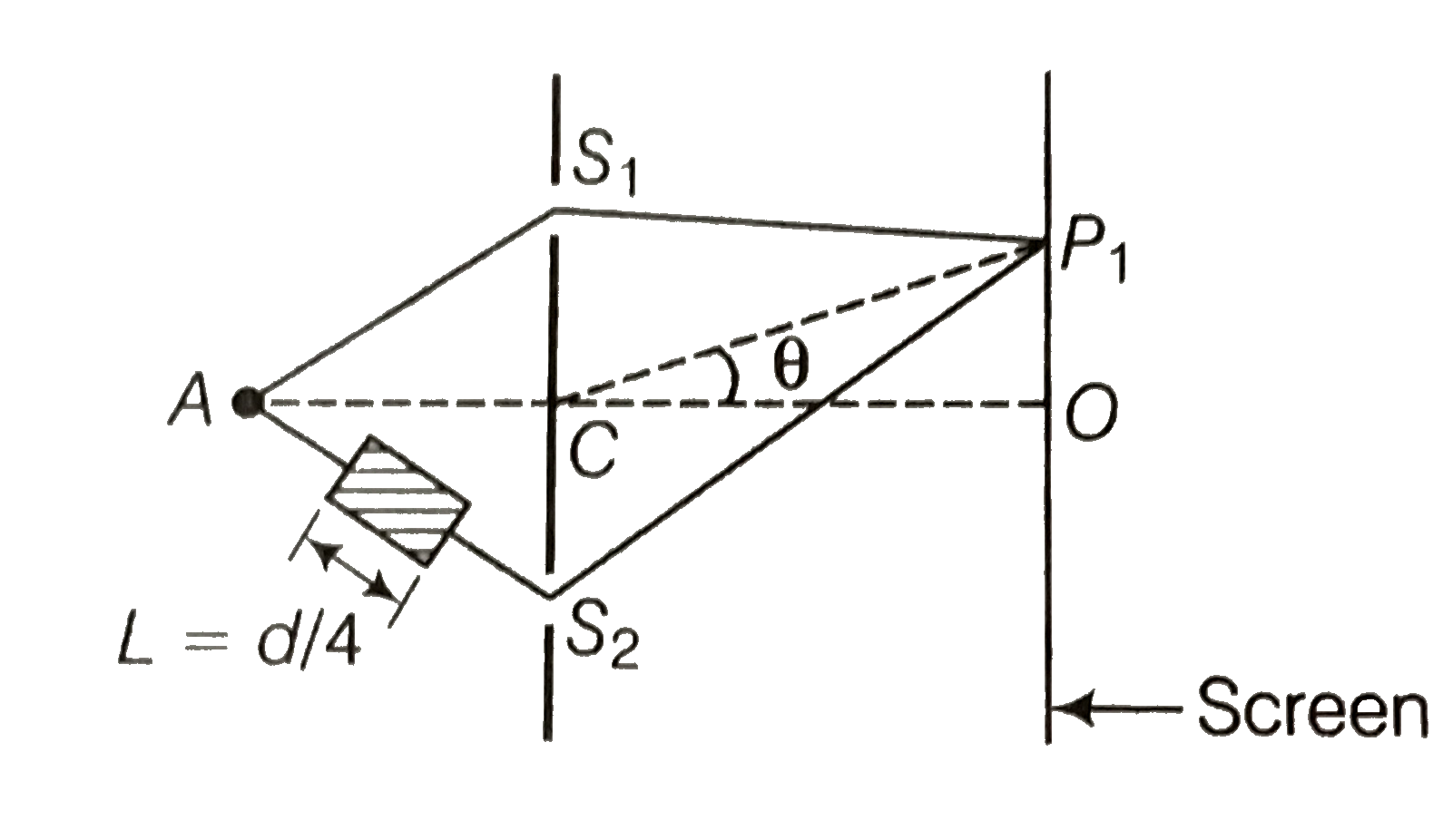
The distance of first minima below the point O isAD√231B3D√231C2D√231D5D√231Young's double slit experiment is conducted in a liquid of refractive index μ1 as shown in figure. A thin transparent slab of refractive index μ2 is placed in front of the slit s2. The magnitude of optical path difference at 'O' is
 A∣∣∣(μ2μ1−1)t∣∣∣B∣∣∣(μ1μ2−1)t∣∣∣C|(μ2−μ1)t|D|(μ2−1)t|
A∣∣∣(μ2μ1−1)t∣∣∣B∣∣∣(μ1μ2−1)t∣∣∣C|(μ2−μ1)t|D|(μ2−1)t|
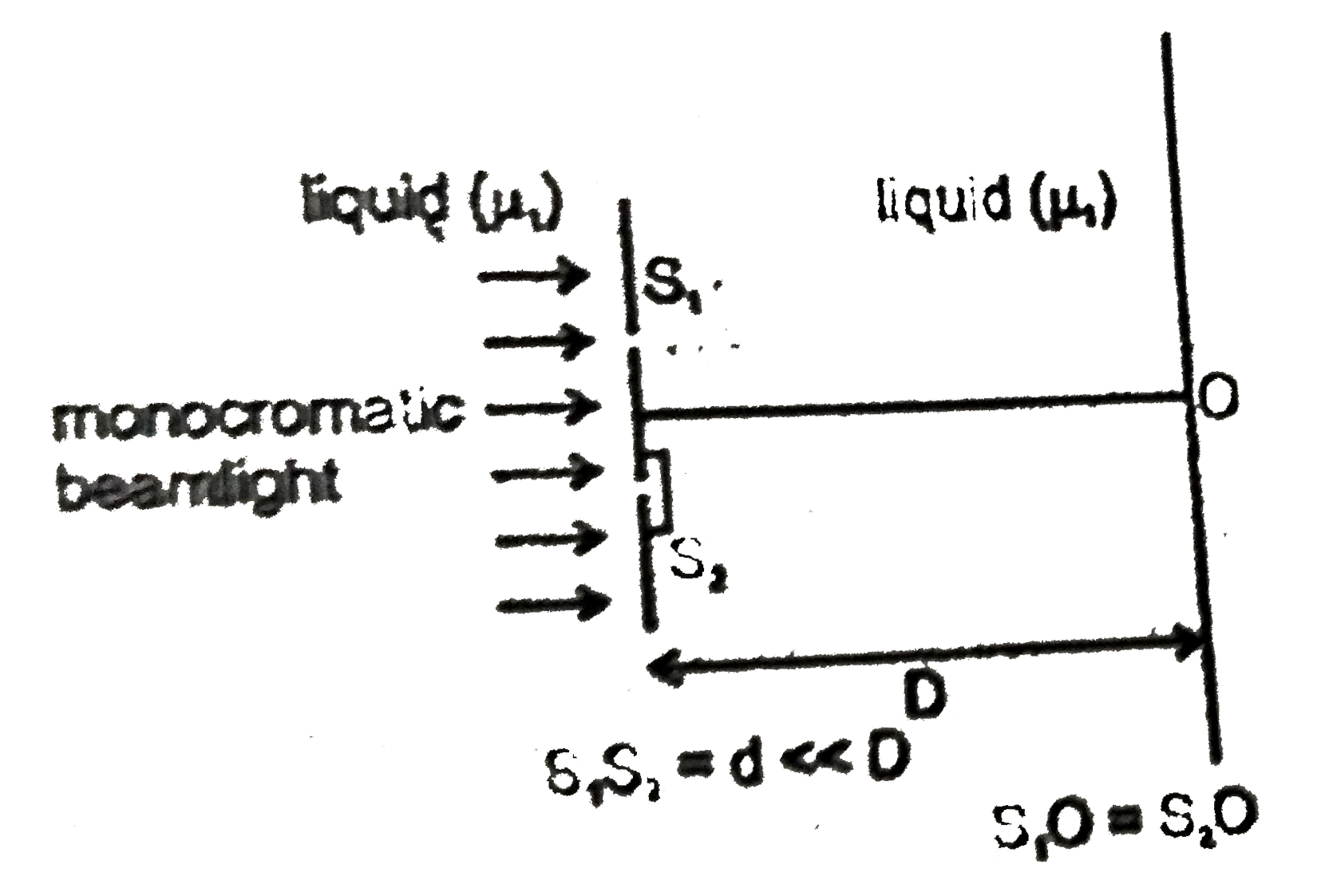
A Young's double slit apparatus is immersed in a liquid of refractive index μ1. The slit plame touches the liquid surface. A parallel beam of monochromatic light of wavelength λ (in air) is incident normally on the slits.
a. Find the fringe width.
b. If one of the slits (say S2) is covered by a transparent slab of refrative index μ2 and thickless t as shown, find the new position of central maxima.
c. Now, the other slit, S1 is also covered by a slab of same thickness and refractive index μ3 as shown in Fig. 2.49 due to which the central maxima recovers it positon, find the value of μ3
Find the ratio of intensities at O in the three conditions (a), (b), and (c).
View SolutionIn a young’s double slit experiment the distance between slits S1 and S2 is d and distance of slit plane from the screen is D(>>d). The point source of light (S) is placed a distance d2 below the principal axis in the focal plane of the convex lens (L). The slits S1 and S2 are located symmetrically with respect to the principal axis of the lens. Focal length of the lens is f(>>d).
Find the distance of the central maxima of the fringe pattern from the centre (O) of the screen.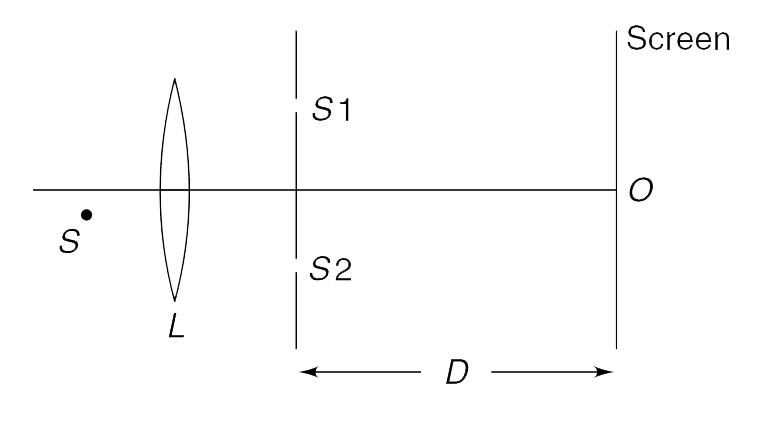
View SolutionMicrowave ocillators S1andS2 are used to show interference of electromagnetic waves on a screen which in parallel to S1S2 and 2 m away from S1S2 . If S1S2=20 cm and λ=4cm, then the distance between the successive maxima and minima will be
View SolutionIn YDSE arangement shown in figure, fringes are seen on screen using monochromatic source S having wavelength 3000∘Δ (in air). S1 and S2 are two slits separate by d=1mm and D=1m . Left of slits S1andS2 medium of refractive index μ1=2 is presernt refractive index μ2=32 is present. A thin slab of thickness 't' is placed in front of S1 . The refractive index of slab μ3 varies with distance from its starting face as shown in figure.

If the thickness of the slab is selected 1μm , then the position of the central maxima will be (y - coordinate)
View SolutionTwo ideal slits S1 and S2 are at a distance d apart, and illuninated by light of wavelength λ passing through an ideal source slit S placed on the line through S2 as shown. The distance between the planes of slits and the source slit is D.A screen is held at a distance D from the plane of the slits. The minimum value of d for which there is darkness at O is (d<<D)
View Solution
