A cube of mass m and densituy D is suspended from the point P by a spring of stiffness k,
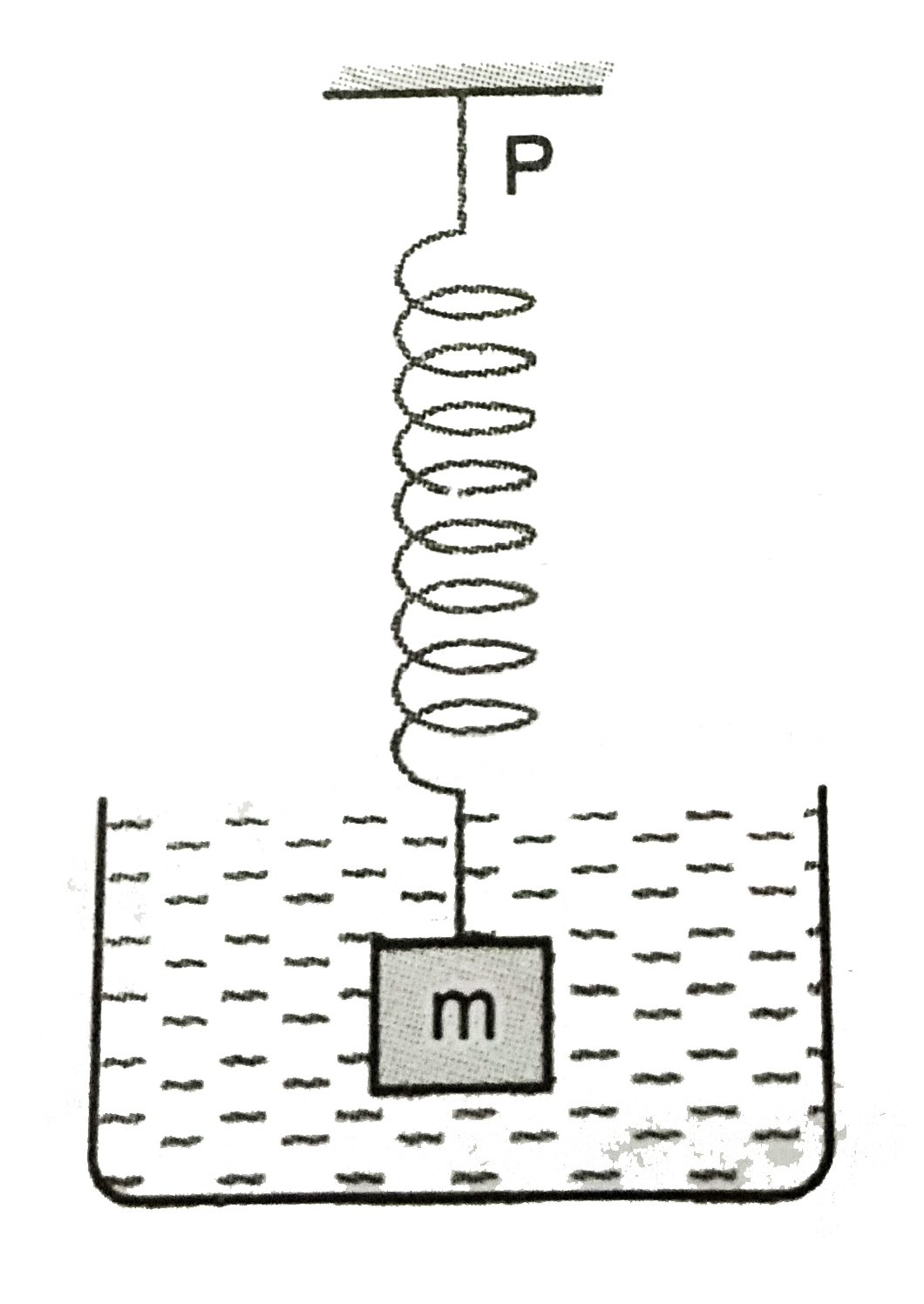
The system is kept inside a beaker filled with a liquid of density d, where D gt d. What is the elongation in the spring ?
The correct Answer is:mgk(1−dD)
Refer to
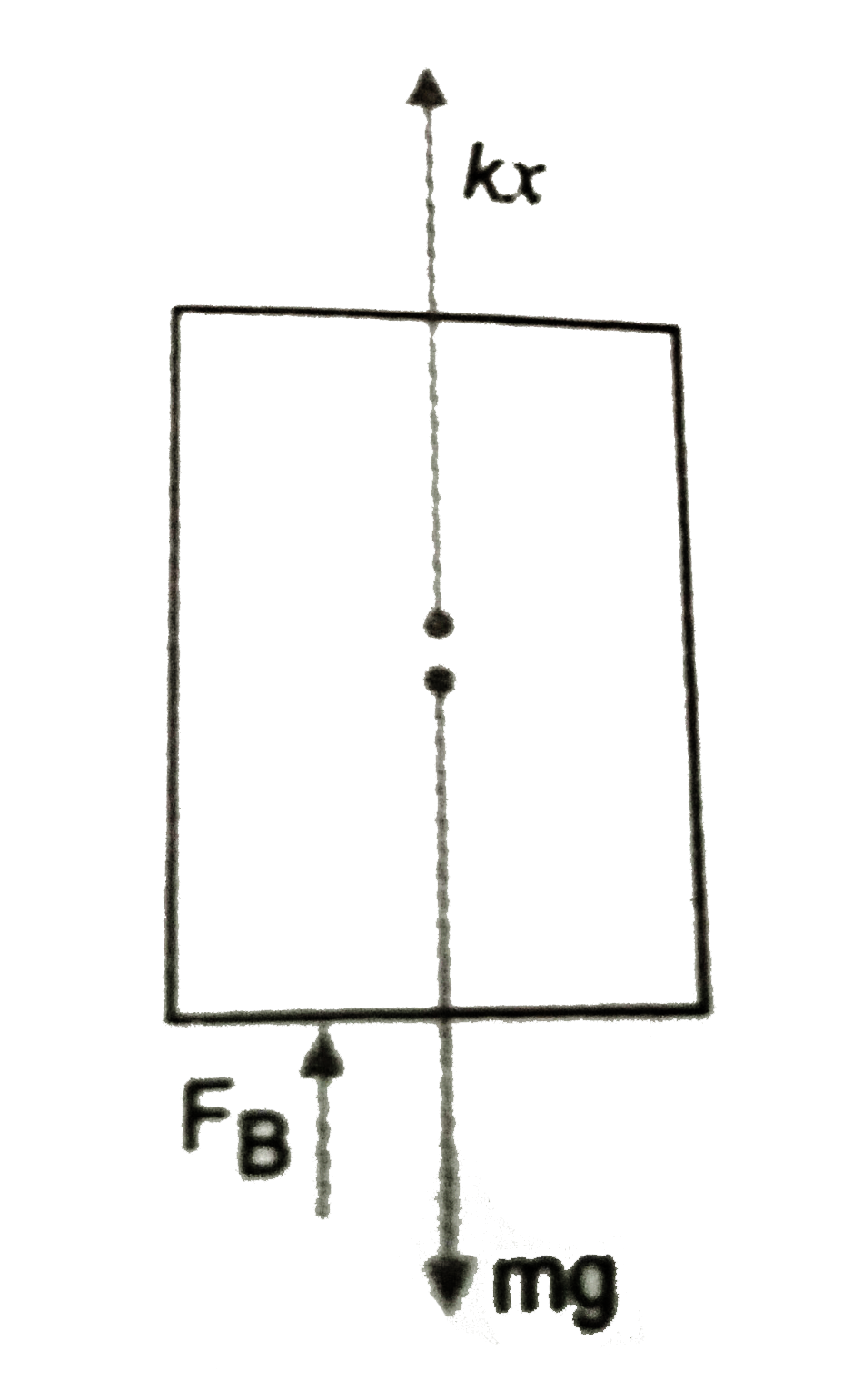
the cube is in equilibrium undeer the effect of following three forces (i) Spring force = kx, acting upwards, where x is the extesnion of the spring. (ii) Gravitational force = mg, acting downwards. (iii) Bouyent force FB = upward thrust on cube due to liquid = Vdg Where V is the volume of the cube completely immersed in liquid. Therefore, for equilibrium of the cube kx+FB=mg
orx=mg−FBk=mg−Vdgk
=mg−(m/D)dgk=mgk[(1−dD)]
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Multiple choice questions-II
Explore 14 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Multiple choice questions-I
Explore 173 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Fill in the Blanks E
Explore 10 Videos
- PHYSICAL WORLD AND MEASUREMENTBook:PRADEEPChapter:PHYSICAL WORLD AND MEASUREMENTExercise:Competiton Focus Jee Medical Entrance
Explore 18 Videos
- RAY OPTICSBook:PRADEEPChapter:RAY OPTICSExercise:Problem For Practice(a)
Explore 25 Videos
Similar Questions
A vessel filled with water is kept on a weighing pan and the scale adjusted of zero. A block of mass M and density ρ is suspended by a masselss spring of spring constant k. This block is submerged inside into the water in the vessel. What is the reading of the scale /
View SolutionA block of mass m is suddenly released from the top of a spring of stiffness constant k.
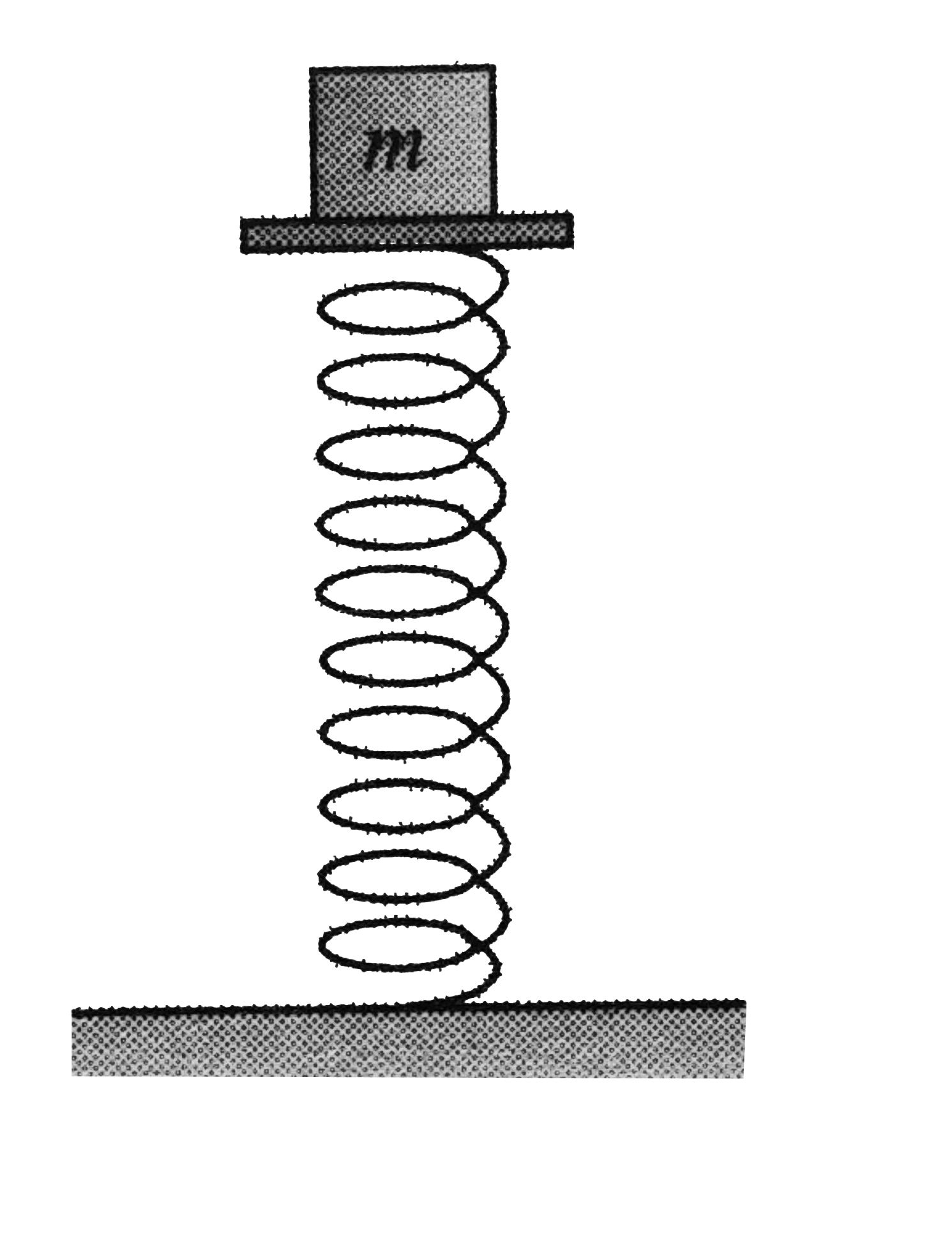
a. Find the acceleration of block just after release.
b. What is the compression in the spring at equilibrium.
c. What is the maximum compression in the spring.
d. Find the acceleration of block at maximum compression in the spring.
View Solution
Knowledge Check
A cube of mass m and density D is suspended from a point P by a spring of stiffness k. The system is kept inside a beaker filled with a liquid of density d. The elongation in the spring, assuming D>d, is :
 Amgk(1−dD)Bmgk(1−Dd)Cmgk(1+dD)DNone of these
Amgk(1−dD)Bmgk(1−Dd)Cmgk(1+dD)DNone of theseA disc of mass m is connected with an ideal spring of stiffness k. If it is released from rest, it rolls without sliding on an inclination plane. The maximum elongation of the spring is:
 AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθk
AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθkA uniform cylinder of length L and mass M having cross-sectional area A is suspended, with its length vertical, from a fixed point by a massless spring such that it is half submerged in a liquid of density σ at equilibrium position. The extension x0 of the spring when it is in equlibrium is:
A(a) MgkB(b) Mgk(1−LAσM)C(c) Mgk(1−LAσ2M)D(d) Mgk(1+LAσM)
A spring elongates by 2 cm when a piece of cobalt of mass 20g is suspended from it. A water conatiner is placed below the pieces in such a way that the piece is immersed completely in water. Calculate the elongation produced in the spring.
Density of cobalt =8700kg/m3,Useg=10ms2.
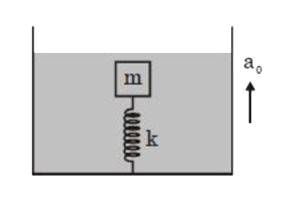
View SolutionA block of mass m and relative density y(<1) si attached to an ideal spring of constant K. The system is initially at rest and at equilibrium. If the container acceleration upwards with a0, find the increase in the elongation of the spring in equilibrium.
View SolutionA body of mass m hangs by an inextensible string that passes over a smooth mass less pulley that is fitted with a light spring of stiffness k as shown in figure. If the body is released from rest and the spring is released, calculate the maximum elongation of the spring.
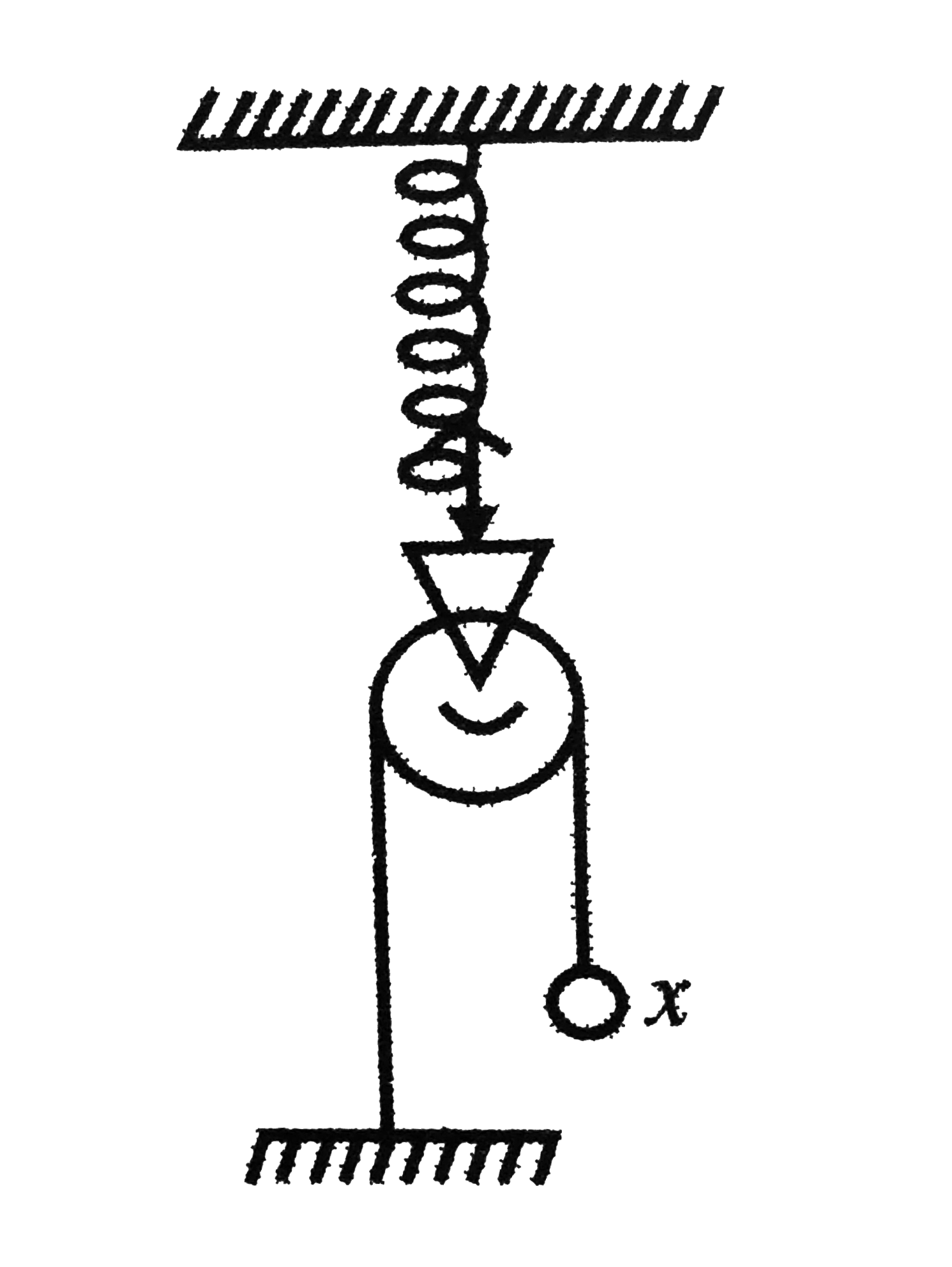
View Solution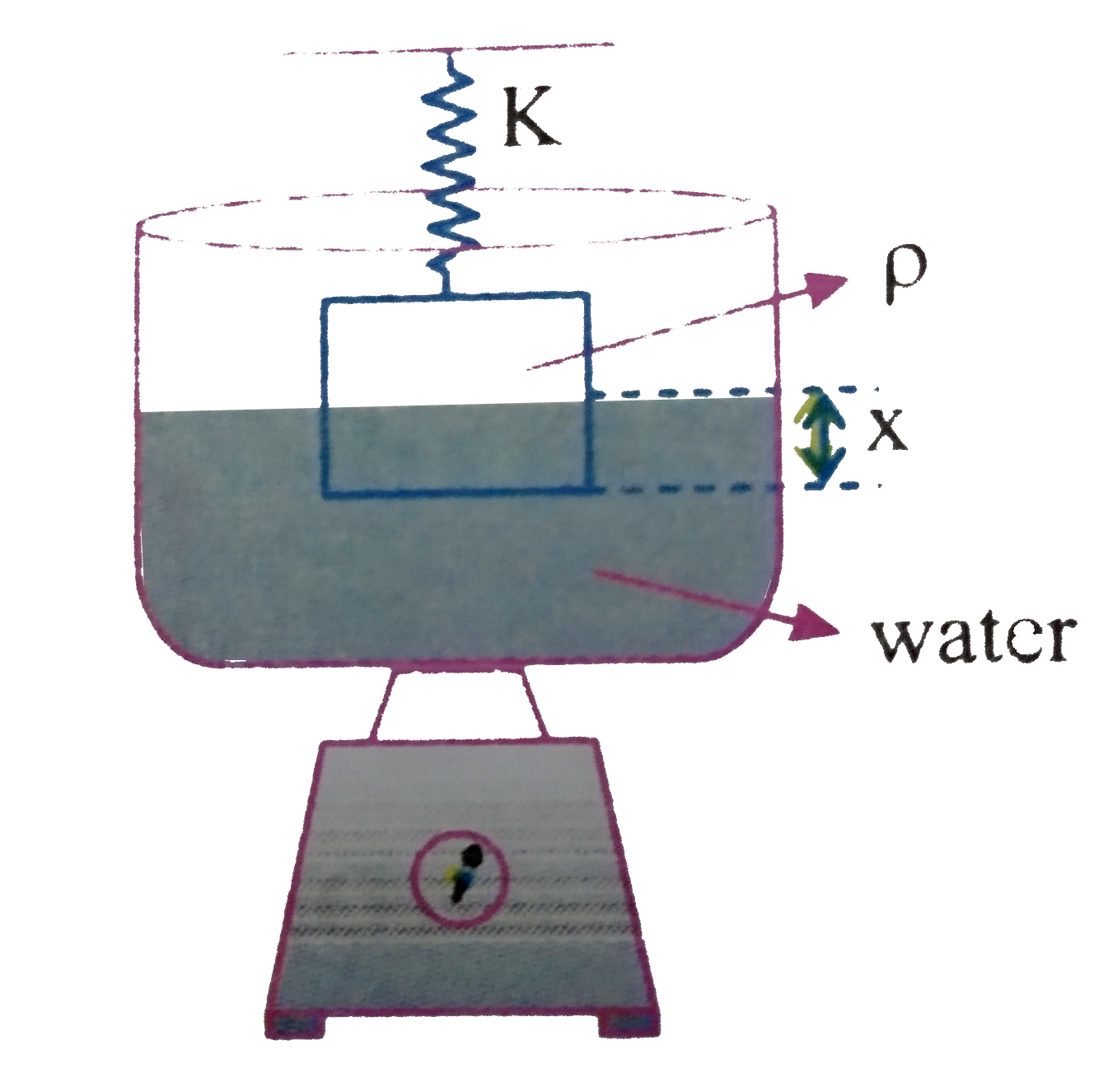
A vessel filled with water is kept on a weighing pan and the scale adjusted to zero. A block of mass 10 kg is suspended by a massless spring of spring constant k=100N/m. This block is submerged inside into the water in the vessel such that elongation i spring is x=10cm. What is the reading of the scale?
View SolutionTwo thin films of same liquid of surface tension 'T' are formed between a smooth rectangular wire frame and two thin uniform strainght wires each of mass 'm' and length 'I' connected to a massless non-deformed spring of stiffness 'k' and then the system is released form rest. The maximum elongation produced in the spring is
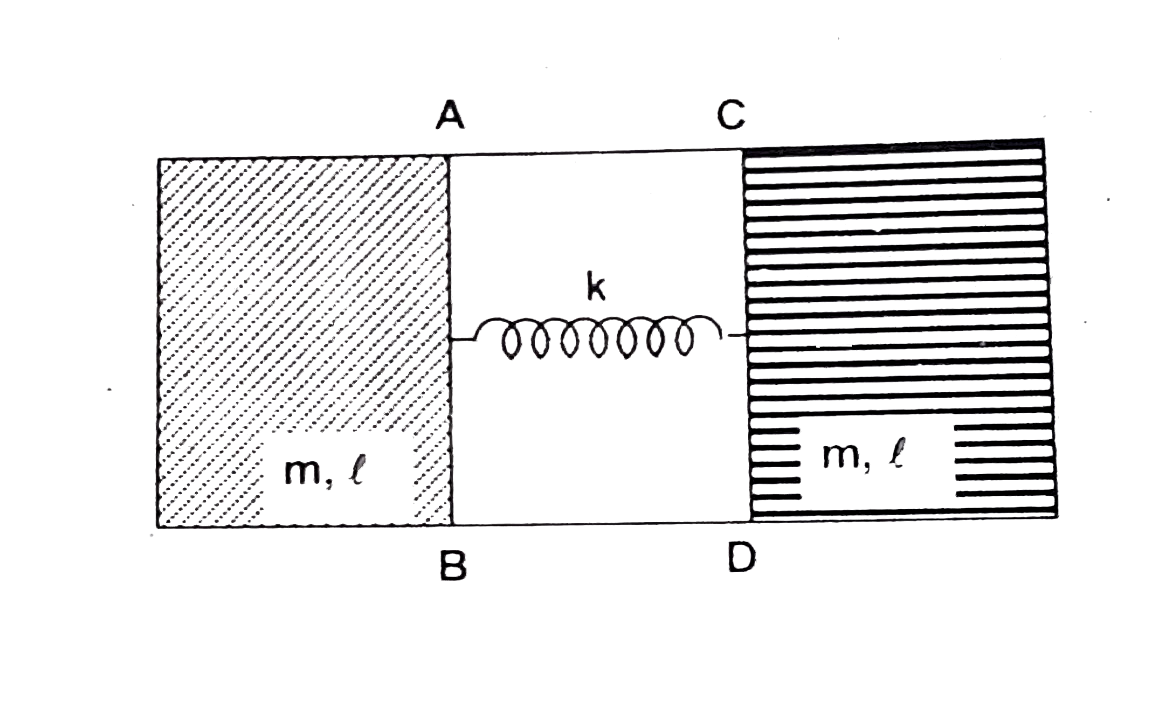
View Solution