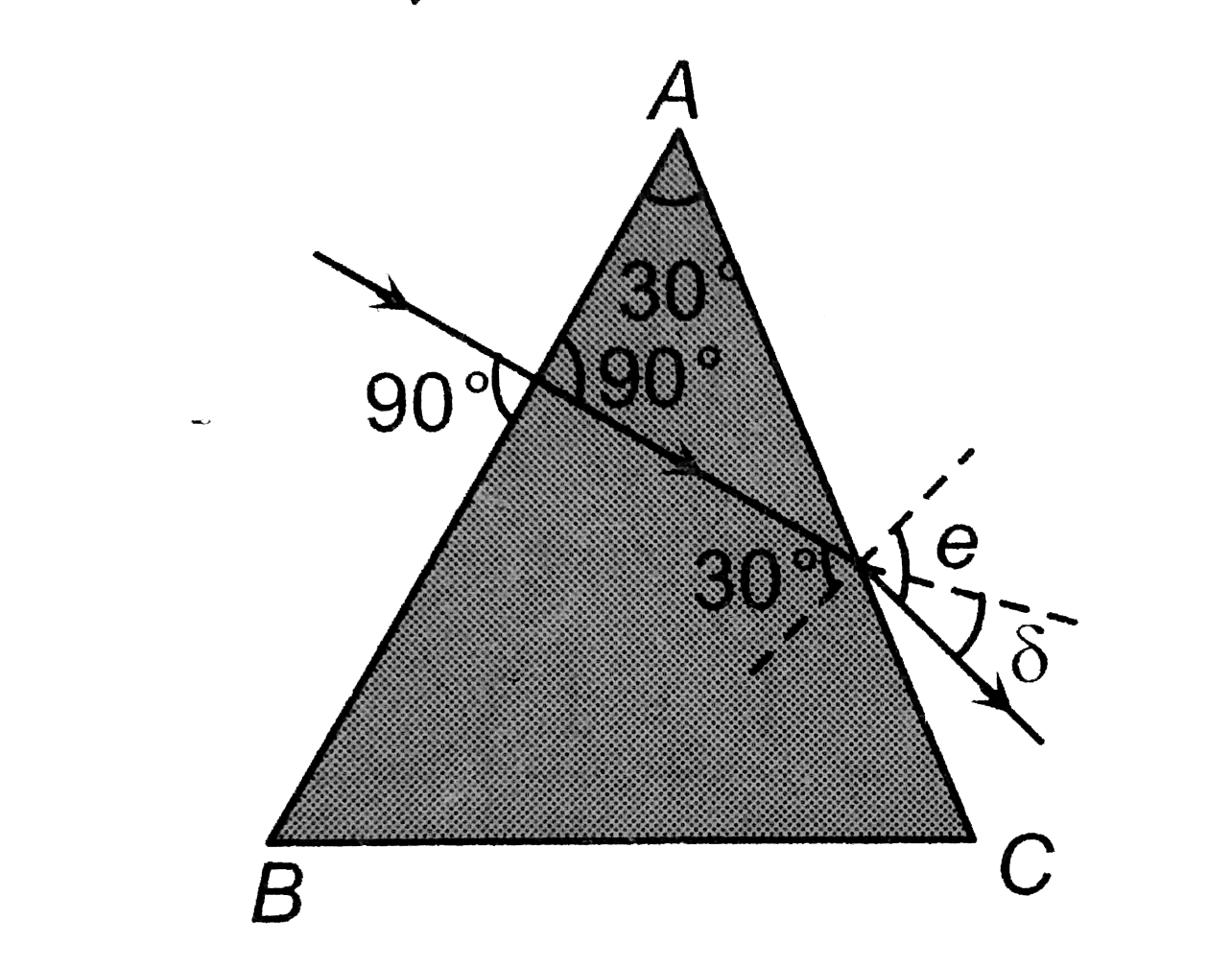
The refracting angle of a glass prism is 30∘. A ray is incident onto one of the faces perpendicular to it. Find the angle δ between the incident ray and the ray that leaves the prism. The refractive index of glass is μ=1.5.
18∘36'
20∘30∘'
18∘
22∘1'
The correct Answer is:A
For surface AC1μsin30∘sine⇒sine=μsin30∘
⇒sine=1.5×12=0.75
⇒e=sin−1(0.75)=48∘36'
From figure δ=e−30∘
=48∘36'−30=18∘36'
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - A2ZCHAPTER - GEOMETRICAL OPTICSEXERCISE - Optical Instruments
55 Videos
- GEOMETRICAL OPTICSBOOK - A2ZCHAPTER - GEOMETRICAL OPTICSEXERCISE - Problems Based On Mixed Concepts
37 Videos
- GEOMETRICAL OPTICSBOOK - A2ZCHAPTER - GEOMETRICAL OPTICSEXERCISE - Refraction At Curved Surface
64 Videos
- ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM BOOK - A2ZCHAPTER - ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM EXERCISE - Section D - Chapter End Test
30 Videos
- MAGNETISM AND MATTERBOOK - A2ZCHAPTER - MAGNETISM AND MATTEREXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
The cross section of a glass prism μ=2 has the form of an isosceles right-angled triangle. It is submerged in a liquid of refractive index 1.2. A ray is incident on to one of the equal faces perpendicularly. What is the angle between the direction of incident ray and the ray that emerges from the prism ?
View SolutionThe angle between the incident ray and the emergent ray in a glass prism is called (angle of incidence/angle of deviation).
View Solution
Knowledge Check
An equilateral triangular prism is made of glass (μ=1.5). A ray of light is incident normally on one of the faces. The angle between the incident and emergent ray is
A60∘B90∘C120∘D180∘Light is incident on a glass surface at polarising angle of 57.5∘ Then the angle between the incident ray and the refracted ray is
A57.5∘B115∘C65∘D205∘The angle of a prism is 30∘. The rays incident at 60∘ on one refracting face suffer a deviation of 30∘. Then the angle of emergence is :
A0∘B30∘C60∘D90∘
The refracting angle of a glass prism is 60∘ and μ of its material is 1.45. Calculate angle of incidence at the first that will just reflect internaly the ray at the second face.
View SolutionA light ray is travelling from air to glass. True reflected and refracted rays are perpendicular to each other. If the angle of incidence in air is I the refractive index of glass is
View SolutionA ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
View SolutionA ray of light is incident at 65∘ on one face of a prism of angle of 30∘ and the emergent ray makes 35∘ with the incident ray. The refractive index of the prism is:
View SolutionA ray of light is incident normally on one of the equal faces of a right isosceles prism of refractive index 1.5. The angle between incident and emergent rays is
View Solution
