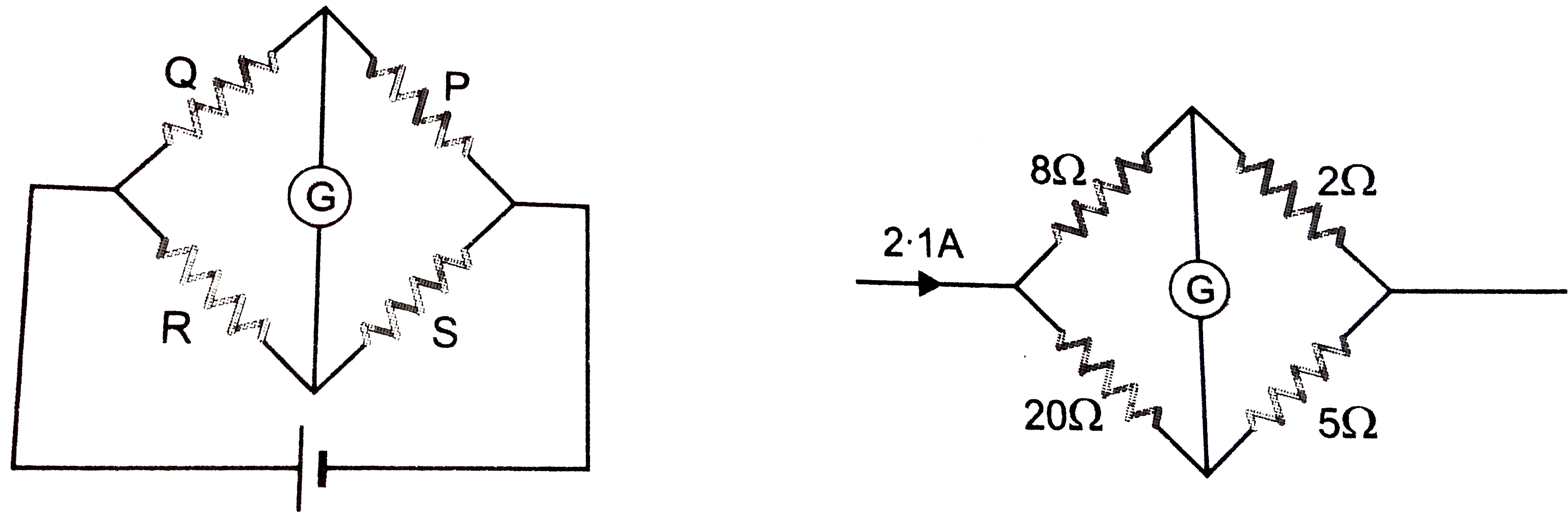
Three resistance each of and an unknown resistance from the four amrs of a Wheatstone's bridge circuit. When a resistance of is connected in parallel to the bridge gets balanced. What is the value of ?
The correct Answer is:B
To find the unknown resistance S in the Wheatstone bridge circuit, we can follow these steps:
Step 1: Understand the Wheatstone Bridge Configuration
In a Wheatstone bridge, we have four resistances arranged in a diamond shape. The resistances are P,Q,R, and S. In this case, P=2Ω, Q=2Ω, and R=2Ω. The resistance S is unknown.
Step 2: Apply the Balancing Condition
When the bridge is balanced, the following condition holds:
PQ=RSeq
Where Seq is the equivalent resistance of S when a 6Ω resistor is connected in parallel with it.
Step 3: Calculate the Equivalent Resistance Seq
The equivalent resistance Seq of S and 6Ω in parallel is given by:
Seq=S⋅6S+6
Step 4: Substitute Known Values into the Balancing Condition
Substituting the known values into the balancing condition:
22=2Seq
This simplifies to:
1=2Seq
Thus, we have:
Seq=2Ω
Step 5: Set Up the Equation
Now, we can set up the equation using the expression for Seq:
S⋅6S+6=2
Step 6: Cross Multiply to Solve for S
Cross multiplying gives:
S⋅6=2(S+6)
Expanding the right side:
6S=2S+12
Step 7: Rearrange the Equation
Rearranging the equation:
6S−2S=12
This simplifies to:
4S=12
Step 8: Solve for S
Dividing both sides by 4:
S=3Ω
Conclusion
The value of the unknown resistance S is 3Ω.
---
In a Wheatstone bridge, we have four resistances arranged in a diamond shape. The resistances are P,Q,R, and S. In this case, P=2Ω, Q=2Ω, and R=2Ω. The resistance S is unknown.
When the bridge is balanced, the following condition holds:
PQ=RSeq
Where Seq is the equivalent resistance of S when a 6Ω resistor is connected in parallel with it.
The equivalent resistance Seq of S and 6Ω in parallel is given by:
Seq=S⋅6S+6
Substituting the known values into the balancing condition:
22=2Seq
This simplifies to:
1=2Seq
Thus, we have:
Seq=2Ω
Now, we can set up the equation using the expression for Seq:
S⋅6S+6=2
Cross multiplying gives:
S⋅6=2(S+6)
Expanding the right side:
6S=2S+12
Rearranging the equation:
6S−2S=12
This simplifies to:
4S=12
Dividing both sides by 4:
S=3Ω
The value of the unknown resistance S is 3Ω.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:A2ZChapter:CURRENT ELECTRICITYExercise:Section - C
Explore 1 Video
- CURRENT ELECTRICITYBook:A2ZChapter:CURRENT ELECTRICITYExercise:AIIMS Questions
Explore 36 Videos
- CURRENT ELECTRICITYBook:A2ZChapter:CURRENT ELECTRICITYExercise:Section B - Assertion Reasoning
Explore 28 Videos
- ATOMIC PHYSICSBook:A2ZChapter:ATOMIC PHYSICSExercise:Section D - Chapter End Test
Explore 30 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:A2ZChapter:DUAL NATURE OF RADIATION AND MATTERExercise:Section D - Chapter End Test
Explore 30 Videos
Similar Questions
Four resistances of 16Ω,12Ω,4Ωand9Ω respectively are connected in cycle order so from a Wheatstone bridge. Calculate the resistance in be connected in parallel with 9Ω resistance to balance the bridge.
View SolutionP,QRandS four resistance wires of resistance 1,2,3and4 ohm respectivily. They are connected in form the four arms of Wheatstone bridge circuit. Find out the resistance with which S meat be shunted in order the bridge may be balanced
View Solution
Knowledge Check
Three resistances P, Q, R each of 2 12 and an unknown resistance S form the four arms of a Wheatstone bridge circuit. When a resistance of 6Ω is connected in parallel to S, the bridge gets balanced. What is the value of S?
A3ΩB6ΩC1ΩD2ΩThree resistances P,Q,R each of 2Ω and an unknown resistance S form the four arms of a Wheatstone bridge circuit. When a resistance of 6Ω is connected in parallel to S the bridge gets balanced. What is the value of S ?
A2ΩB3ΩC1ΩD1ΩThree resistances P,Q,R each of 2Ω and an unknown resistance S form the four arms of a Wheatstone bridge circuit. When a resistance of 6Ω is connected in parallel to S the bridge gets balanced. What is the value of S ?
A2ΩB3ΩC1ΩD1Ω
A 6Ω resistance is connected in the left gap of a meter bridge.In the second gap 3Ω and 6Ω are joined in parallel.The balance point of the bridge is at
View SolutionIn a metre bridge the null point is found at a distance of 35 cm from A. If now a resistance of 10Ω is connected in parallel with S, the null point occurs at 50 cm. Determine the values of R and S.
View SolutionA, B, C and D are four resistance of 2Ω, 2Ω, 2Ω and 3Ω respectivey. They are used to form a wheatstone bridge. The resistance D is short circuited with a resistance R in order to get the bridge balanced. The value of R will be
View SolutionIn a Wheatstone bridge resistance connected the bridge is balanced , when the resistance are in the ratio
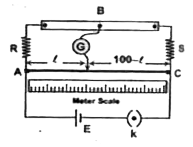
View SolutionIn a meter bridge, the null point is found at a distance of 40 cm from A. If now a resistance of 12 Ω is connected in parallel with S, the null point occurs at 60 cm from A. Determine the value of R.
View Solution
