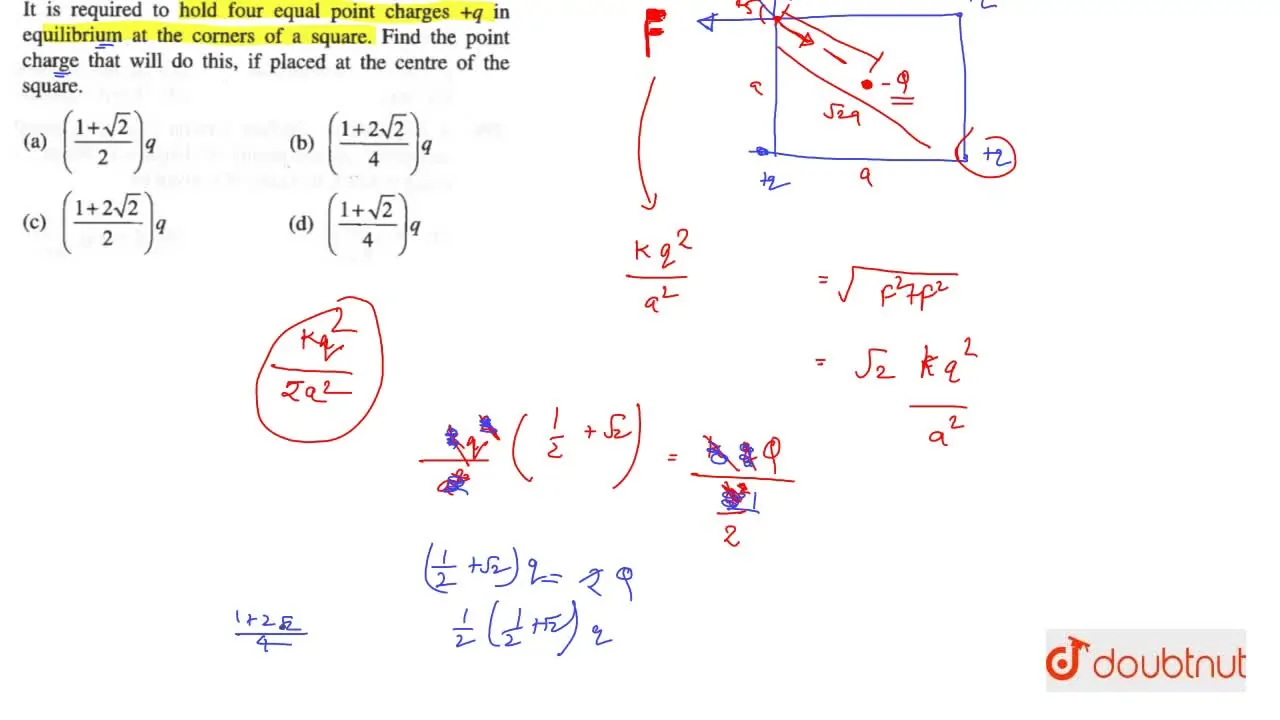
It is requird to hold four equal point charges +q in equilibrium at the corners of a square. Find the point charge that will do this, if placed at the centre of the square.
(1+√22)q
(1+2√24)q
(1+2√22)q
(1+√24)q
The correct Answer is:b
Suppose that the charge Q placed at the centre of the square keeps all the four charges in equilibrium. It follows that the charge Q has to be negative in nature.
If the net force on a charge at any corner (sayA) is zero, then by, symmetry it follows that the net force experienced by the charge at other corners will also be zero. The charge at corner A will experience force FB,FC and FD due to the charges at the centre of he square.
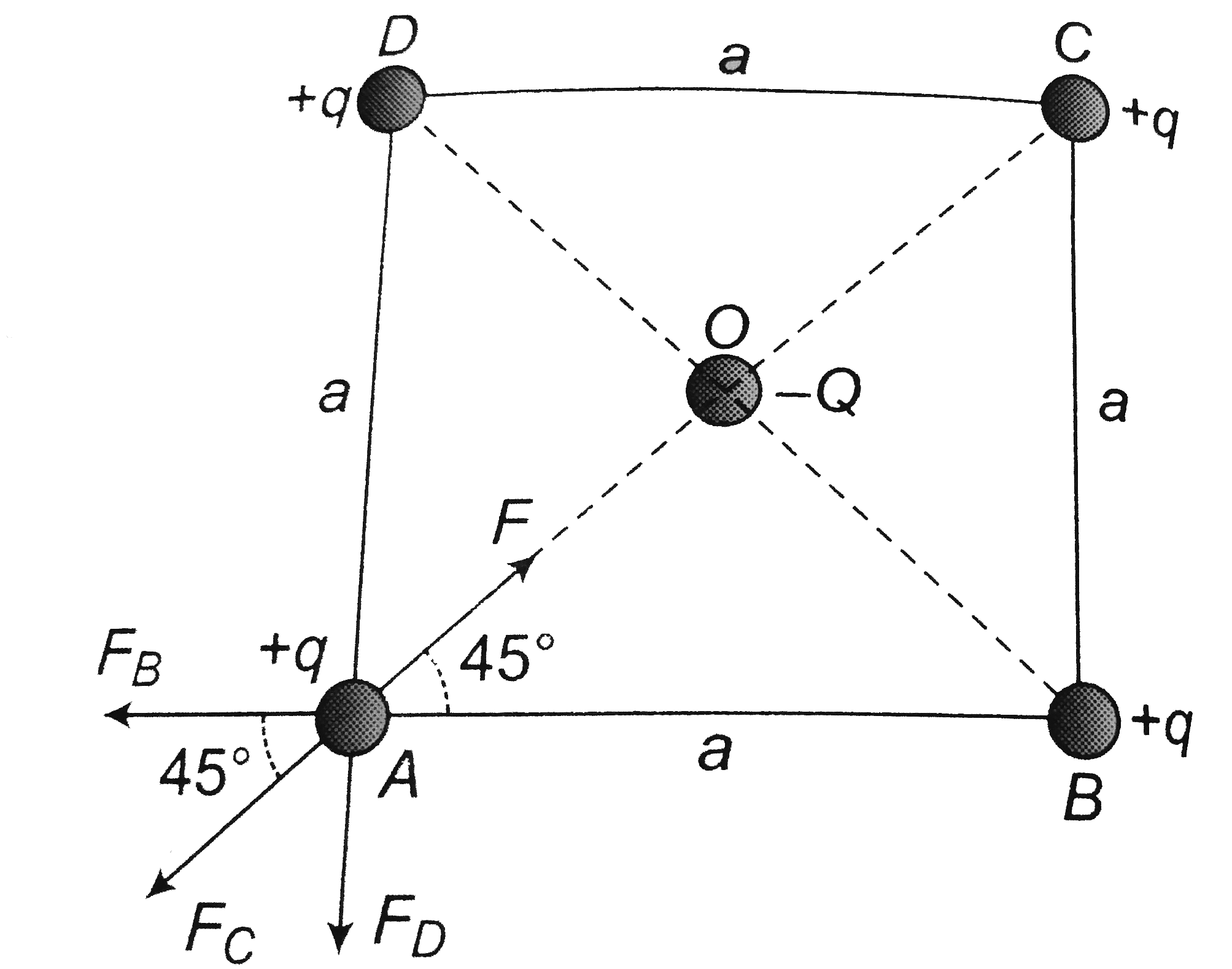
If each side of the square is of length a, then
AC=√a2+a2=√2a
and OA=12AC=a√2
Now, FB=FD=14πε0.q2a2
FC=14πε0.Qq2(√2a)2=14πε0.q22a2
and F=14πε0.Qq2(a/√2)2=14πε0.2Qqa2
For net force on the charges at A to be zero,
FB+FCcos45∘=Fcos45∘
and FD+FCsin45∘=Fsin45∘
Substituting for FB,FC and F in the equation (i), we get
Q=(1+2√24)q (negative)
Related Playlists
- BOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Section B - Assertion Reasoning25videos
ELECTRIC CHARGE, FIELD & FLUX
- BOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - AIPMTNEET Questions24videos
ELECTRIC CHARGE, FIELD & FLUX
- BOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Electric Dipole29videos
ELECTRIC CHARGE, FIELD & FLUX
- BOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section D - Chapter End Test30videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Section D - Chapter End Test29videos
ELECTRIC POTENTIAL & CAPACITANCE
Similar Questions
IT si required to hold four equal point charges each having a charge Q=87(1−2√2)C in equilibrium at the corners of a square.Find the point charge in coulombs that will do this if placed at the centre of the square.
View SolutionFour equal point charges, each of magnitude +Q, are to be placed in equilibrium at the corners of a square. What should be the magnitude and sign of the point charge that should be placed at the center of the square to do this job?
View SolutionA point charge Q is placed at the corner of a square of side a. Find the flux through the square.
View SolutionFour point charge are placed at the corners of a square with diagonal 2a as shown. What is the total electric field at the center of the squre?
 View Solution
View SolutionFour charges are arranged at the corners of a square as shown in the figure. The direction of electric field at the centre of the square is along
 View Solution
View SolutionA point charge Q is placed at the corner of a square of side a, then find the flux through the square.
 View Solution
View SolutionFour point charges of +1μc each are placed on the four corners of a square of side 1 m. Calculate the electric potential at the centre of the square.
View SolutionFour point charges are placed at the corners of a square ABCD of side 10 cm, as shown in figure. The force on a charge of 1μC placed at the centre of square is
 View Solution
View SolutionFour charges are arranged at the corners of a square ABCD, as shown. The force on a positive charge kept at the centre of the square is
 View Solution
View SolutionFour identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .View Solution
.View Solution