Two short dipoles pˆk and P2ˆk are located at (0,0,0) & (1m,0,2m) respectively. The resultant electric field due to the two dipoles at the point (1m,0,0) is
9p32πε0ˆk
−7p32πε0ˆk
7p32πε0ˆk
none of these
The correct Answer is:b
To find the resultant electric field at the point (1m, 0, 0) due to the two dipoles located at (0, 0, 0) and (1m, 0, 2m), we will follow these steps:
Step 1: Identify the Dipoles and Their Positions
- The first dipole, →p, is located at point (0,0,0) and has a dipole moment →p=p^k.
- The second dipole, p2, is located at point (1,0,2) and has a dipole moment →p2=p2^k.
Step 2: Calculate the Electric Field Due to the First Dipole at (1, 0, 0)
- The distance r from the first dipole to the point (1, 0, 0) is:
r1=√(1−0)2+(0−0)2+(0−0)2=1 m
- The electric field →E1 due to a dipole at the equatorial position is given by:
→E1=k⋅→pr3
Since we are at the equatorial position, the direction of the electric field will be opposite to the dipole moment:
→E1=−k⋅p13^k=−kp^k
Step 3: Calculate the Electric Field Due to the Second Dipole at (1, 0, 0)
- The distance r from the second dipole (1, 0, 2) to the point (1, 0, 0) is:
r2=√(1−1)2+(0−0)2+(0−2)2=2 m
- The electric field →E2 due to the second dipole at the axial position is given by:
→E2=2k⋅→p2r3
Here, →p2=p2^k:
→E2=2k⋅(p2)23^k=kp4^k
Step 4: Combine the Electric Fields
- The resultant electric field →E at point (1, 0, 0) is the vector sum of →E1 and →E2:
→E=→E1+→E2=−kp^k+kp4^k
- Simplifying this gives:
→E=(−kp+kp4)^k=(−4kp4+kp4)^k=−3kp4^k
Step 5: Express in Terms of ϵ0
- Recall that k=14πϵ0:
→E=−34⋅p4πϵ0^k=−3p16πϵ0^k
Final Result
The resultant electric field at the point (1m, 0, 0) due to the two dipoles is:
→E=−3p16πϵ0^k
- The first dipole, →p, is located at point (0,0,0) and has a dipole moment →p=p^k.
- The second dipole, p2, is located at point (1,0,2) and has a dipole moment →p2=p2^k.
- The distance r from the first dipole to the point (1, 0, 0) is:
r1=√(1−0)2+(0−0)2+(0−0)2=1 m
- The electric field →E1 due to a dipole at the equatorial position is given by:
→E1=k⋅→pr3
Since we are at the equatorial position, the direction of the electric field will be opposite to the dipole moment:
→E1=−k⋅p13^k=−kp^k
- The distance r from the second dipole (1, 0, 2) to the point (1, 0, 0) is:
r2=√(1−1)2+(0−0)2+(0−2)2=2 m
- The electric field →E2 due to the second dipole at the axial position is given by:
→E2=2k⋅→p2r3
Here, →p2=p2^k:
→E2=2k⋅(p2)23^k=kp4^k
- The resultant electric field →E at point (1, 0, 0) is the vector sum of →E1 and →E2:
→E=→E1+→E2=−kp^k+kp4^k
- Simplifying this gives:
→E=(−kp+kp4)^k=(−4kp4+kp4)^k=−3kp4^k
- Recall that k=14πϵ0:
→E=−34⋅p4πϵ0^k=−3p16πϵ0^k
The resultant electric field at the point (1m, 0, 0) due to the two dipoles is:
→E=−3p16πϵ0^k
Topper's Solved these Questions
- ELECTRIC CHARGE, FIELD & FLUXBOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Problems Based On Mixed Concepts
40 Videos
- ELECTRIC CHARGE, FIELD & FLUXBOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Section B - Assertion Reasoning
25 Videos
- ELECTRIC CHARGE, FIELD & FLUXBOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Electric Field And Gauss'S Law
25 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section D - Chapter End Test
30 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Section D - Chapter End Test
29 Videos
Similar Questions
Two point dipoles pˆk and P2ˆk are located at (0, 0, 0) and (1m, 0, 2m) respectively. Find the resultant electric field due to the two dipoles at the point M(1m, 0, 0)
[−(7P32π∈0)ˆk]
View Solution'Two short dipoles pˆk and p2ˆk are located at (0,0,0) and (1(~m),0,2(~m)), respectively. The resultant electric field due to the two dipoles at the point (1,m,0,0) is 4np32πɛ0k. Find n.
View Solution
Knowledge Check
Two short dipoles pˆk and P2ˆk are located at (0,0,0) & (1m,0,2m) respectively. The resultant electric field due to the two dipoles at the point (1m,0,0) is
A9p32πε0ˆkB−7p32πε0ˆkC7p32πε0ˆkDnone of theseTwo short dipoles pˆk and P2ˆk are located at (0,0,0) & (1m,0,2m) respectively. The resultant electric field due to the two dipoles at the point (1m,0,0) is
A9p32π∈0ˆkB−7p32π∈0ˆkC7p32π∈0ˆkDnone of these
[" Two point dipoles "],[phat k" and "(p)/(2)hat k" are located at "(0,0,],[0)" and "(1m,0,2m)" respectively."],[" if the resultant electric field "],[" due to the two dipoles at the "],[" point "(1m,0,0)" is "x(-(7)/(8)kphat k)" ."],[" Where "k" is electro static "],[" constant.Find "x" ? "]
View SolutionTwo fixed charges of +2Cand−2C are kept at (1,0)and(0,−1) respectively. Find the electric field at the point (1,−1).
View SolutionTwo point charges of, 1 μC and -1 μC are kept at (-2 m, 0) and (2 m, 0) respectively. Calculate the electric field at point (0, 5 m).
View Solution4.A electric dipole of dipole moment →p=9ˆi+3ˆj−3ˆk is placed at the point M(2 ,-3 ,1) .If the electric potential due to this dipole at the point B(4 ,-1, 0) is N times 109 volt.Then find N if the given data is in S.l units
View SolutionThe point charges q1=1μC,q2=−2μC and q3=3μC are placed at (1m,0,0),(0,2m,0) and (0,0,3m) respectively. Find the electric potential at origin.
View SolutionAn electric dipole is formed by two charges +q and -q located in xy-plane at (0,2) mm and (0,-2) mm, respectively, as shown in the figure. The electric potential at point P (100,100) mm due to the dipole is V_{0} The charges +q and -q are then moved to the points (-1,2) mm and (1,-2) mm, respectivelyWhat is the value of electric potential at P due to the new dipole?
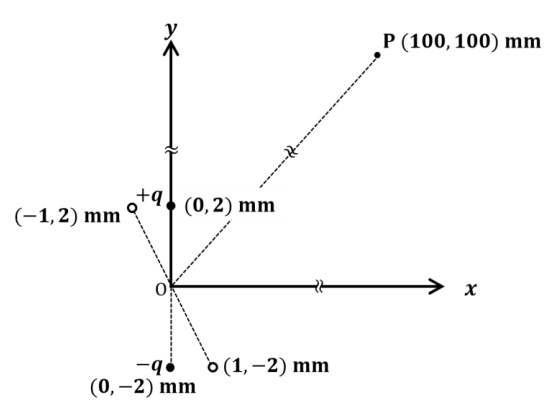
View Solution