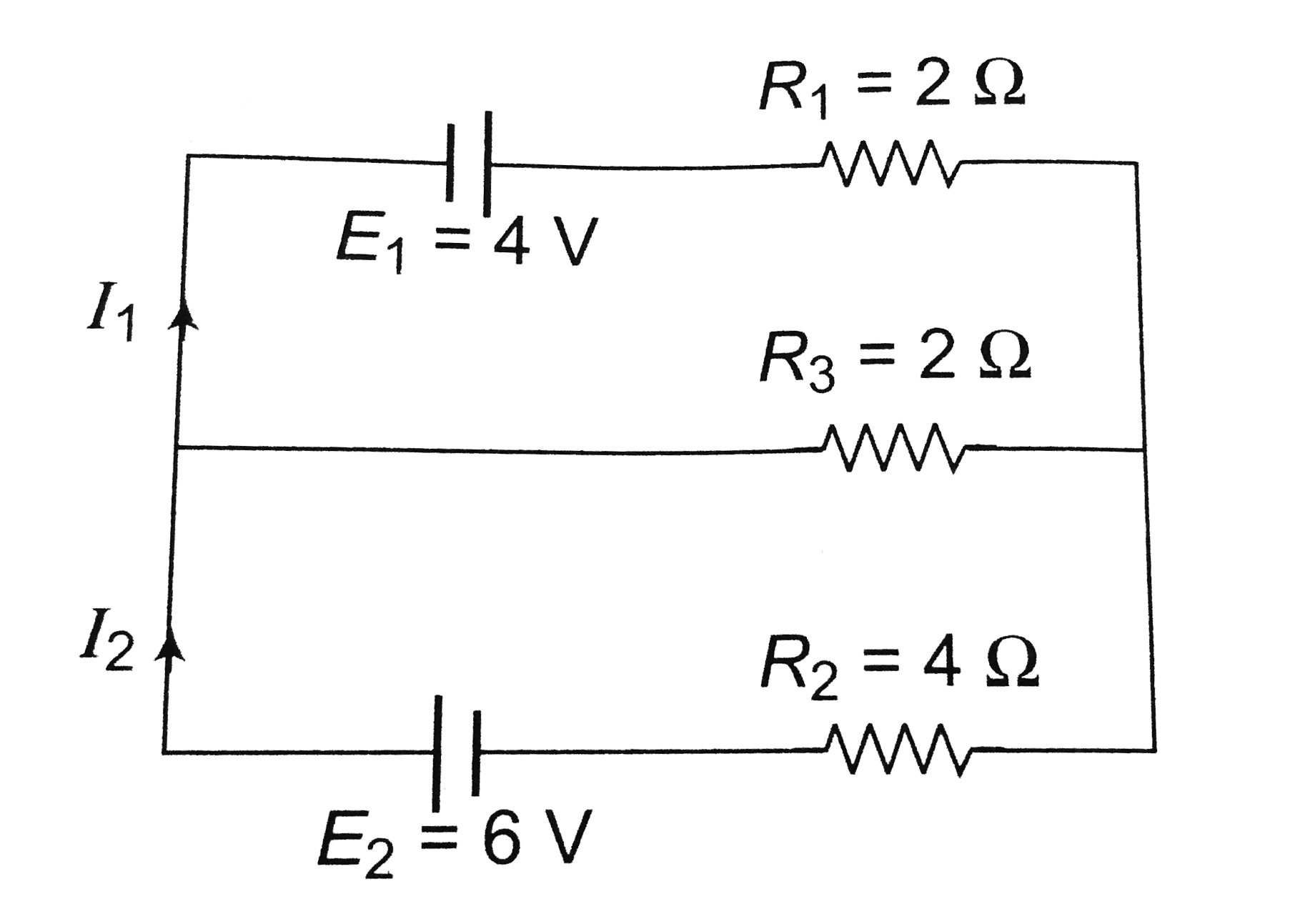
In the circuit shown in figure :
and . Current in circuit is in circuit is and the main current is
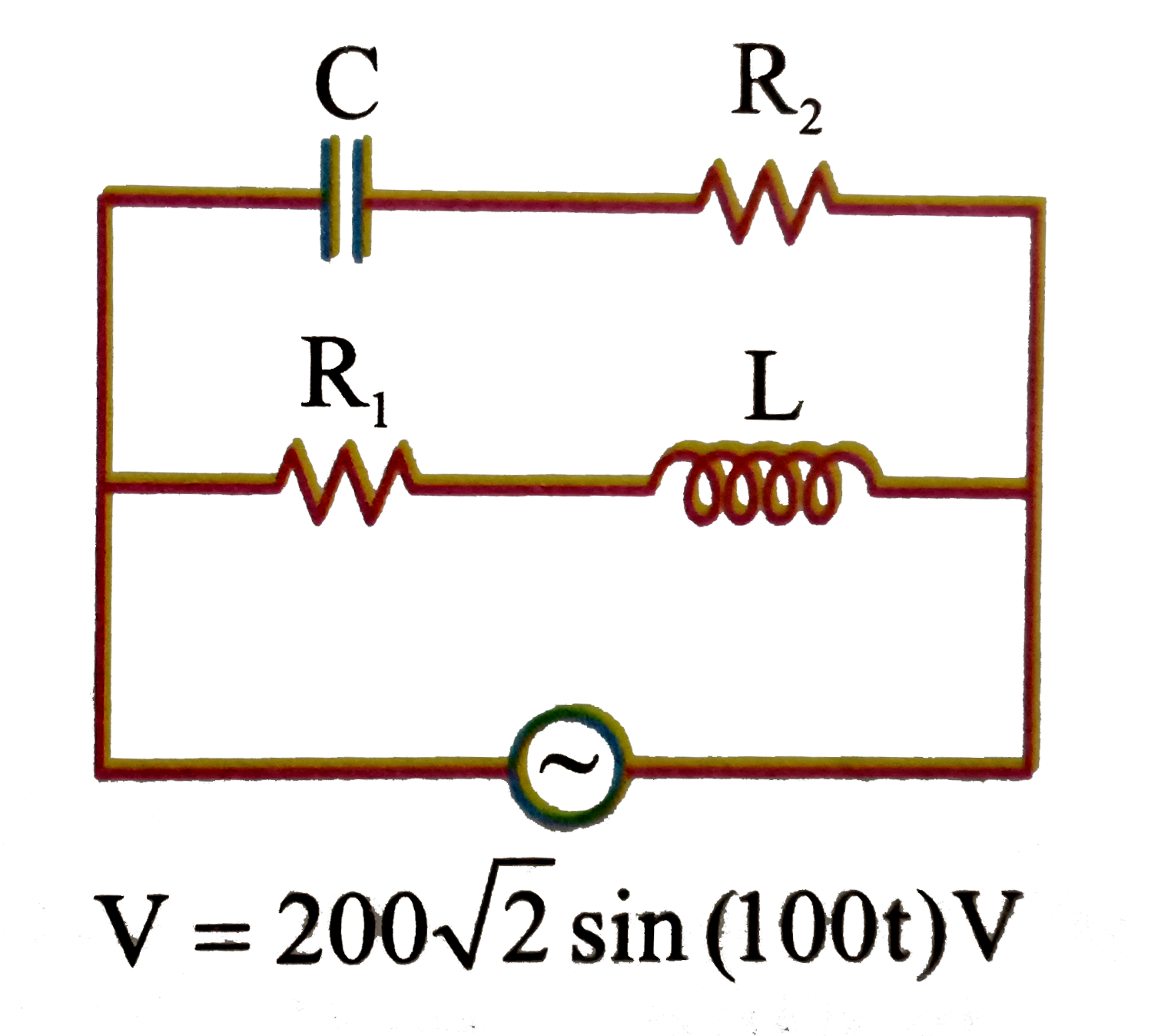
At some instant current in circuit is . At the same instant current in branch will be
Video Solution
Text Solution
For -L branch
or
Hence current lags voltage by For branch
or
Hence current leads voltage by
The phase difference between and branch is
The maximum current through branch is
.
Hence when current through branch is amp. the current through branch will be zero.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the circuit shown in figure :
R=10Ω,L=√310H,R2=20Ω and C=√32mF. Current in L−R1 circuit is I1 in C−R1 circuit is I2 and the main current is I
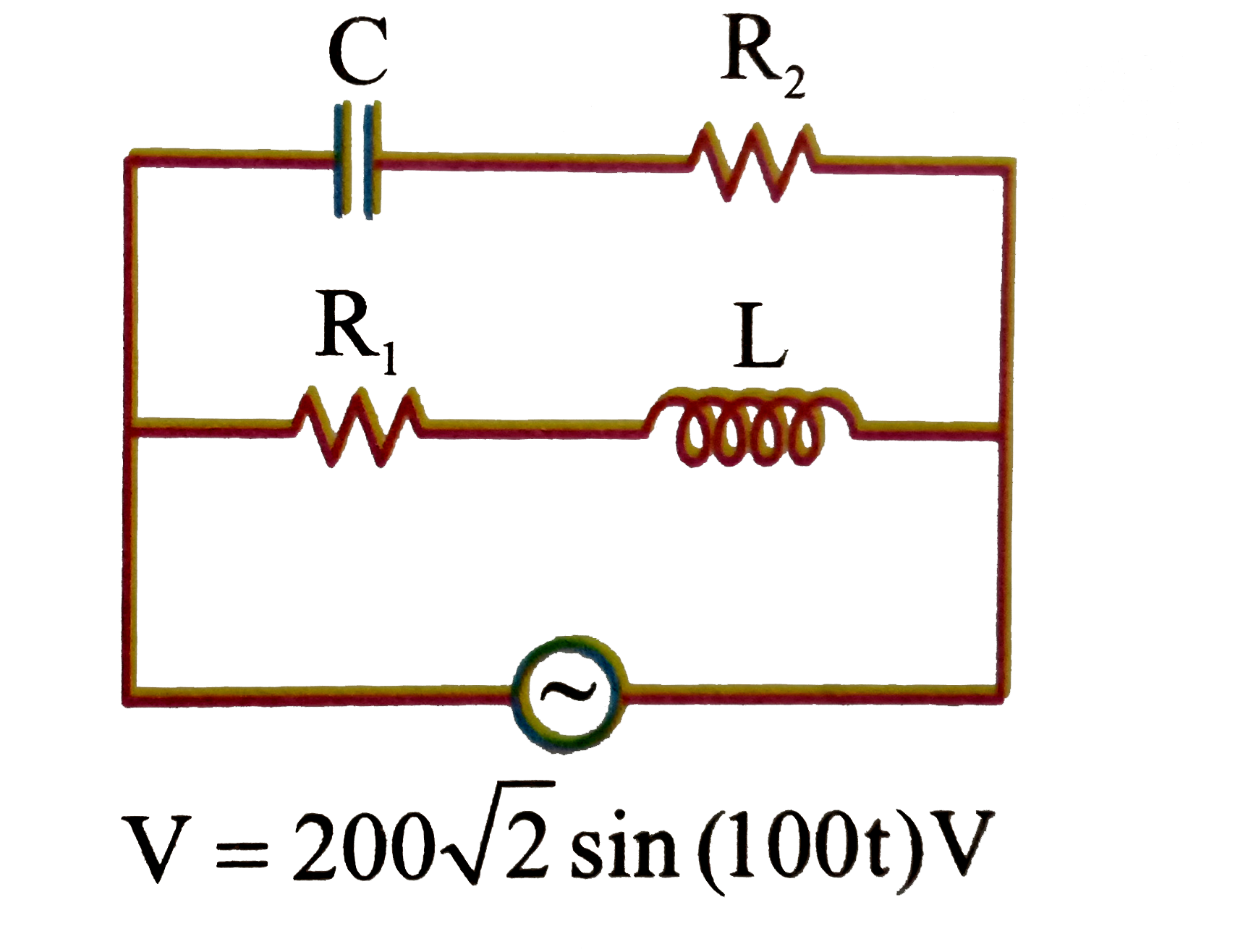
Phase difference between I1 and I2 isA0∘B90∘C180∘D60∘In the circuit shown in figure :
R=10Ω,L=√310H,R2=20Ω and C=√32mF. Current in L−R1 circuit is I1 in C−R1 circuit is I2 and the main current is I
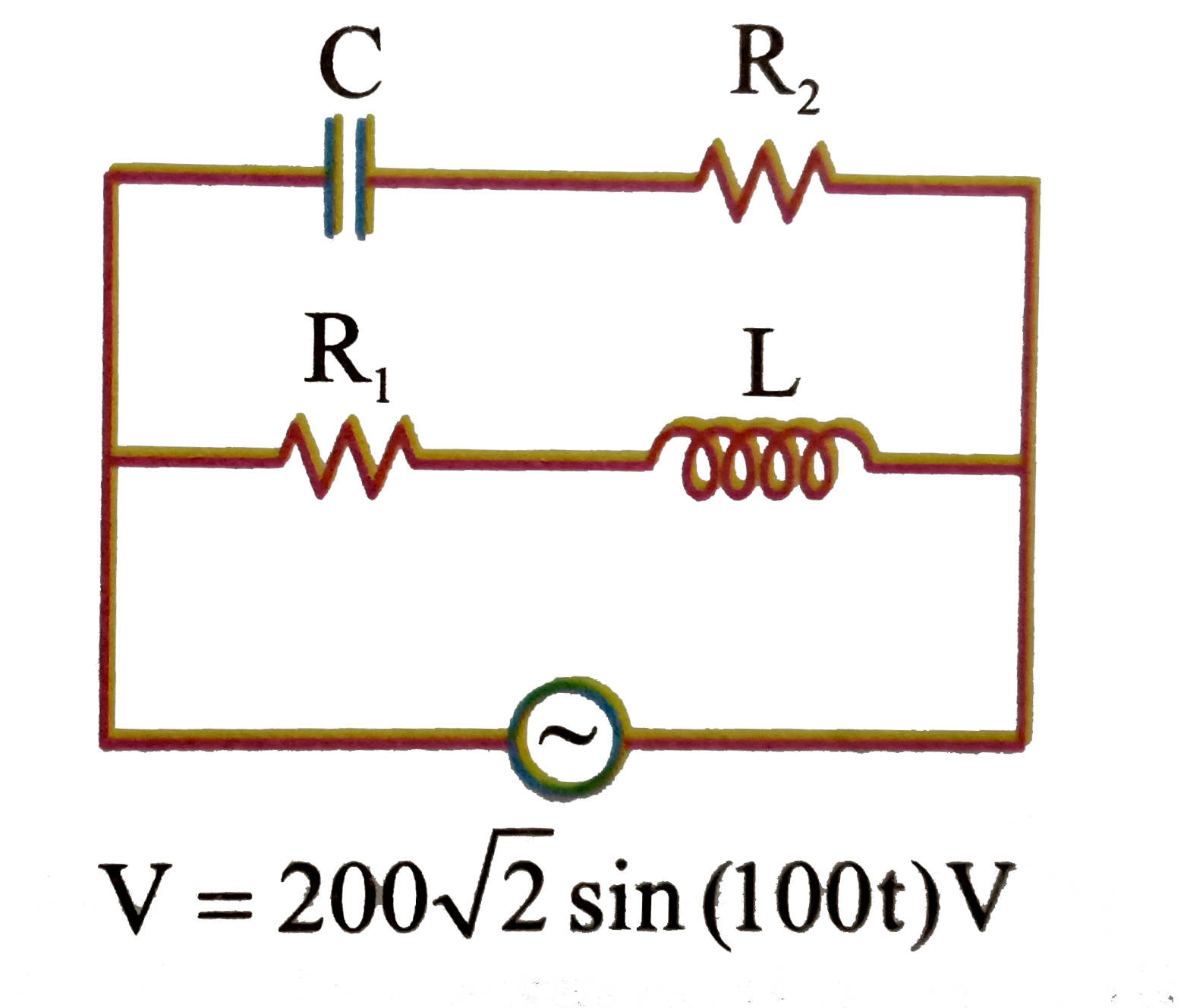
At some instant I1 in the circuit is 10√2A, then at this instant current I will beA20AB10√2AC20√2AD25AThe current in the series L-C-R circuit is
Ai=imsin(ωt+ϕ)Bi=vm√R2+(Xc−XL)2sin(ωt+ϕ)Ci=2imcos(ωt+ϕ)DBoth (a) and (b)
In the circuit shown in figure

Current through R2 is zero if R4=2Ω and R3=4Ω In this case
View SolutionIf the impedance of an L-C R circuit is 40Ω, then the admitance of the circuit will be
View SolutionIn the circuit shown in fig. XC=100Ω,(XL)=200ΩandR=100Ω. The effective current through the source is

View SolutionIn the circuit shows in Fig E=15V, R1=1Ω, R2=1Ω, R3=2Ω, and L=1.5H. The currents flowing through R1,R2,and R3 are i1,i2, and i3, respectively.
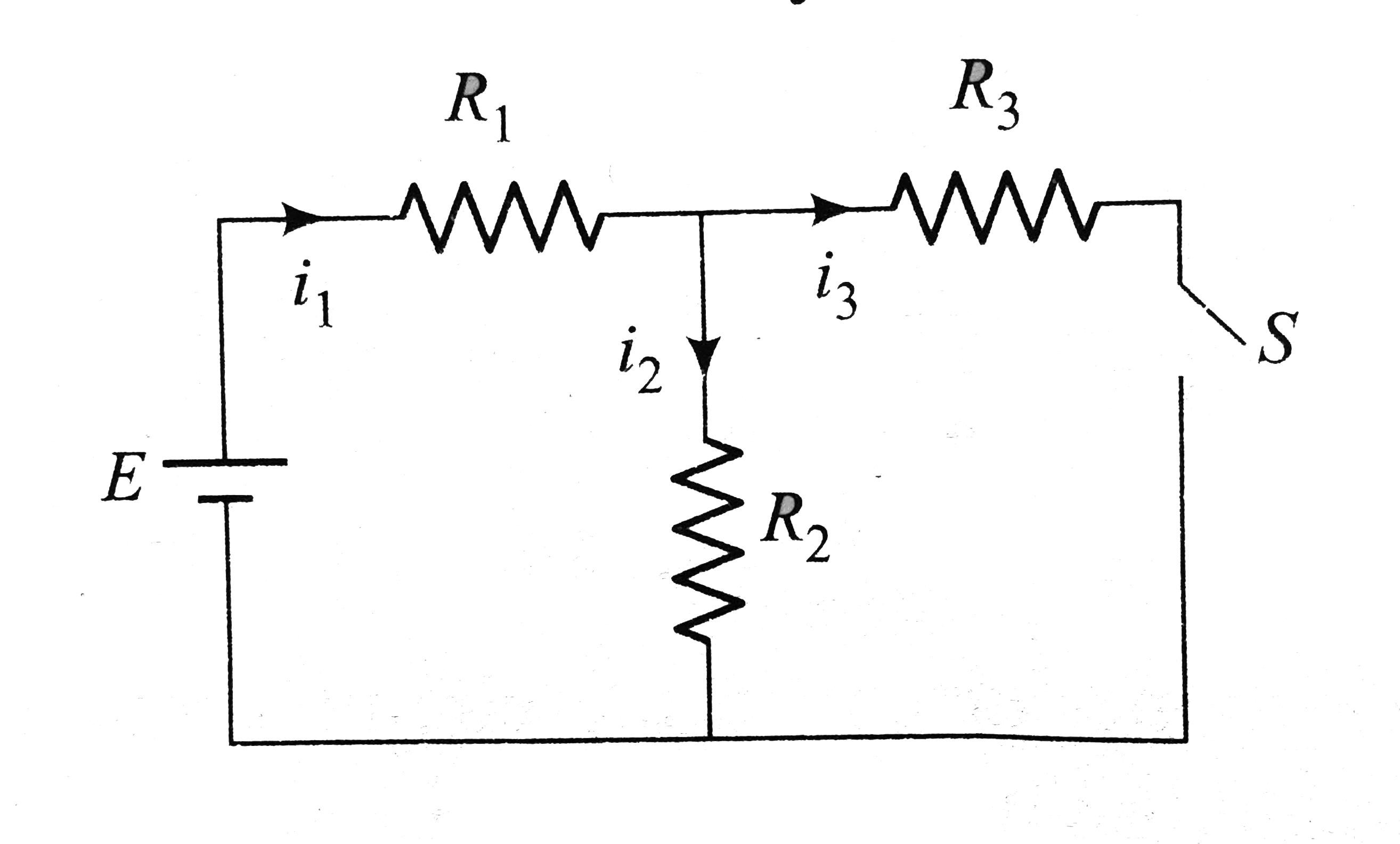
After the circuit reaches the steady state,
View SolutionAt resonance the peak value of current in L−C−R series circuit is
View Solution