A light ray parallel to the principal axis is incident (as shown in the figure) on a thin planoconvex lens with radius of curvature of its curved part equal to 10 cm. Assuming that the refractive index of the material of the lens is 4/3 and medium on both sides of the lens is air, find the distance of the point from the lens where this ray meets the principal axis. Find your answer in the form X7cm and fill value of X .
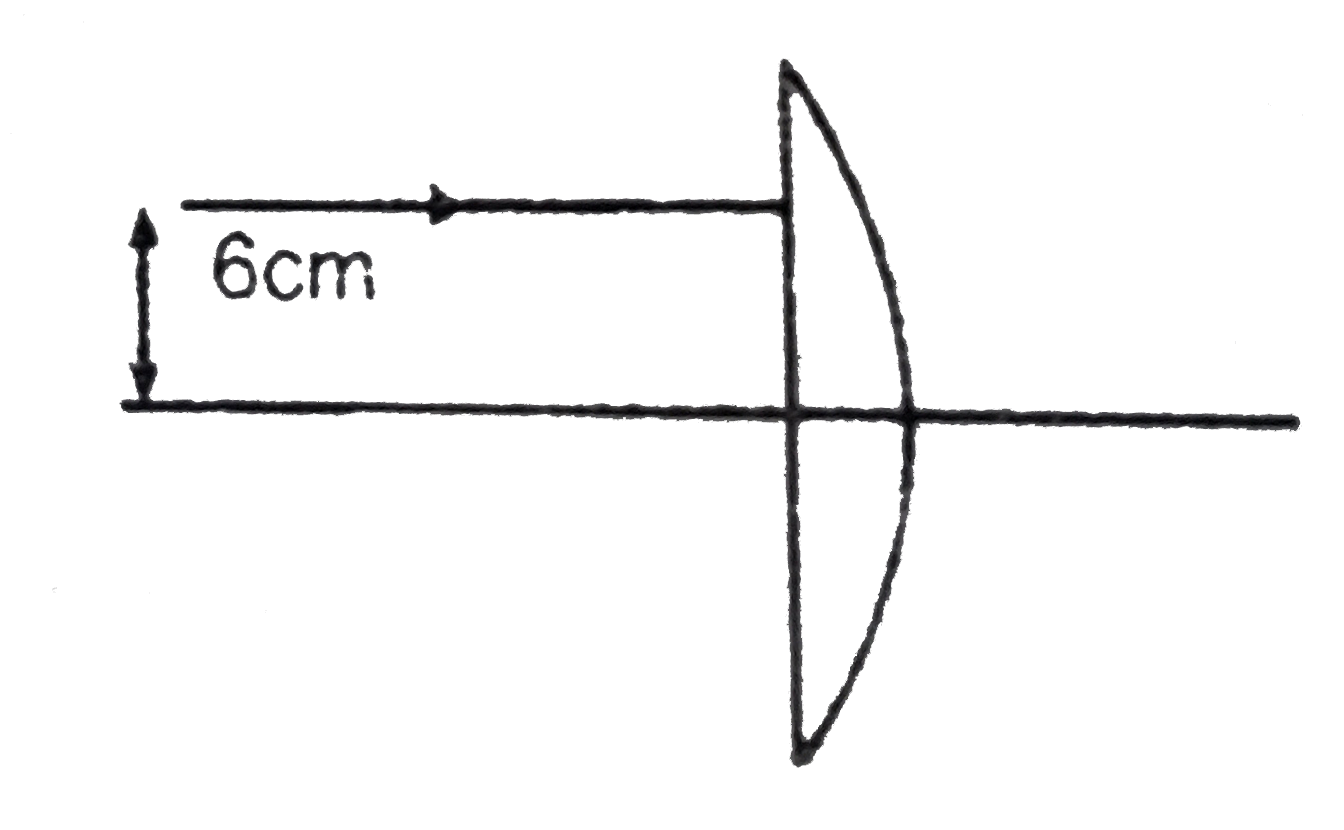
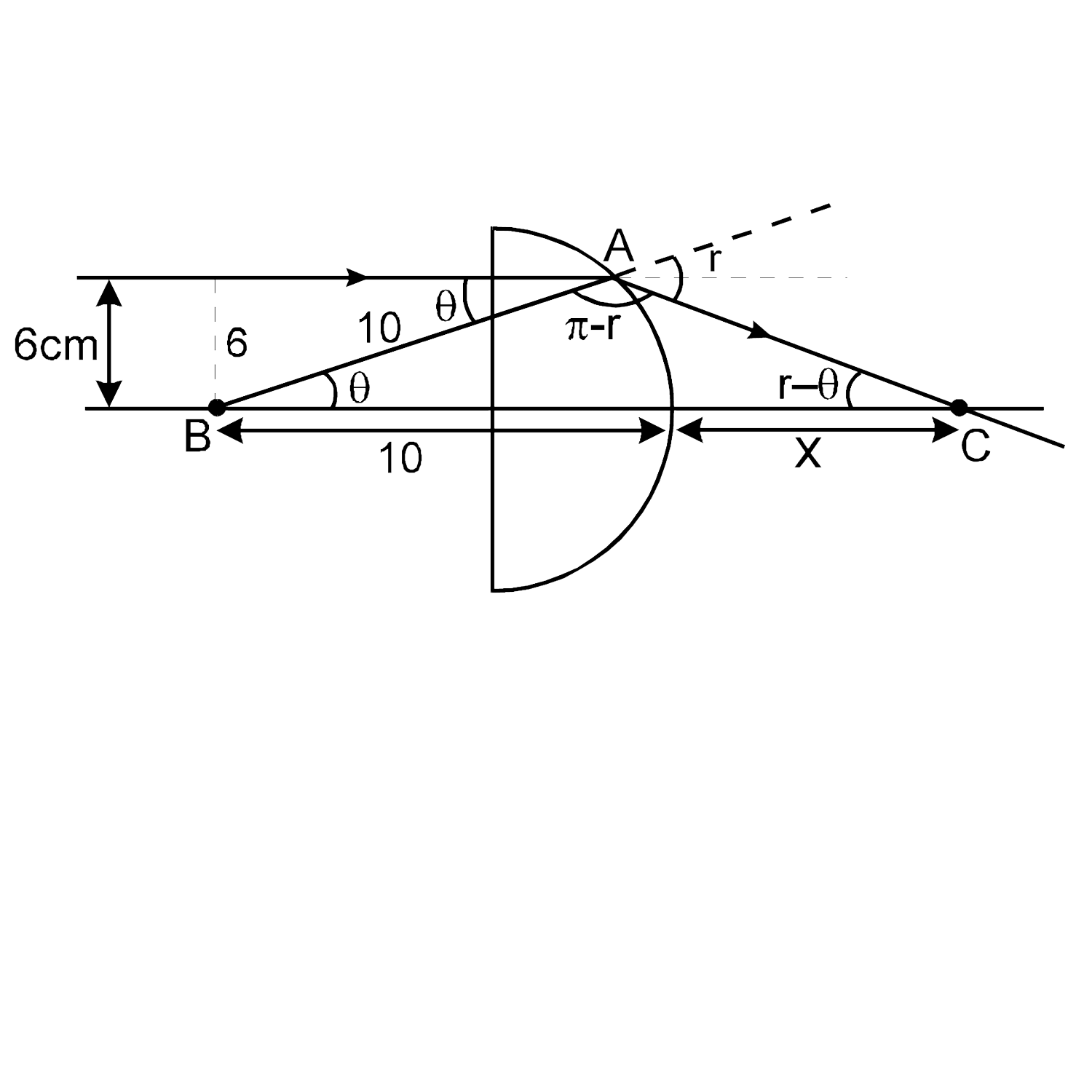
R=10cm
Applying snell's law
sinθsinr=34⇒r=53∘
By sine law in △ABCsin(r−θ)10=sin(π−t)(10+x),10+x10=45(sinrcosθ−cosrsinθ)
=45(45×45−35×35) 10+x=2007⇒x=200−707=1307
Topper's Solved these Questions
Similar Questions
The power of a biconvex lens is 10 dioptre and the radius of curvature of each surface is 10 cm. Then the refractive index of the material of the lens is
View SolutionThe power of a biconvex lens is 10 dioptre and the radius of curvature of each surface is 10 cm. Then the refractive index of the material of the lens is
View Solution
Knowledge Check
- Question 1 - Select One
The power of a biconvex lens is 10 dioptre and the radius of curvature of each surface is 10 cm. Then the refractive index of the material of the lens is
A3/2B4/3C9/8D5/3 - Question 2 - Select One
For a plano convex lens, the radius of curvature of convex surface is 10 cm and the focal length is 30 cm. The refractive index of the material of the lens is
A1.5B1.66C1.33D2.5 - Question 3 - Select One
If in a planoconvex lens, the radius of curvature of the convex surface is 10cm and the focal length is 30cm, the refractive index of the material of the lens will be
A1.5B1.66C1.33D3
The radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
View SolutionIf in a plano-convex lens, the radius of curvature of the convex surface is 10 cm and the focal length of the lens is 30 cm , then the refractive index of the material of lens will be
View SolutionPower of biconvex lens is 10 diopters and radius of curvature of each surface is 10cm.Then refractive index of material of lens is
View SolutionIf in a plano-convex lens, the radius curvature of the convex surface is 10 cm and the focal length of the lens is 20 cm , then the refractive index of the material of lens will be
View SolutionIf in a plano-convex lens, the radius curvature of the convex surface is 10 cm and the focal length of the lens is 30 cm, then the refractive index of the material of lens will be
View Solution

