A meteorite approaching a planet of mass M (in the straight line passing through the centre of the planet) collides with an automatic space station orbiting the planet in a circular trajectory of radius R. The mass of the station is ten times as large as the mass of the meteorite. As a result of the collision, the meteorite sticks in the station which goes over to a new orbit with the minimum distance R/2 from the planet. Speed of the meteorite just before it collides with the planet is : .

$\sqrt{\frac{58 G M}{R}}$

$\sqrt{\frac{38 G M}{R}}$

$\sqrt{\frac{28 G M}{R}}$

$\sqrt{\frac{18 G M}{R}}$

Video Solution

Text Solution

Verified by Experts

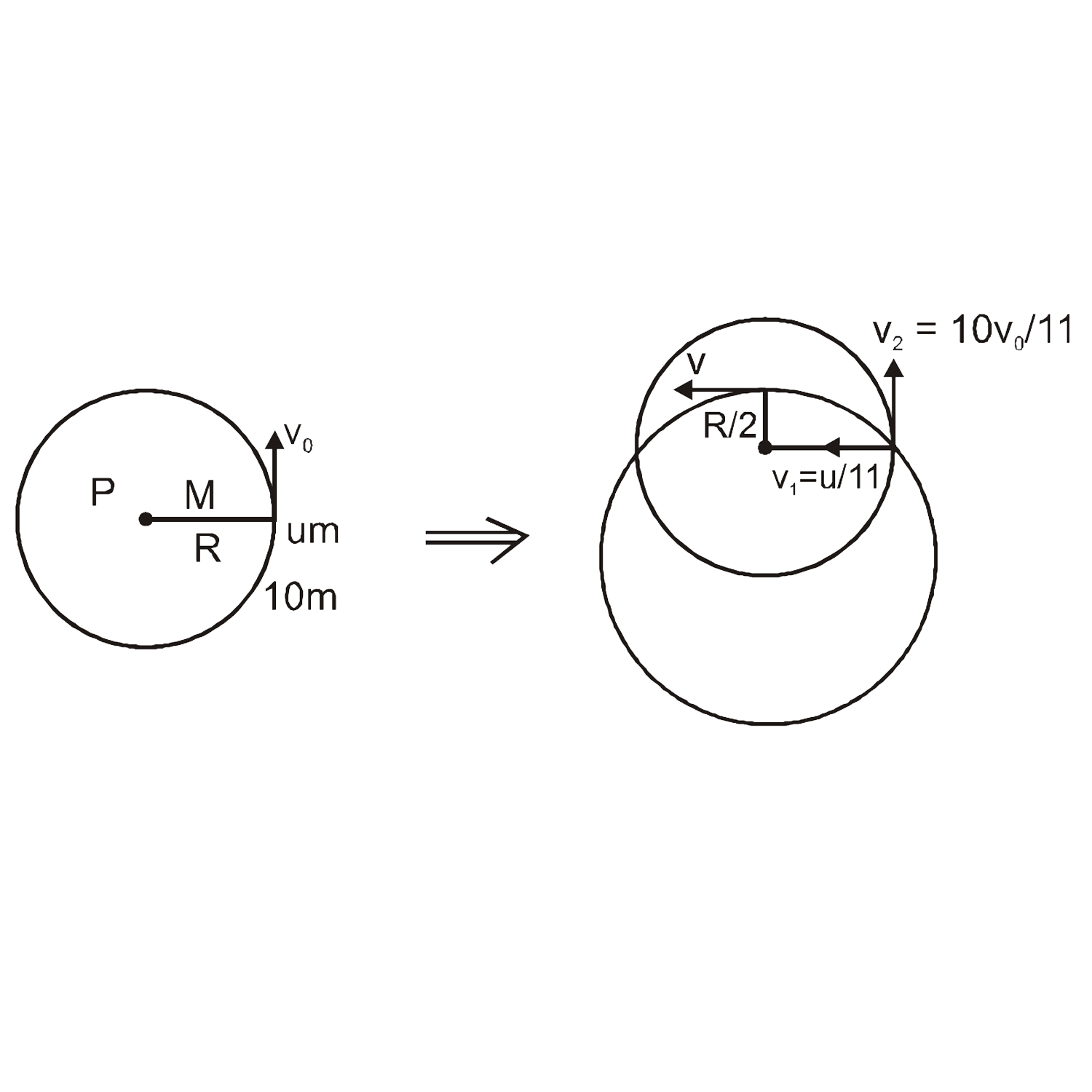
As the space station is moving in circular orbit,
$\frac{G M (10 m)}{R^{2}} = \frac{(10 m) v_{0}^{2}}{R}$
$\Rightarrow v_{0} = \sqrt{\frac{G M}{R}}$ ..(i)
Let u be the velocity of meteorite.
Velocity of the space station after collision can be obtained from momentum conservation
$μ = (10 m + m) v_{1} \Rightarrow v_{1} = \frac{u}{11}$
$10 m, v_{0} = (10 m + m) v_{2} \Rightarrow v_{2} = \frac{10}{11} v_{0}$
Let v be the velocity space stationa at closest distance
from angu7lar momentum conservation
$10 m v_{0} \times R = 11 m v \frac{R}{2} \Rightarrow v = \frac{20 v_{0}}{11}$
from energy conservation
$\frac{1}{2} \times (11 m) (v_{1}^{2} + v_{2}^{2}) - \frac{G M (11 m)}{R} = \frac{1}{2} \times (11 m) v^{2} - \frac{G M .11 m}{R / 2}$
$\Rightarrow {(\frac{u}{11})}^{2} + {(\frac{10 v_{0}}{11})}^{2} - \frac{2 G M}{R} = {(\frac{20 v_{0}}{11})}^{2} - \frac{4 G M}{R}$
$\Rightarrow \frac{u^{2}}{11^{2}} = \frac{400 v_{0}^{2}}{11^{2}} - \frac{100 v_{0}^{2}}{11^{2}} - \frac{2 G M}{R}$
$\Rightarrow u^{2} = \frac{G M}{R} (400 - 100 - 242) = 58 \frac{G M}{R}$
Ans $u = \sqrt{\frac{58 G M}{R}}$

Updated on:21/07/2023