Figure, shows a vertical cross section of a surface. A and B are two points on the cross sections. A particle of mass 0.15kg is released from rest at A. (i) Assuming that particle reaches B with a speed of 8m/s and there is no resistance to motion, find the height of A above B.
(ii) Assuming instead that the particle reaches B with a speed of 6m/s and that the height of A above B is 4m, find work done against resistance to motion.
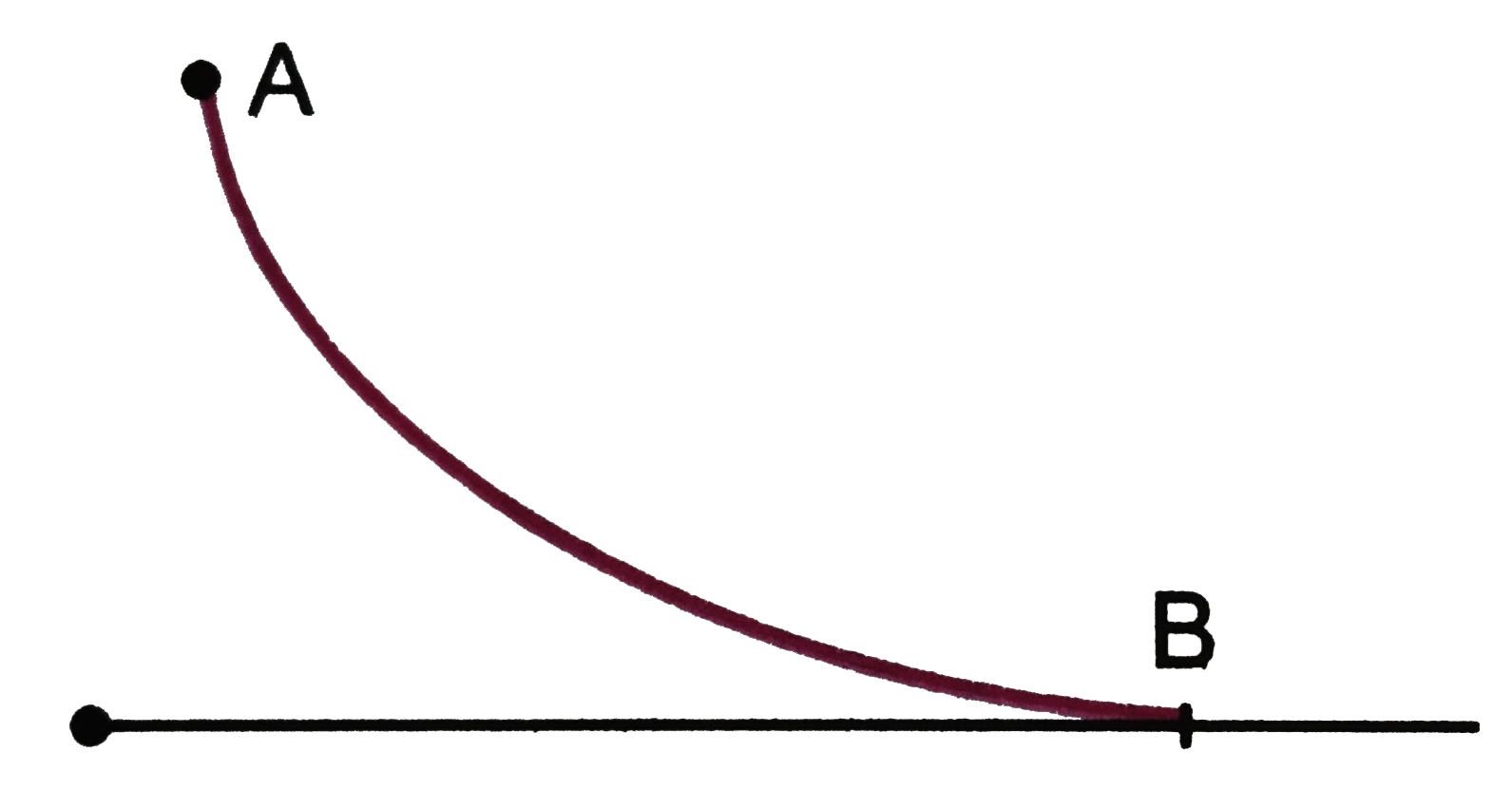
(i) Here, m=0.15kg,vB=8m/s
If h is height of A above B, then accordint to the law of conservation of energy,
loss in PE (from A to B ) = gain in KE (at B)
mgh=12mv2
h=v22g=8×82×9.8=3.27m
(ii) Now, h′=4m,vB=6m/s
Accordint to the law of conservation of energy, Loss in PE (from A to B) = gain in KE at B + wokr down against friction
mgh′=12mv2B+W
W=mgh′−12mv2B
=0.15×9.8×4−12×0.15×62
W=5.88−2.7
W=3.18J
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - NCERT EXERCISES WITH SOLUTIONS
30 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Additional Exercises
3 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Long Answer Question
24 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
The sign of work done by a force on a body is important to understand. State carefully if the following quantities are poisitve or negative :
(a) Work done by a man in lifting a bucket out of a well by means of a rope tied to the bucket.
(b) Work done by gravitational force in the above case. (c ) Work done by friction on a body sliding down an iniclined plane.
(d) Work done by an applied force on a body moving on a rough horizontal plane with uniform velocity.
(e) Work done by the resistive force of air on a vibrating pendulum in bringing it to rest.
View SolutionA and B are two particles having the same mass m. A is moving along X-axis with a speed of 10ms−1 and B is at rest. After undergoing a perfectly elastic collision with B, particle A gets scattered through an angle of 30∘. What is th edirection of motion of B, and the speeds of A and B, after the collision?
View SolutionExplain what is meant by work. Obtain an expression for work done by a constant force.
View SolutionAssertion : Internal energy of an ideal gas does not depend upon volume of the gas.
Reason : This is because internal energy of ideal gas depends only on temperature of gas.
View SolutionShown a conductor of length l having a circular cross section. The radius of cross section varies linearly form a→b. The resistivity of the material is(ρ).Assuming that b−a<<l,find the resistance of the conductor.

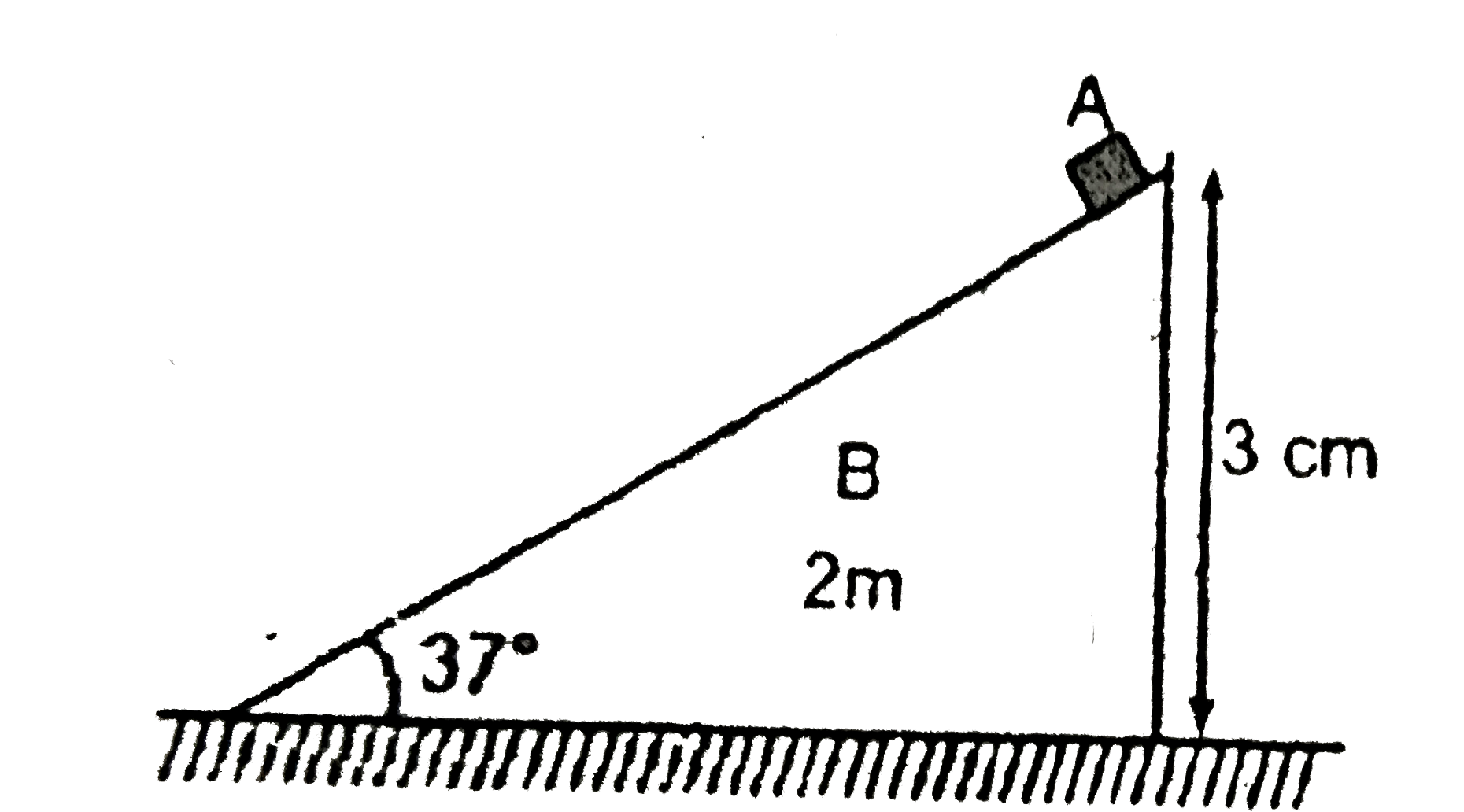
View SolutionA particle A of mass m is situated at highest point of wedge B of mass 2m is released from rest. Then distance travelled by wedge B( with respect to ground ) when particle A reaches at lowest position. Assume all surfaces are smooth.
View SolutionTwo particle A and B (of masses m and 4m ) are released from rest in the two tunnels as shown in the figure-6.93. Which particle will cross the equatorial plane first?
View SolutionTwo particles A and B are projected simultaneously from a fixed point of the ground. Particle A is projected on a smooth horizontal surface with speed v, while particle B is projected in air with speed v, while particle B is projected in air with speed (2v)/(sqrt(3)) . If particle B hits the particle A, the angle of projection of B with the vertical is
View SolutionFigure shows a conductor of length l having a circular cross-section. The radius of cross-section varies linearly from a to b. Find the resistance of the conductor. ltbtgt
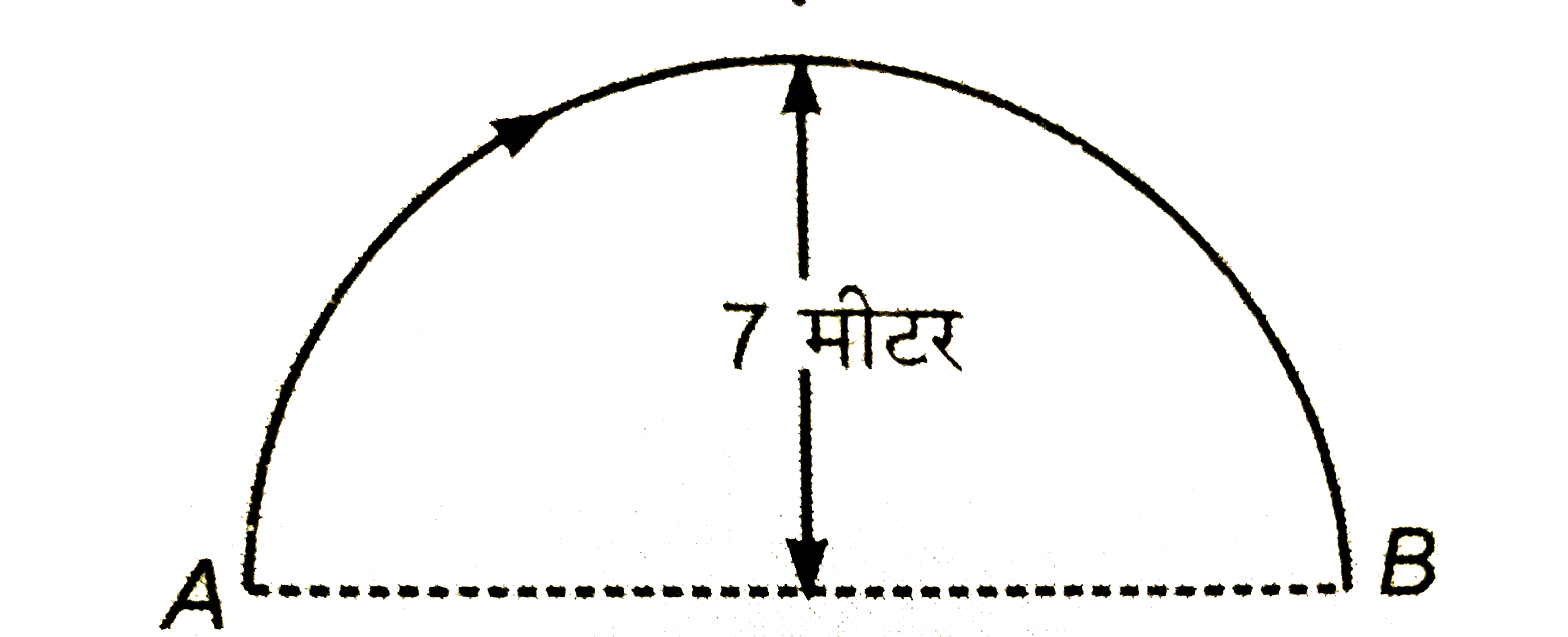
View Solutionएक कण 7 मीटर त्रिज्या के अर्धवृत पर बिंदु A से बिंदु B तक 10 सेकण्ड में पहुंचता है कण की औसत चाल तथा इसका औसत वेग ज्ञात कीजिय ।
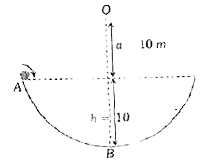
View Solutionचित्रानुसार 20 g द्रव्यमान के एक कण को 5m/s व वक्र के अनुगत प्रारंभिक वेग से बिंदु A से छोड़ा जाता है । बिंदु A की बिंदु B से ऊंचाई h है । यह कण घर्षणहीन पृष्ठ पर सरकता है। जब कण बिन्दु B पर पहुंचता है , तो इसका बिंदु O के सापेक्ष कोणीय संवेग क्या होगा -
View SolutionTwo particles A and B having different masses are projected from a tower with same speed. A is projected vertically upward and B vertically downward. On reaching the ground
View Solutionmass ‘m’ is projected vertically upwards with a speed ‘u’ and at the same instance another particle of mass ‘m’ is released from height ‘h’ vertically above the first particle. The two particles suffer a perfectly inelastic collision, and again reach a maximum height ‘h’ as one combined particle. What is the value of u?
View Solutionदो कण A तथा B, जिनका द्रव्यमान अलग-अलग है, को एक टॉवर से एक समान गति से प्रक्षेपित किया जाता है। कण A ऊर्ध्वाधर ऊपर की ओर तथा कण B ऊर्ध्वाधर नीचे की ओर प्रक्षेपित होता है, सतह पर पहुंचने पर
View SolutionShown a conductor of length l having a circular cross section. The radius of cross section varies linearly form a→b. The resistivity of the material is(ρ).Assuming that b−a<<l,find the resistance of the conductor.

View Solution
