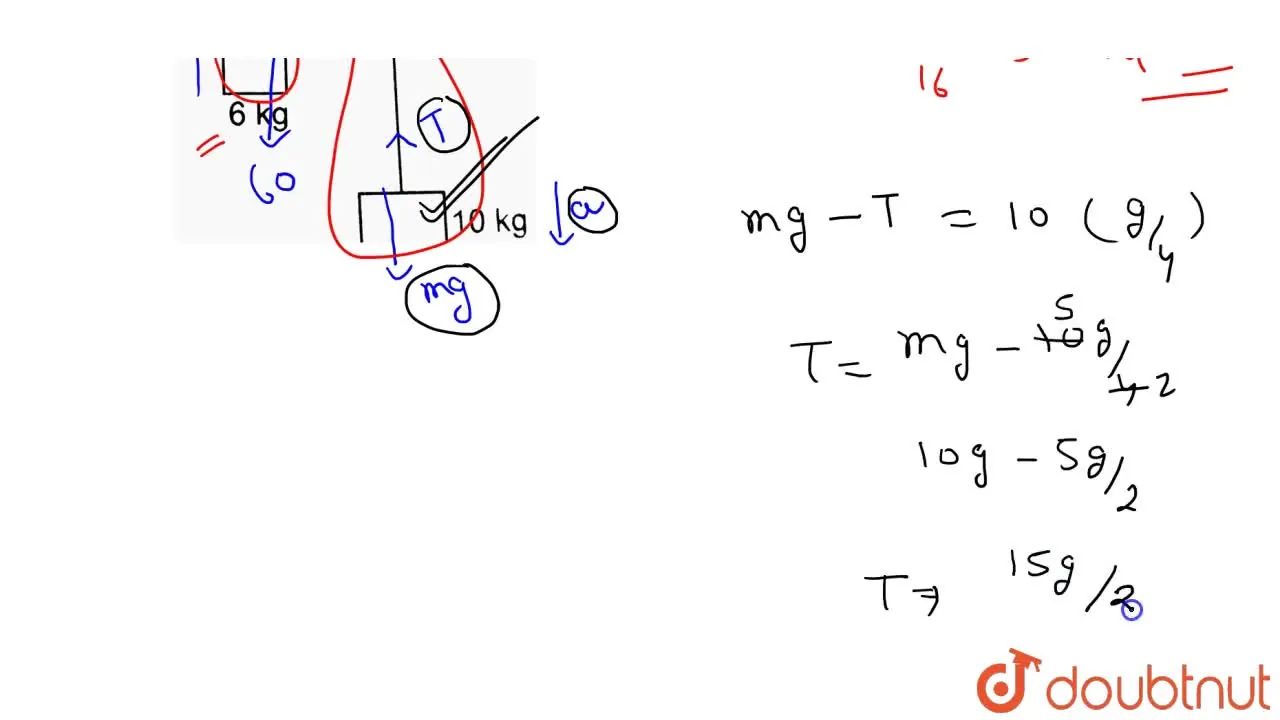
The tension in the string in the pulley system shown in
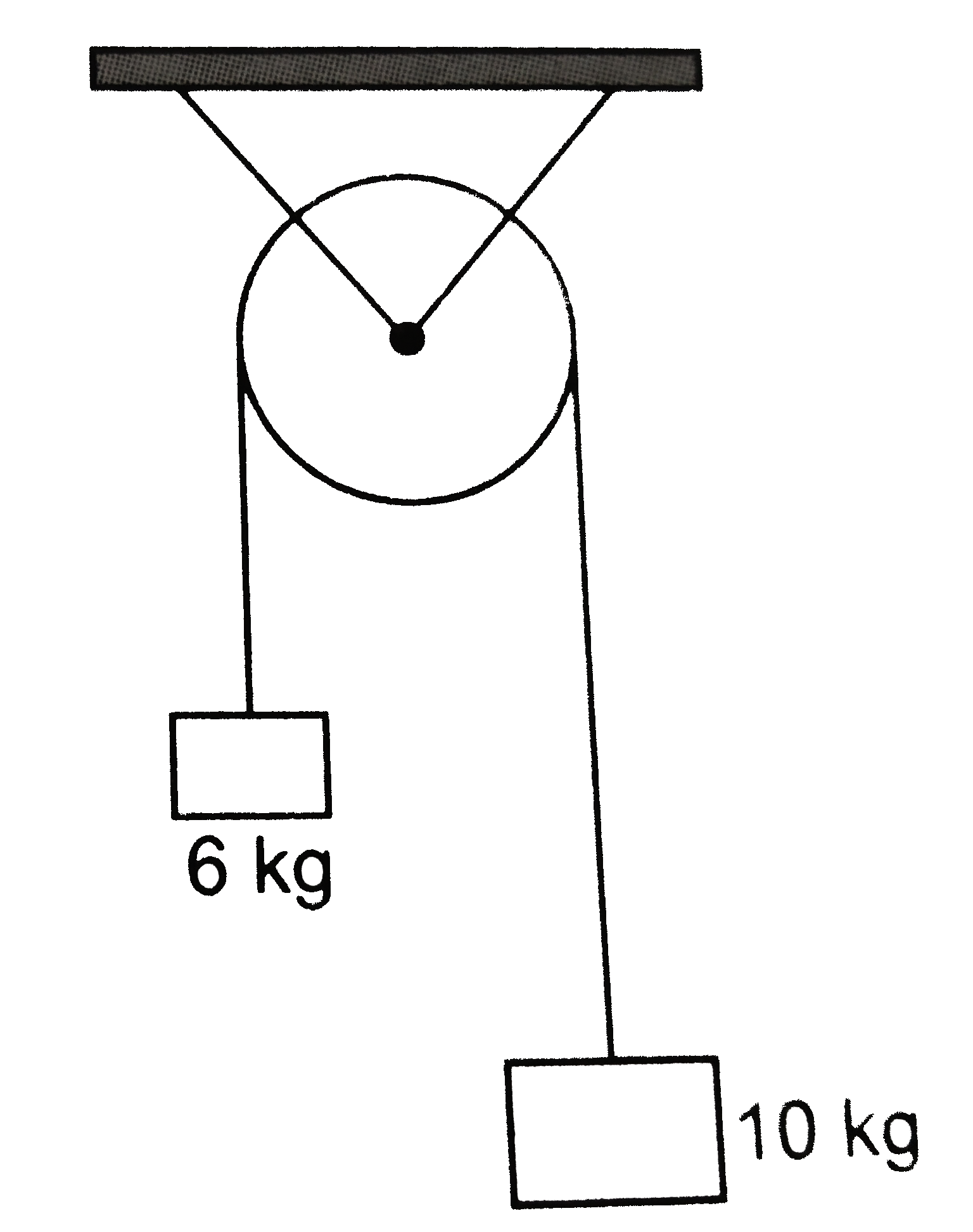 .
.
5.7N
7N
7.5N
73.5N
The correct Answer is:d
T=2m1m2gm1+m2=2×6×10×9.8(6+10)=12×9816
=73.5N .
Related Playlists
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Integer Type Questions7videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions17videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Problems for practice112videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension4videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks5videos
MATHEMATICAL TOOLS
Similar Questions
The tension in a string holding a solid block below the surface of a liquid (of density greater than that of solid) as shown in figure is T0 when the system is at rest. What will be the tension in the string if the system has an upward acceleration a?
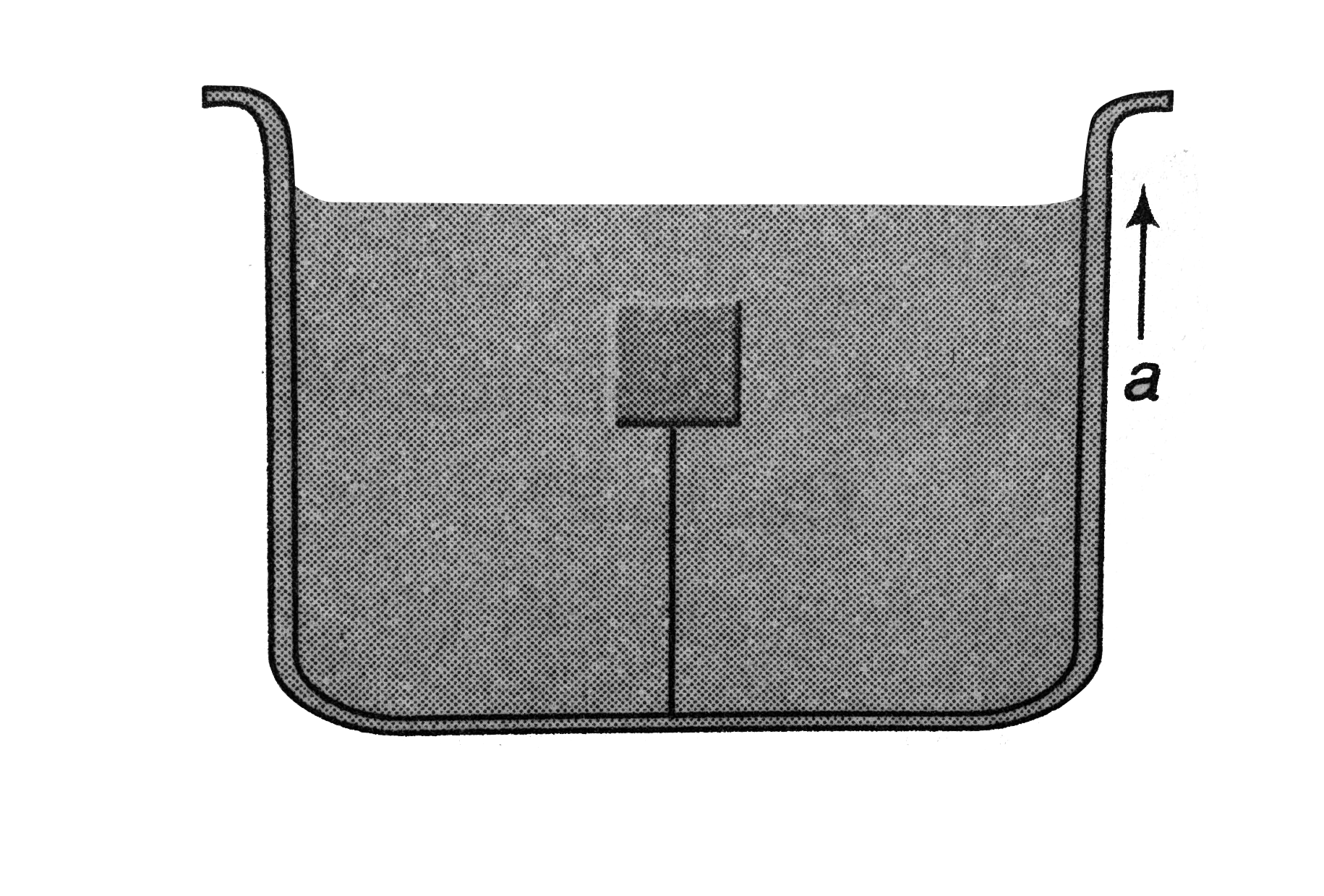 .View Solution
.View SolutionCalculate the accelerations of the pulleys B and C and the tension in the string passing over the pulley A of the figure Figure 5.22.
View SolutionFind the tension in the string and the extension in the spring at equilibrium . Where pulley, strings and springs are ideal.
 View Solution
View SolutionThe tension T in the string shown in figure is
 View Solution
View SolutionCalculate the tension in the string shown in
(a) The pulleys and the string are light and all surfaces are frictionless. (g=10m/s2)
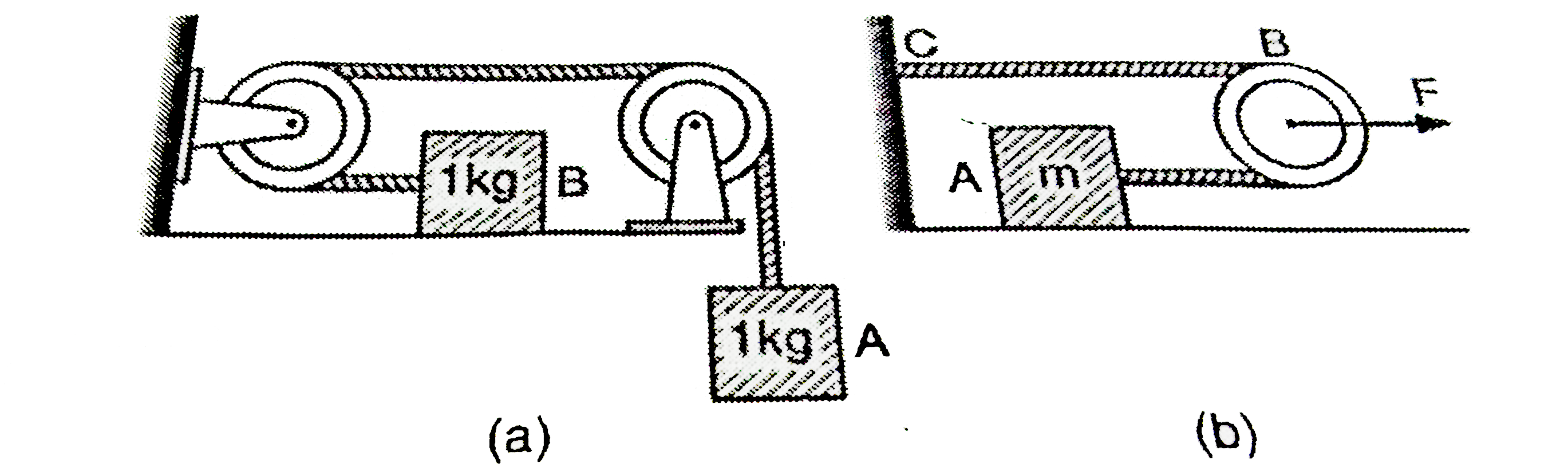 View Solution
View SolutionIn the arrangement shown, find the acceleration of each block, tension in the string and reaction in the pulley. The string and pulley are light (massless) (m1>m2). Neglect the friction in pulley.
 View Solution
View SolutionA string is would over a cylinder of mass M, radius R and moment of inertia I and then the string is would over the pulley as shown in the figure. If the system is released from rest then determine the tension is tring. Assume there is no slipping between string and pulley cylinder.
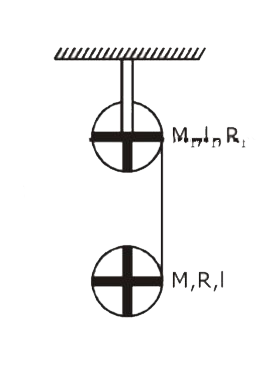 View Solution
View SolutionCalculate the tension in the string shown in figure. The pulley and the string are light and all surfaces are frictionless. Take g=10 m/s2.
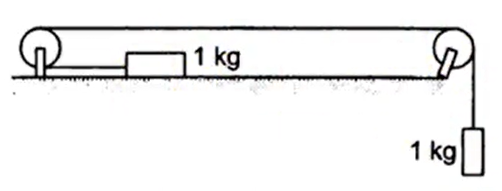 View Solution
View SolutionIn the system shown, the blocks A,B and C are of weight 4W, W and W respectively. The system set free. The tension in the string connecting the blocks B and C is
 View Solution
View SolutionThe system shown in fig, is released from rest. Calculate the tension in the string and the force exerted by the string on the pulleys, assuming pulleys and strings are massless.
 View Solution
View Solution