A block is gently placed on a conveyor belt moving horizontal with constant speed After t=4s the velocity of the block becomes equal to velocity of the belt If the coefficient of friction between the block and the belt is μ=0.2, then the velocity of the conveyor belt is .
8/s
6m/s
4m/s
2m/s
The correct Answer is:a
On account of friction (a=μg) velocity of block will become equal to velocity of block will become equal to velocity of conveyor belt and the relative motion between the two will stop
υ=at=μgt=0.2×10×4=8m/s .
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Integer Type Questions
7 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Problems for practice
112 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension
4 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
Similar Questions
A block lying on a long horizontal conveyer belt moving at a constant velocity receives a velocity v0=5m/s relative to the ground in the direction opposite to the direction of motion of the conveyer. After t=4s, the velocity of the block becomes equal to the velocity of the belt. The coefficient of friction between the block and the belt is μ=0.2. Determine the velocity v of the conveyer belt.
View SolutionFind out the distance travelled by the block on incline before it stops. Initial velocity of the block is 10 m/s and coefficient of friction between the block and incline is μ=0.5.
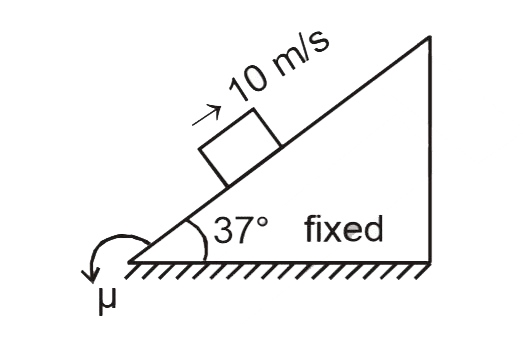
View Solution
Knowledge Check
In Fig. 7.149 , coefficient of friction between the block and the floor is μ . Force F that will move the block on the floor with a uniform speed is :
 A(μsinθ+cosθ)mgB(μsinθ−cosθ) mgCμmgcosθ−μsinθDμmgsinθ−μcosθ
A(μsinθ+cosθ)mgB(μsinθ−cosθ) mgCμmgcosθ−μsinθDμmgsinθ−μcosθA block of mass m is gently placed over a massive plank moving horizontal over a smooth surface with velocity 10ms−1. The coefficient of friction between the block and the plank is 0.2. The distance travelled by the block till it slides on the plank is [g=10ms−2]
A10 mB15mC25 mD35mAn object is gently placed on a long converges belt moving with 11ms−1. If the coefficient of friction is 0.4, then the block will side in the belt upto a distance of
A10.21 mB15.125 mC20.3 mD25.6 m
A block of mass m is pulled by a constant power P placed on a rough horizontal plane. The coeficient of friction between the block and surface is μ. Find the maximum velocity of the block.
View SolutionA suitcase is gently dropped on a conveyor belt moving at 3ms−1 If the coefficient of friction between the belt and suitcase is 0.5 how far will the suitcase move on the belt before coming to rest ?
View SolutionA block slides with a velocity of 10 m/s on a rough horizontal surface. It comes to rest after covering a distance of 50 metre. If g is 10m/s2, then the coefficient of dynamic friction between the block and the surface is
View SolutionA block of mass m is placed on a vertical fixed circular track and then it is given velocity v along the track at position A on track. The coefficient of friction between the block and the track varies with the angle θ . If the block moves on track with constant speed then the coefficient of friction is
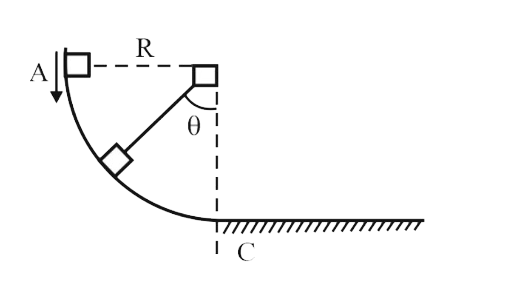
View SolutionAn object is gently placed on a long conveyer belt moving with a speed of 5 ms−1 If the coefficient of friction between the object and the belt is 0.5, the block will slide on the belt up to a distance (Take g = 10 ms−2)
View Solution
