A target is fixed on the top of a tower 13m high. A person standing at a distance of 50m from the pole is capable of projecting a stone with a velocity 10√gms−1. If he wants to strike the target in shortest possible time, at what angle should he project the stone ?
From the equtaion of path of projectile,
y=xtanθ−gx22u2cos2θ, we have
13=50tanθ−g(50)22×(10√g)2cos2θ
=50tanθ−252sec2θ 13=50tanθ−252(1+tan2θ)
or 25tan2θ−100tanθ+51=0
tanθ=⎛⎜
⎜⎝100=−√(100)2−4×25×5150
=155or35
The horzontal distance coverd in time (t) is given
by x= ucos theta t or 50 = (10 sqrt g) cos theta t<br> or t=5010√gcosθ
Hence, (t) will be minimum of the value of csoθ is maximum. It will be so if the value of tanθ is least
i.e. tanθ=35=0.6 or θ30∘58'.
Topper's Solved these Questions
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 Multiple choice
10 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 2 Multiple choice
10 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 3 Problems for practice
67 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions
19 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
Similar Questions
A target is fixed on the top of a pole 13 metre high. A person standing at a distance of 50 metre form the pole is capable of projecting a stone with a velocity 10√gms−1. If he wants to strike the target in shortest possible time, at what angle should he project the stone ?
View Solution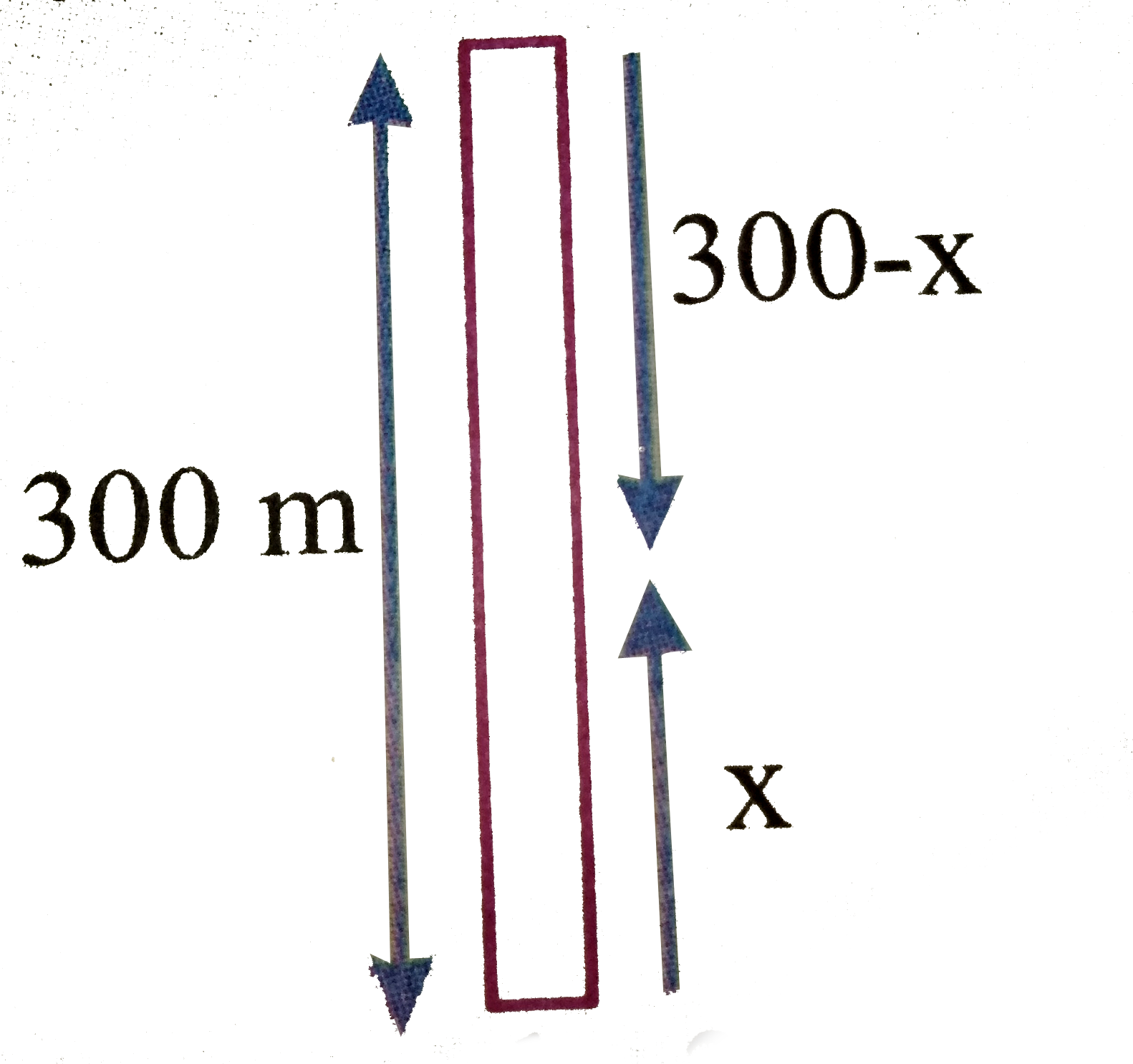
A stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100m/s. Find when and where the two stones meet?
View Solution
Knowledge Check
A stone is dropped from the top of a tower and one second later, a second stone is thrown vertically downward with a velocity 20ms−1. The second stone will overtake the first after travelling a distance of (g=10ms−2)
A13mB15mC11.25mD19.5mFrom the top of a tower of height 100 m, a ball is projected with a velocity of 10m/sec. It takes 5 seconds to reach the ground. If g=10m/sec2 then the angle of projection is
A30∘B45∘C60∘D90∘A ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
A4sB−28sC12sD−2s
A stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stone will meet.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25ms.Calculate when and where the two stones will meet.
View SolutionA stone is dropped from the top of a tower 200 m high. At the same time, another stone is projected vertically upwards from the ground with a velocity of 40m/s. Calcuate when and where the two stones will mest.
View SolutionA body thrown horizontally from the top of a tower touches the ground at a distance of 160m from the foot of the tower.If the velocity of projection is 40m/s then the height of the tower is (g=9.8ms2)
View SolutionA stone is thrown from the top of a tower at an angle of 30∘ above the horizontal level with a velocity of 40 m/s. it strikes the ground after 5 second from the time of projection then the height of the tower is
View Solution