A target is fixed on the top of a pole 13 metre high. A person standing at a distance of 50 metre form the pole is capable of projecting a stone with a velocity 10√gms−1. If he wants to strike the target in shortest possible time, at what angle should he project the stone ?
Step by step video & image solution for A target is fixed on the top of a pole 13 metre high. A person standing at a distance of 50 metre form the pole is capable of projecting a stone with a velocity 10 sqrt(g) ms^(-1). If he wants to strike the target in shortest possible time, at what angle should he project the stone ? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROJECTILE MOTIONBOOK - SL ARORACHAPTER - PROJECTILE MOTIONEXERCISE - Problen Solution
17 Videos
- PROJECTILE MOTIONBOOK - SL ARORACHAPTER - PROJECTILE MOTIONEXERCISE - Problem For Self Practice
50 Videos
- PHYSICAL WORLD AND MEASUREMENTSBOOK - SL ARORACHAPTER - PHYSICAL WORLD AND MEASUREMENTSEXERCISE - Based on Combination
21 Videos
- PROPERTIES OF SOLIDSBOOK - SL ARORACHAPTER - PROPERTIES OF SOLIDSEXERCISE - All Questions
97 Videos
Similar Questions
A target is fixed on the top of a tower 13m high. A person standing at a distance of 50m from the pole is capable of projecting a stone with a velocity 10√gms−1. If he wants to strike the target in shortest possible time, at what angle should he project the stone ?
View SolutionAt what angle should a body be projected with a velocity 20ms−1 just to pass over the obstacle 12m high at a horizontal distance of 24m? Take g=10−2).
View Solution
Knowledge Check
A truck is moving with a constant velocity of 54kmh−1. In which direction (angle with the direction of motion of truck) should a stone be projected up with a velocity of 20ms−1, from the floor of the truck of the truck, so as to appear at right angles to the truck, for a person standing on earth ?
Acos−1(−34)Bcos−1(−14)Ccos−1(−24)Dcos−1(34)A stone is projected from the ground with a velocity of 14ms−1. One second later it clears a wall 2m high. The angle of projection is (g=10ms−2)
A45∘B30∘C60∘D15∘A river is flowing from west to east at a speed of 5 metres per minute.A man on the south bank of the river, capable of swimming at 10 metres per minute in still water, wants to swim across the river in the shortest time. He should swim in a direction.
ADue northB30∘ each of northC30∘ west of northD60∘ east of north
A particle is dropped from the top a tower h metre high and at the same moment another particle is projected upward from the bottom. They meet the upper one has descended a distance h/n . Show that thevelocities of the two when they meet are in the ratio 2: (n−2) and that the initial velocity of the particle projected upis √(1/2)ngh.
View SolutionThere is a tall cylindrical building standing in a field. Radius of the cylinder is R=8m. A boy standing at A (at a distance of 10 m from the centre of the cylindrical base of the building) knows that his friend is standing at B behind the building. The line joining A and B passes through the centre of the base of the building. Distance between A and B is 50 m. A wants to throw a ball to B but he realizes that the building is too tall and he cannot throw the ball over it. He throws the ball at a speed of 20m/s such that his friend at B has to move minimum distance to catch it.
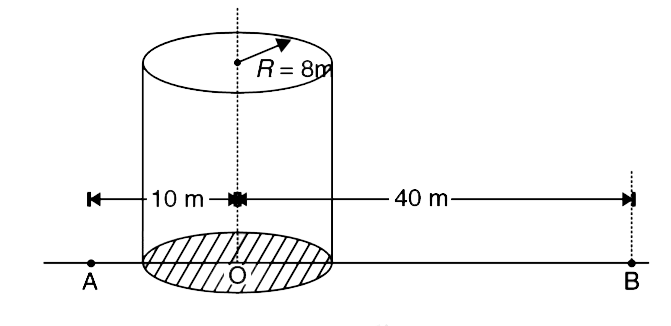
(a) What is the minimum distance that boy at B will have to move to catch the ball?
(b) At what angle to the horizontal does the boy at A throws the ball? Assume that the ball is released and caught at same height above the ground.
[Take g=10m/s2 and sin−1(0.75)≅48.6∘
View SolutionA stone is dropped from a height of 100m .At the same instant another stone is projected vertically upwards with velocity of 50ms^(-1) .If g=10ms^(-2) ,the height from the ground at which the 2 stones meet is
View SolutionA river is flowing from west to east at a speed of 5metresperminute . A man on the south bank of the river , capable of swimming at 10metresperminute, in still water , wants to swim across the river in the shortest time . He should swim in a direction
View SolutionAn object is project with a speed 10ms−1 at an angle of 30∘ with the horizontal. It breaks into n equal fragments during its motion . One fragment strikes the ground at a distance of √3m from the point of projection. The centre of mass of the remaining fragments strikes the ground at a distance of 7√3m from the point of projection . If all fragments strike the ground at the same time , Find the value of n.
View Solution
