Explain clearly, with ezamples, the distaintion between :
(a) magnitude of displacemnt (sometimes called distance ) overand interval of time, and the total length of the path coverd by a particle over the same interval.
(b) magnitude of average velocity over an intercal of time , and the average speed ocer the same interval. [ Average speed of a particle over an interval of time is defined as the toal path length divided by the time intrval]. Show in both (a) and (b) that the second quantity is either greater than or equal to first. When is the equality sing true ? [ For simplocity, consider one- dimensional motion only]
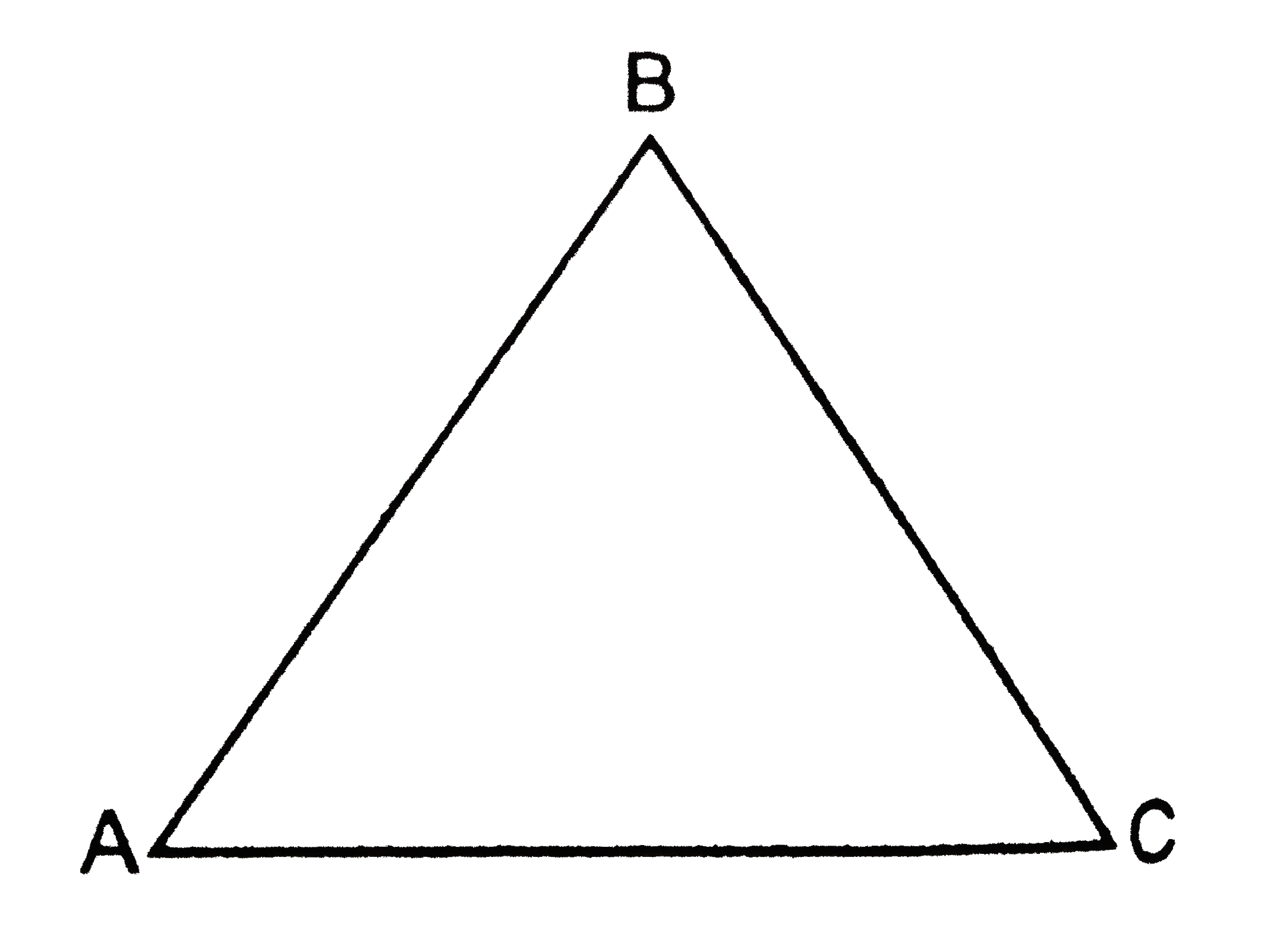
(a) Magnitude of displacement of a particle in motion for a given time is the shortest distance between the initial and final position of the particle in that time, whereas the total length of the path covered by particle is the actual path traversed by the particle in the given time. If a particle goes from A→B to C∈time(t)asshown∈Fig.2(NCT).4,thenMagnitudeofdisplacrmt=distance Ab+ distance BC<br>Fromabovewe¬etˆ−−→talpath≤n>h(AB+BC)isgreaterthanmagnitudeofdispacement(AC).<br>Ifthereisamotionofthepartic≤∈o≠dimensioni.e.alongastraightl∈e,thenthemagnitudeofdisplacementbeconmesequal→→talpath≤n>htraversedbythepartic≤∈thegiventime.<br>(b)Magnitudeofavera≥velocity=magnitudeofdisplacmenttime∫erval=ACt
and Acera≥speed =(total pathe length )/( time interval ) = (AB+BC) /t
As, (AB+ BC) gt AC, so average speed is greater than the magnitude of average velocity. If the particle is moving along a straight line, then in a given time the magniude of displacemnt is equal to total path length traversed by particle in that time, so average speed is equal to magnitude of average velocity.
Topper's Solved these Questions
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - Additional Exercises
13 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - Motion
25 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - Advanced Problems
20 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions
19 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
Similar Questions
Prove that average velocity of an object can never be greater than the average speed of an object over a given interval of time.
View SolutionShow that avertage velocity of the object over an interval of time is either smaller than or equal to the average speed of the object over the same interval.
View Solution
Knowledge Check
The magintude of displacemnt is equal to the distance coverd in a given interval of time if the particle .
AMoves with constant acceleration along any pathBMoves with constant speedCMoves in same direction with constant velocity or with variable velocity.DMoves with constant velocityIt magnitude of average speed and average velocity over an interval of time are same, then
AParticle must move with zero accelerationBParticle must move with uniform accelerationCParticle must be at restDParticle must move in a straight line without turning backThe magnitude of average velocity is equal to the average speed when a particle moves:-
Aon a curved pathBin the same directionCWith constant accelerationDwith constant retardation
Find average speed in time interval t= 2 to t=10s
View SolutionIf magnitude of average speed and average velocity over a time interval are same, then
View SolutionMagnitude of average velocity and speed are found to be the saame in an interval of time.
View SolutionA : The magntidue of average velocity in an interval can never be greater than average speed in that interval.
R : For a moving object distance traveled ≥|Displacement|
View SolutionThe area under velocity-time graph for a particle in a given interval of time represents
View Solution
