A cord is wound round the circumference of wheel of radius r. The axis of the wheel is horizontal and fixed and moment of inertia about it is I. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h, the angular velocity of the wheel will be.
√2ghI+mr
[2mghI+mr2]12
[2mghI+2m]12
√2gh
The correct Answer is:B
To solve the problem, we will use the principle of conservation of energy. The potential energy lost by the falling mass will be equal to the kinetic energy gained by both the mass and the rotating wheel.
1. Identify the Initial and Final Energies:
- Initially, the mass m has potential energy given by:
PEinitial=mgh
- The mass starts from rest, so its initial kinetic energy is:
KEinitial=0
2. Determine the Final Energies:
- After falling a distance h, the potential energy becomes:
PEfinal=0
- The kinetic energy of the mass as it falls is:
KEmass=12mv2
- The kinetic energy of the wheel (rotational kinetic energy) is:
KEwheel=12Iω2
3. Apply Conservation of Energy:
- According to the conservation of energy:
PEinitial=KEmass+KEwheel
- Substituting the expressions we have:
mgh=12mv2+12Iω2
4. Relate Linear and Angular Velocity:
- The linear velocity v of the falling mass is related to the angular velocity ω of the wheel by the equation:
v=rω
- Therefore, we can express ω in terms of v:
ω=vr
5. Substitute ω into the Energy Equation:
- Substitute ω into the energy equation:
mgh=12mv2+12I(vr)2
- This simplifies to:
mgh=12mv2+12Iv2r2
6. Factor Out v2:
- Factor out v2:
mgh=12v2(m+Ir2)
7. Solve for v2:
- Rearranging gives:
v2=2mghm+Ir2
8. Find ω:
- Now, substituting back to find ω:
ω=vr=1r
⎷2mghm+Ir2
Final Result:
Thus, the angular velocity ω of the wheel after the mass has fallen through a distance h is given by:
ω=1r
⎷2mghm+Ir2
- Initially, the mass m has potential energy given by:
PEinitial=mgh
- The mass starts from rest, so its initial kinetic energy is:
KEinitial=0
- After falling a distance h, the potential energy becomes:
PEfinal=0
- The kinetic energy of the mass as it falls is:
KEmass=12mv2
- The kinetic energy of the wheel (rotational kinetic energy) is:
KEwheel=12Iω2
- According to the conservation of energy:
PEinitial=KEmass+KEwheel
- Substituting the expressions we have:
mgh=12mv2+12Iω2
- The linear velocity v of the falling mass is related to the angular velocity ω of the wheel by the equation:
v=rω
- Therefore, we can express ω in terms of v:
ω=vr
- Substitute ω into the energy equation:
mgh=12mv2+12I(vr)2
- This simplifies to:
mgh=12mv2+12Iv2r2
- Factor out v2:
mgh=12v2(m+Ir2)
- Rearranging gives:
v2=2mghm+Ir2
- Now, substituting back to find ω:
ω=vr=1r
⎷2mghm+Ir2
Thus, the angular velocity ω of the wheel after the mass has fallen through a distance h is given by:
ω=1r
⎷2mghm+Ir2
Topper's Solved these Questions
Similar Questions
A cord is wound around the circumference of a wheel of diameter 0.3 m. The axis of the wheel is horizontal. A mass of 0.5 kg is attached at the end of the cord and it is allowed to fall from rest. If the weight falls 1.5 m in 4s, what is the angular acceleration of the wheel ? Also find out the moment of inertia of the wheel.
View SolutionA cord is wound around the circumference of a bicycle wheel (without tyre) of diameter 1m. A mass of 2kg is tied at the end of the cord and it is allowed to fall from rest. The weight falls 2m in 4s. The axle of the wheel is horizontal and the wheel rotates which its plane vertical. if g=10ms−2, what is the angular acceleration of the wheel ?
View Solution
Knowledge Check
- Question 1 - Select One
A cord is wound round the circumference of wheel of radius r. The axis of the wheel is horizontal and fixed and moment of inertia about it is I. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h, the angular velocity of the wheel will be.
A√2ghI+mrB√2mghI+mr2C√2mghI+2mr2D√2gh - Question 2 - Select One
A cord is wound around the circumference of wheel of radius r the axis of the wheel is horizontal and moment of inerita about it is / The weight mg is attached to the end of the cord and falls from rest after falling throgh a distance h the angular velocity of the wheel will be
A[mgh]1/2B[2mghI+2mr2]1/2C[2mghI+mr2]1/2D[mghI+2mr2]1/2 - Question 3 - Select One
A cord is wound round the circumference of wheel of radius r. The axis of the wheel is horizontal and the moment of inertia about it is I. A weight mg is attached to the cord at the end. The weight falls from rest. After falling through a distance 'h', the square of angular velocity of wheel will be :-
A2mghI+2mr2B2mghI+mr2C2ghD2ghI+mr2
A cord of negligible mass is wound around the rim of a wheel of radius 20 cm. An object of mass 600 g is attached to the end of the cord and is allowed to fall from rest. It took 5 s for the object to fall by 2 m. Calculate the angular acceleration and moment of inertia of the wheel. Assume that the axis of wheel is horizontal.
View SolutionA cord is wound around the circumference of wheel of radius r. The axis of the wheel is horizontal and MI is I. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h, the angular velocity of the wheel will be
View SolutionA cord is wound round the circumference of a wheel of radius r. The axis of the wheel is horizontal and its moment of inertia about this axis is I. A weight mg is attached to the end of the cord and is allowed to fall from rest. The angular velocity of the wheel, when the weight has fallen through a distance h, is
View SolutionA cord is wound round the circumference of a solid cylinder radius R and mass M. The axis of the cylinder is horizontal. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h.
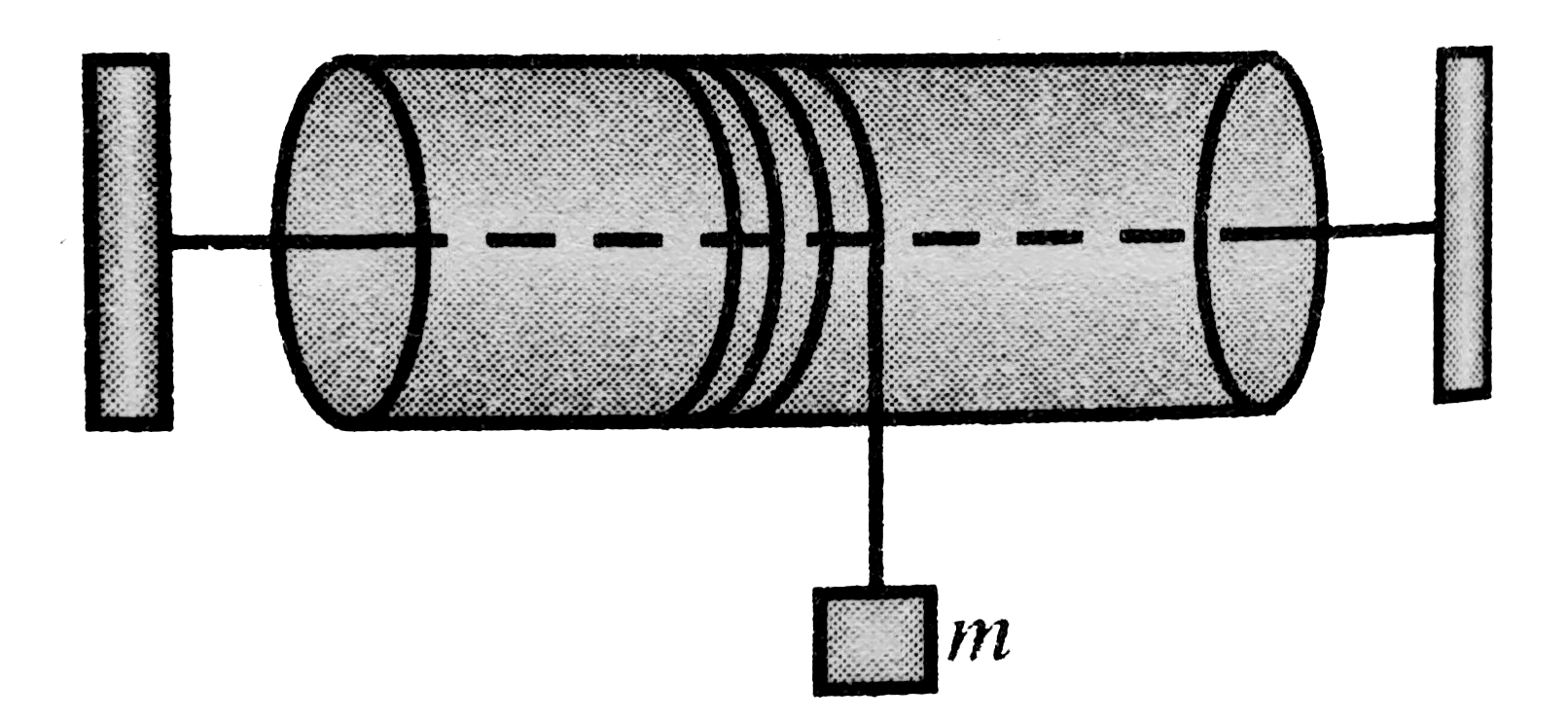
View SolutionA cord is wound round the circumference of a solid cylinder radius R and mass M. The axis of the cylinder is horizontal. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h.
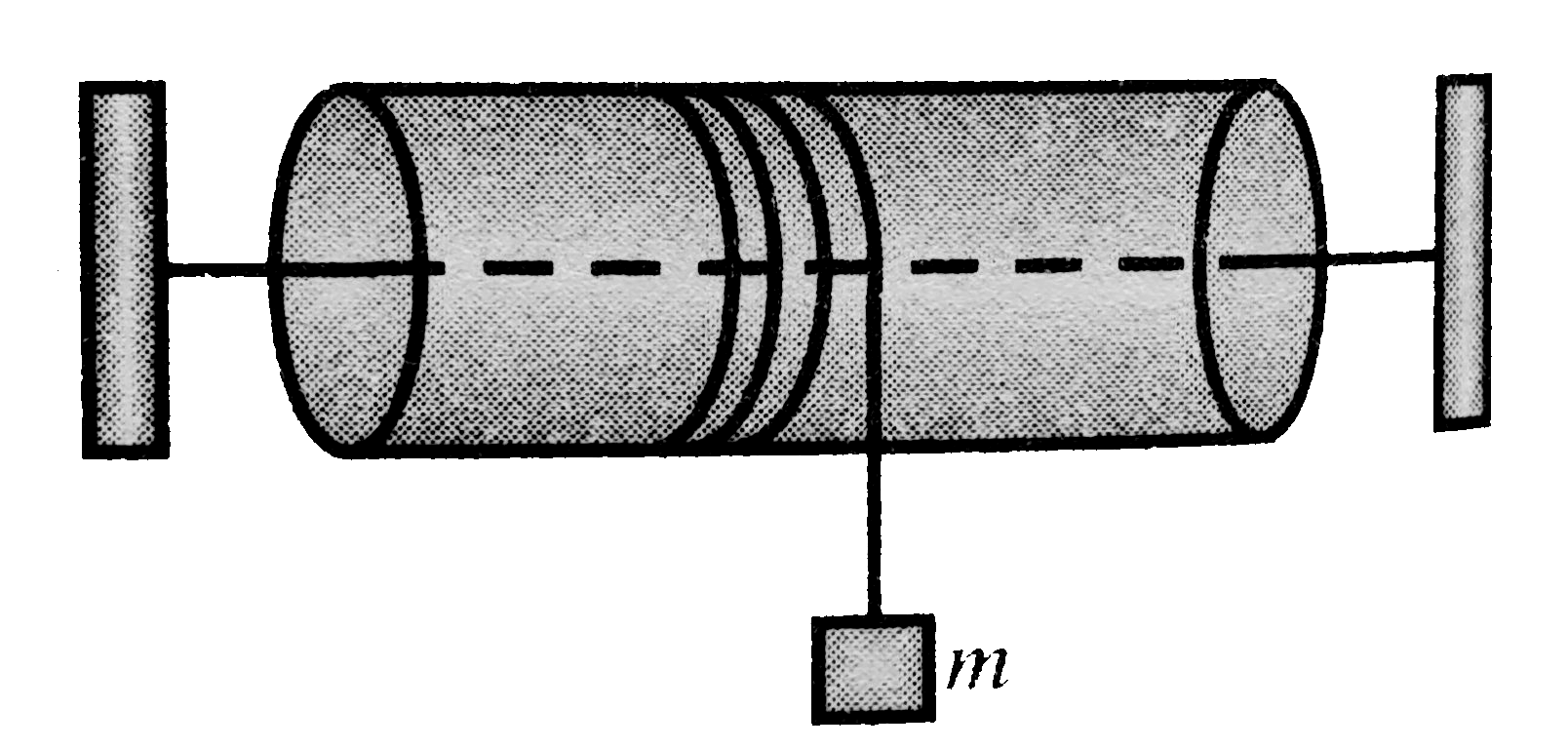
If the mass starts from rest and falls a distance h, then its speed at that instant is:View Solution

