The top in figure has a moment of inertia of and is initially at rest. It is free to rotate about the stationary axis . A string, wrapped around a peg along the axis of the top, is pulled in such a manner as to maintain a constant tension of .If the string does not slip while it is unwound from the peg. What the angular speed of the top after of string has been pulled off the peg ?
 .
.
The correct Answer is:D
(d) For the non-isolated system of the top,
.
Topper's Solved these Questions
- ROTATIONAL DYNAMICSBOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Rotation And Translation Combined And Rolling Motion
18 Videos
- ROTATIONAL DYNAMICSBOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Problems Based On Mixed Concepts
31 Videos
- ROTATIONAL DYNAMICSBOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Angular Momentum And Its Conservation
30 Videos
- PROPERTIES OF MATTERBOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test
29 Videos
- THERMAL PROPERTIES OF MATTERBOOK - A2ZCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Chapter Test
30 Videos
Similar Questions
The top is figure has moment of inertia equal to 4.00×104kgm2 and is initially at rest. It is free to rotate about the stationary axis AA'. A string wrapped around a ped alonng the axis of the top is pulled in such a manners as to maintain a constant tension of 5.57N.If the string does not slip while it is unwound from the peg, what is the angular speed of the top after 80.0CM of string has been pulled of the peg?

View SolutionThe toy-top (initially at rest) shown in the figure, has a moment of inertia 4×10−4kg m2 and it is free to rotate about the stationary axis AA'. The string wrapped around the peg of the toy-top is pulled outwards without letting it slip and simultaneously maintaining a constant tension of 5.625N. If ω is the angular speed (in rad s−1) of the top after 80 cm of string has been pulled of the peg, then mark your answer as ω100.

View Solution
Knowledge Check
A flywheel of moment of inertia 0.4 kg m2 and radius 0.2 m is free to rotate about a central axis. If a string is wrapped around it and it is pulled with a force of 10N. Then its angular velocity after 4s will be
A10rads−1B5rads−1C20rads−1DNoneA uniform cylinder free to turn around a fixed axis has a string wrapped around it as shown. The string is pulled with a force F equal to the cylinders weight. What is the acceleration of the string?
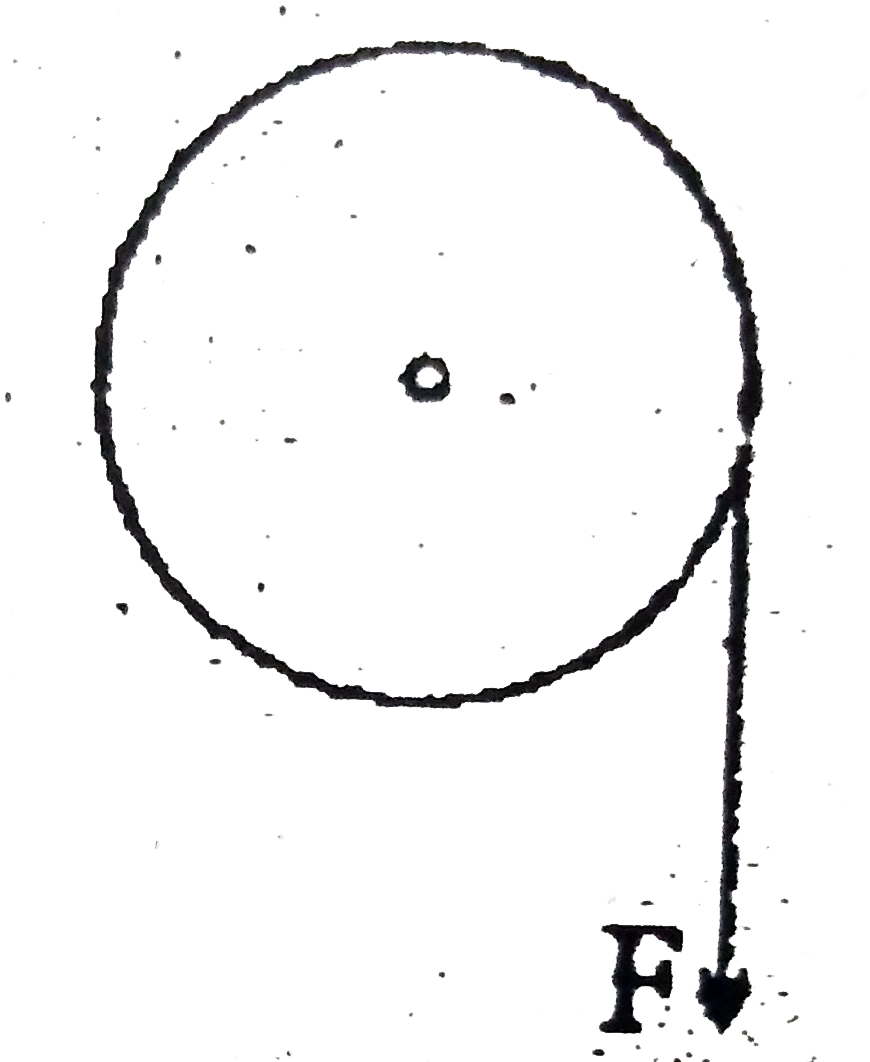 A2gBgCg/2Dg/4
A2gBgCg/2Dg/4A string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
AMg6BMg3CMg2D2Mg3
Solve the previous problem if the pulley has a moment of inertia I about its axis and the string does not slip over it.
View SolutionMoment of inertia of a body about an axis is 4kg−m2. The body is initially at rest and a torque of 8Nm starts acting on it along the same axis. Workdone by the torque in 20s, in joules is
View SolutionThe pulley shown in figure has a moment of inertia I about it's axis and its radius is R. Find the magnitude of the acceleration of the two blocks. Assume that the string is light and does not slip on the pulley.
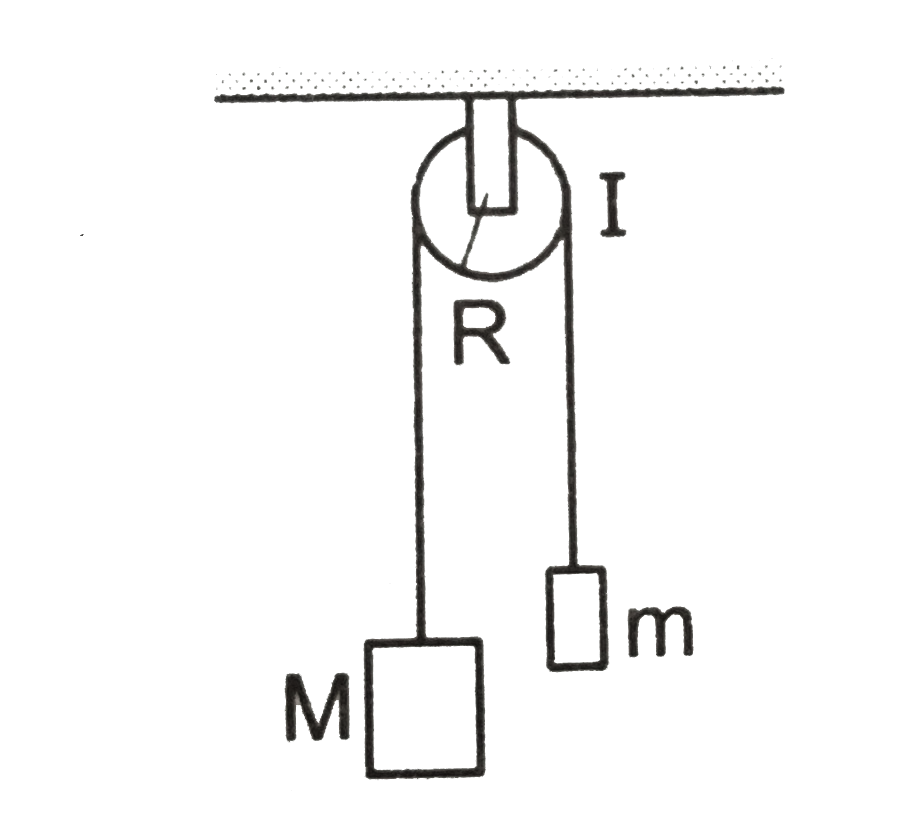
View SolutionA solid cylinder of mass 50 kg and radius 0.5 m is free to rotate about the horizontal axis . A massless string is wound around the cylinder with one end attached to it and other hanging freely . Tension in the string required to produce an angular acceleration of 2 revolutions s−2 is
View SolutionA string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
View Solution
