A small block slides with velocity 0.5√gr on the horizontal frictionless surface as shown in the figure. The block leaves the surface at point C . Calculate angle θ in the figure.
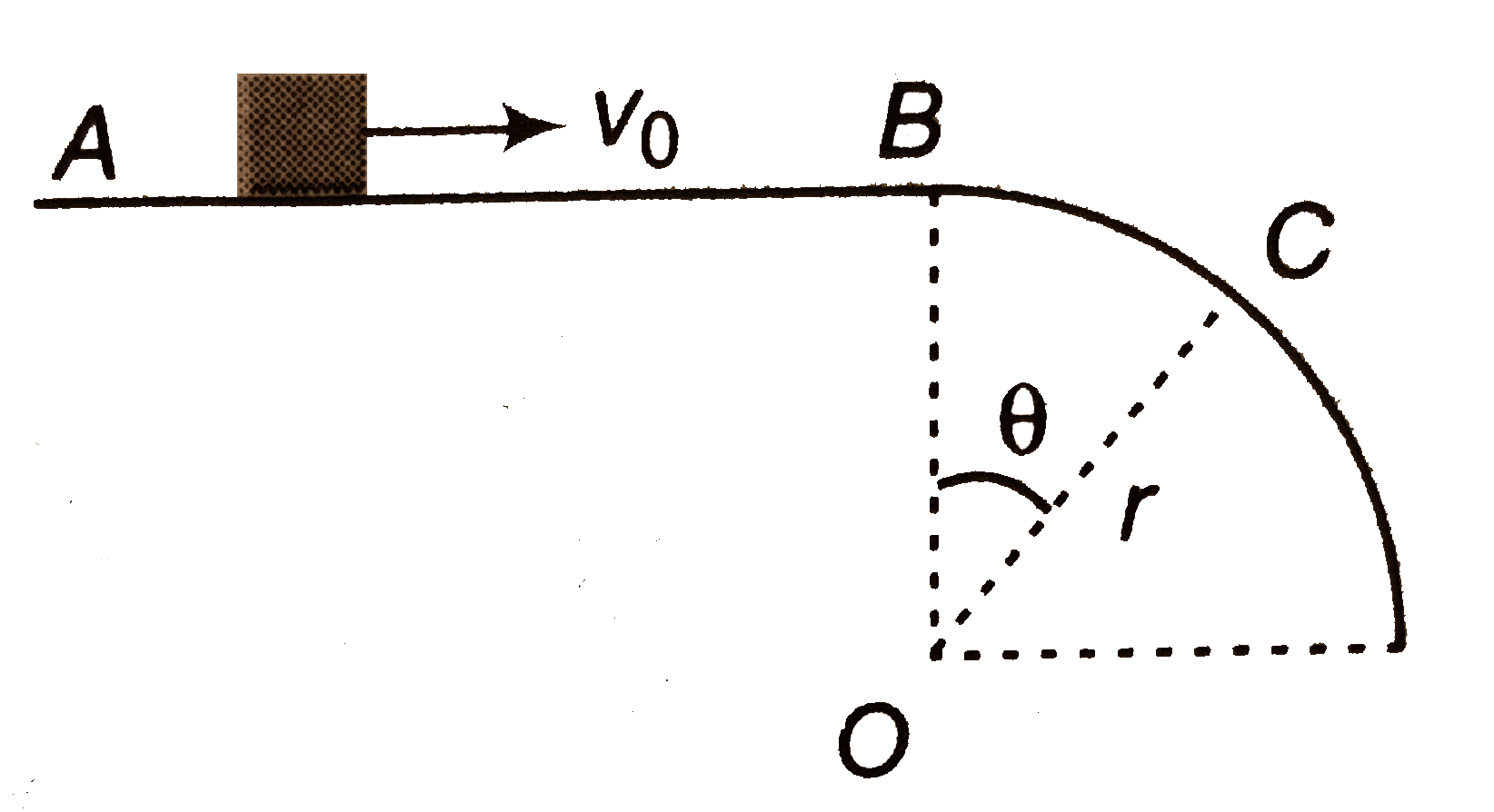
cos−1.49
cos−1.34
cos−1.14
cos−1.45
The correct Answer is:B
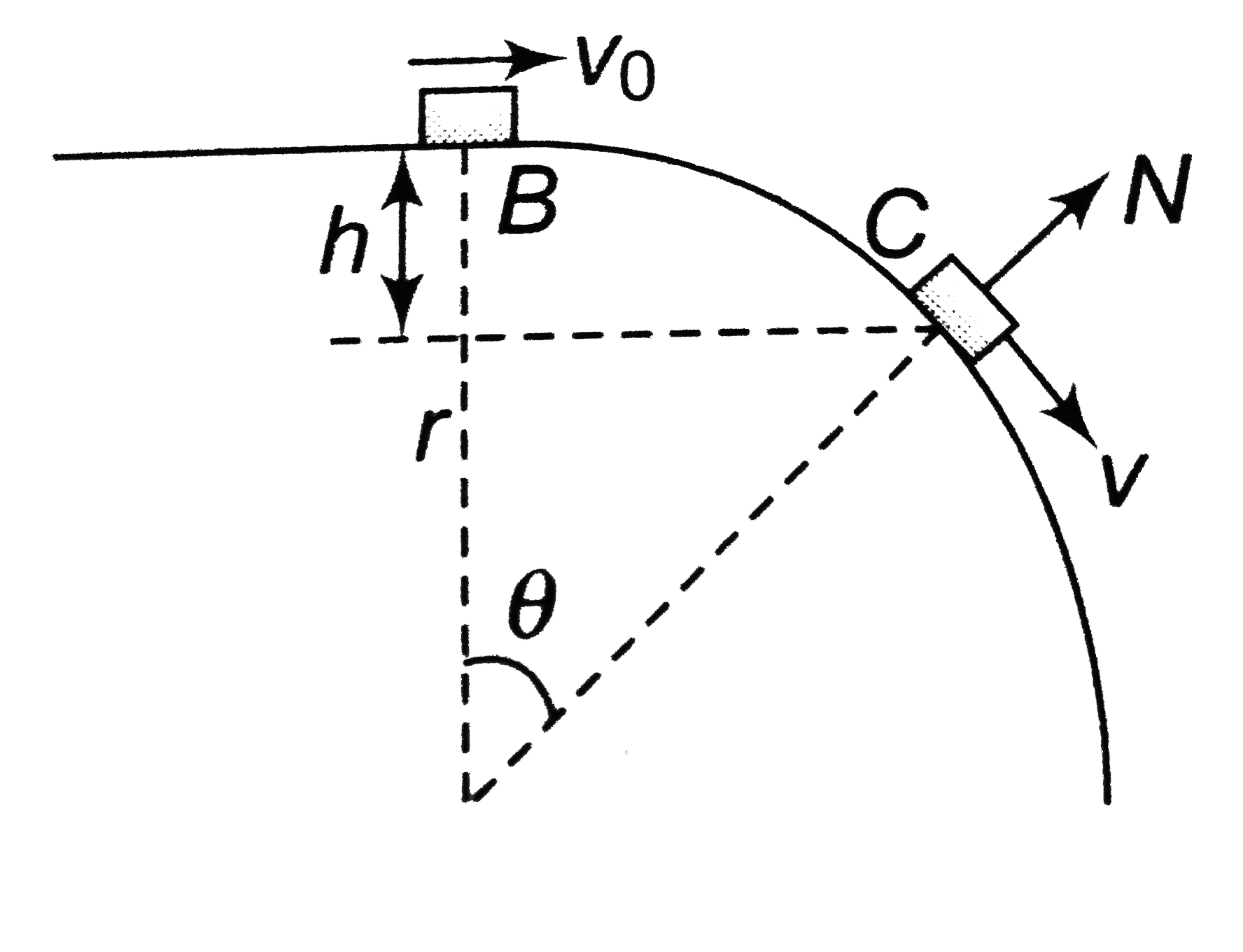
At point Cmgcosθ−N=mv2r
(when block leavels the surface normal force becomes zero, so putting N=0)⇒gcosθ=v2r
v2=v20+2gh,h=r−rcosθ
gr cos theta = (0.5 sqrtgr)^(2) + 2g (r - r cos theta)
⇒cosθ=14+2−2cosθ⇒cosθ=34
Topper's Solved these Questions
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Collision
31 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Problems Based On Mixed Concepts
32 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Mechanical Power
25 Videos
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Chapter Test
30 Videos
Similar Questions
A small block slides with velocity v0=0.5√gr on the horizontal frictionless surface as shown in the figure. The block leaves the surface at point C. The angle θ in the figure is cos−1(x)y. Find (x+y).
'(##CEN_KSR_PHY_JEE_C07_032_Q21##)'
View Solution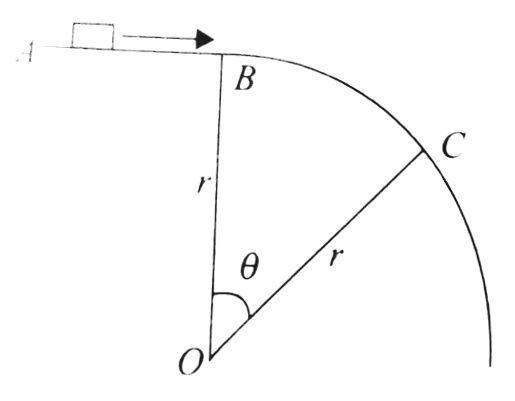
A small block slides with velocity v0=0.5√gr on the horizontal frictiohnless surface as shown in the fig. the block leaves the surface at point C. The angle θ in the figure is:
View Solution
Knowledge Check
A block of mass √3kg rests on a horizontal frictionless surface. What will be the acceleration of the block if it is subjected to two forces as shown in the figure.
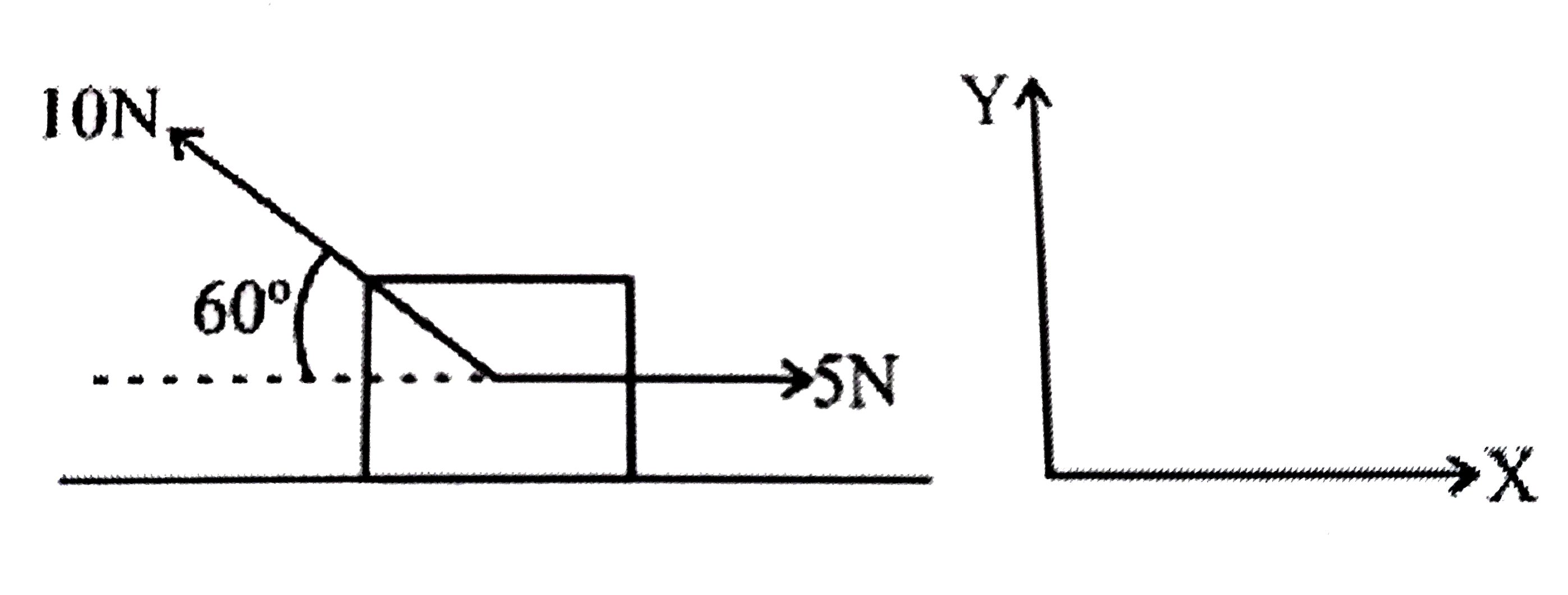 AAcceleration will be along Y-axisBAcceleration will be along X-axisCAcceleration will be in between X & YDNo acceleration will be there
AAcceleration will be along Y-axisBAcceleration will be along X-axisCAcceleration will be in between X & YDNo acceleration will be thereA block of mass 2 m is pulled along a horizontal frictionless surface by a rope of mass m as shown in the figure given. What force is exerted by the rope on the block ?
 AF3BF2C2F3DF
AF3BF2C2F3DFTwo blocks rest on a horizontal frictionless surface as shown in the figure. The surface between the top and bottom blocks is roughened so that there is no slipping between the two blocks. A 30-N force is applied to the bottom block as suggested in the figure.
 AWhat is the minimum coefficient of static friction necessary to keep the top block from slipping on the bottom block?B0.05C0.20D0.10
AWhat is the minimum coefficient of static friction necessary to keep the top block from slipping on the bottom block?B0.05C0.20D0.10
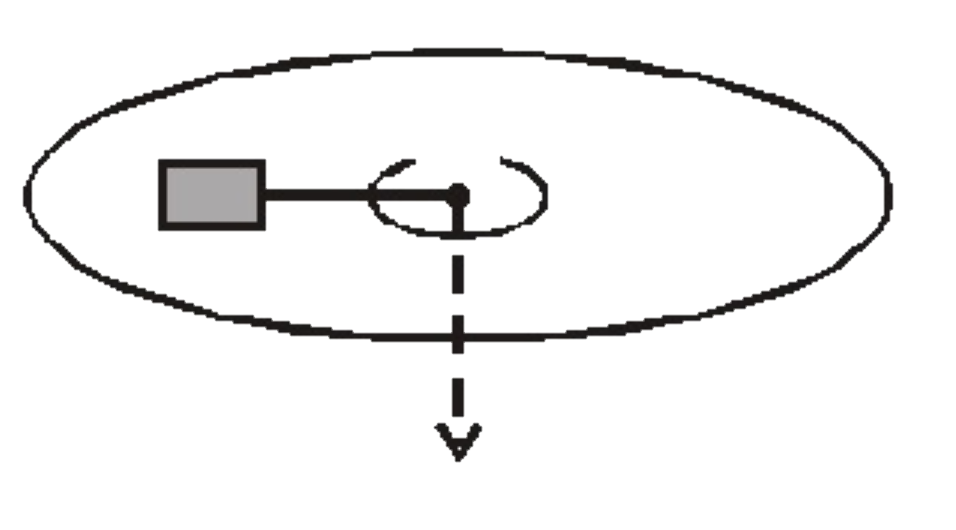
A block of mass 0.1 kg is attached to a cord passing through a hole in a horizontal frictionless horizontal surface as shown in the figure. The block is originally revolving at a distance of 0.4 m from the hole, with an angular velocity of 2 rad s−1. The cord is then pulled from below, shortening the radius of the circle in which the block revolves to 0.2 m. Considering the block to be point mass, calculate the new angular velocity (in rad s−1)
View Solution
A block of mass 2 m is pulled along a horizontal frictionless surface by a rope of mass m as shown in the figure given above. What force is exerted by the rope on the block ?
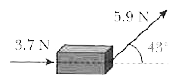
View SolutionTwo forces act on a 4.5 kg block resting on a frictionless surface as shown in the given figure. What is the magnitude of the horizontal acceleration of the block ?
View SolutionTwo blocks rest on a horizontal frictionless surface as shown in the figure. The surface between the top and bottom blocks is roughened so that there is no slipping between the two blocks. A 30-N force is applied to the bottom block as suggested in the figure.
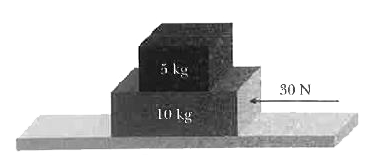
What is the magnitude of the force of static friction between the top and bottom blocks?
View Solution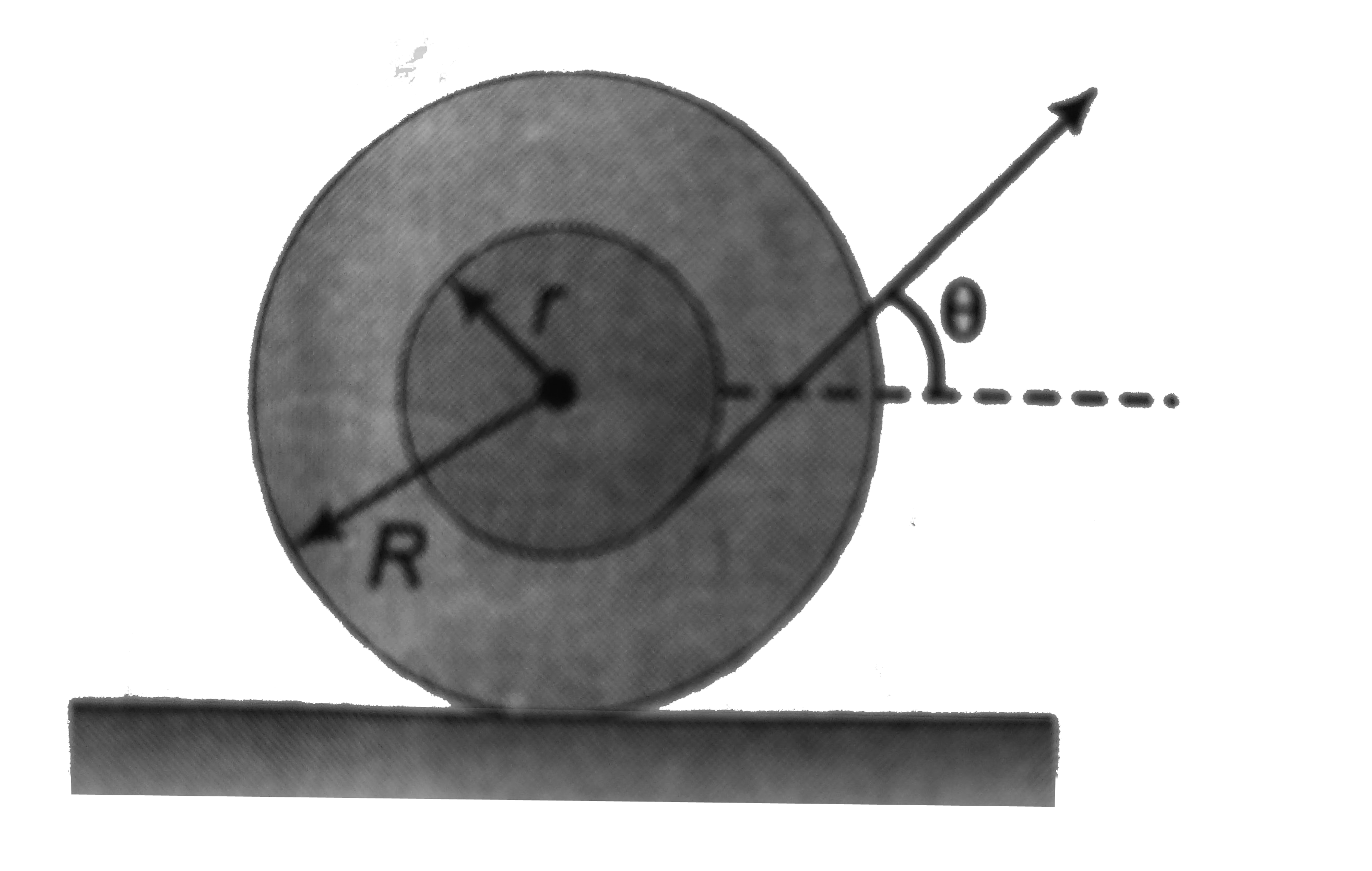
A spool is pulled at an angle θ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle θ is equal to
View Solution
