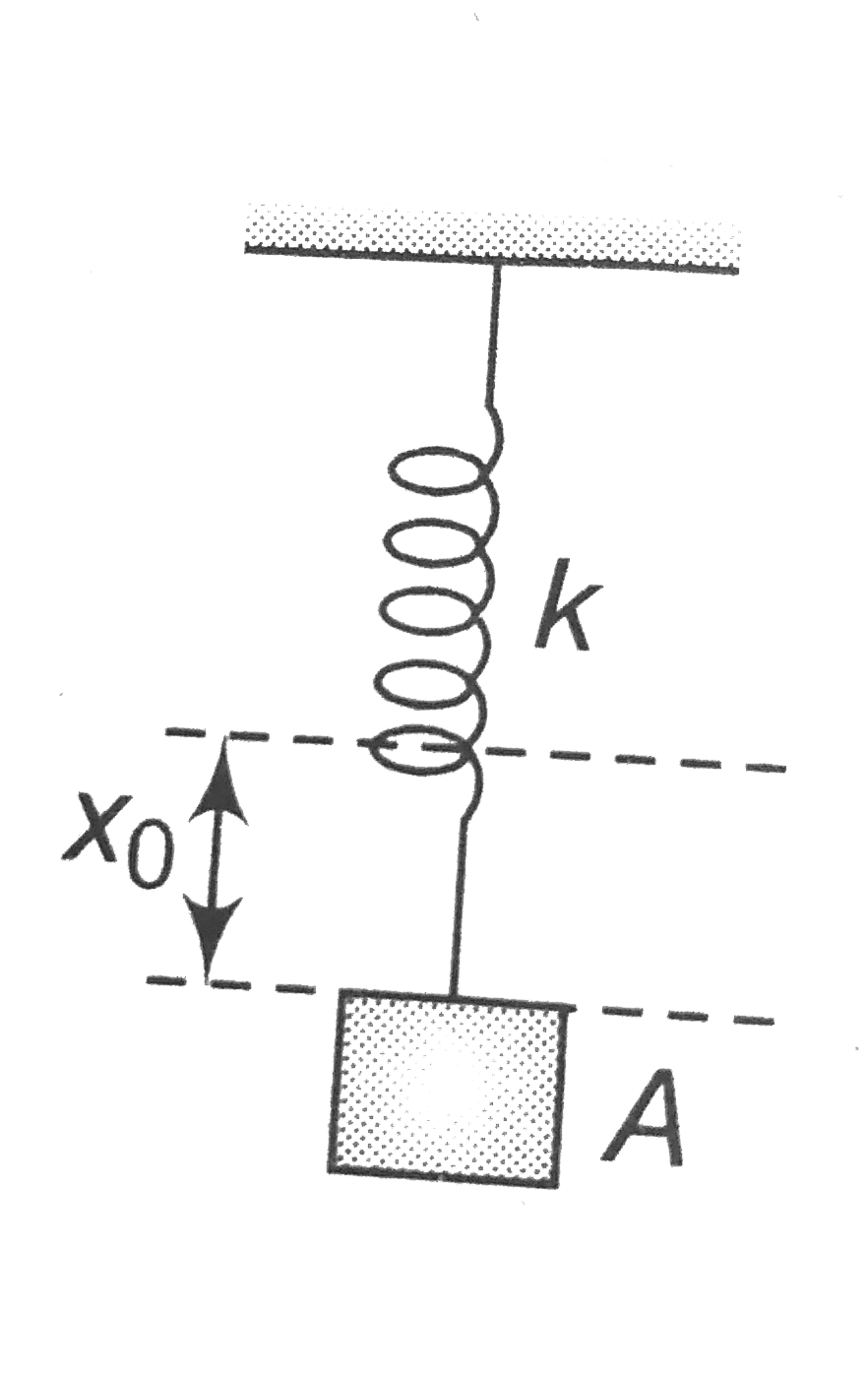
Block A of the mass M in the figure is released from rest when the extension in the spring is x0. The maximum downwards displacement of the block is (assume x0<Mg/k).
2(Mgk−x0)
Mg2k+x0
2Mgk−x0
2Mgk+x0
The correct Answer is:A
Loss in gravitational potential energy of block is equal to gain in elastic potential energy of spring
∴Mgx=12k(x+x0)2−12kx20
On solving x=2(Mgk−x0)
Topper's Solved these Questions
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Mechanical Power
25 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Circular Motion In Vertical Plane
21 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Potential Energy, Conservative And Non-Conservative Forces
28 Videos
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Chapter Test
30 Videos
Similar Questions
In figure, the stiffness of the spring is k and mass of the block is m. The pulley is fixed. Initially, the block m is held such that the elongation in the spring is zero and then released from rest. Find:
a. the maximum elongation in the spring.
b. the maximum speed of the block m. Neglect the mass of the spring and that of the string. Also neglect the friction.
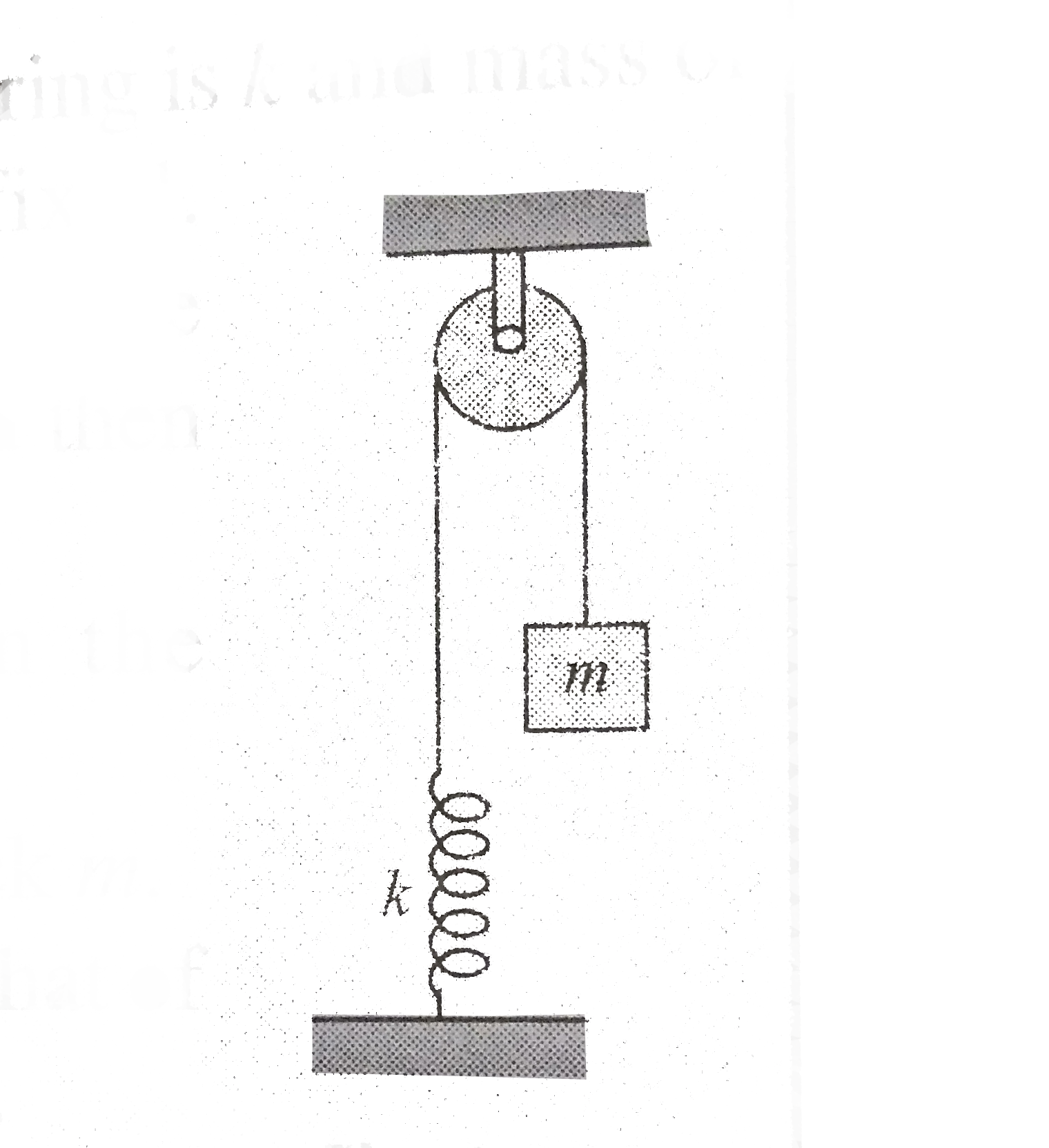
View SolutionThe block shown in figure is released from rest. Find out the speed of the block when the spring is compressed by 1 m
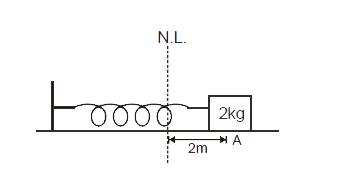
View Solution
Knowledge Check
Block A in the figure is released from the rest when the extension in the spring is x0. The maximum downward displacement of the block will be :
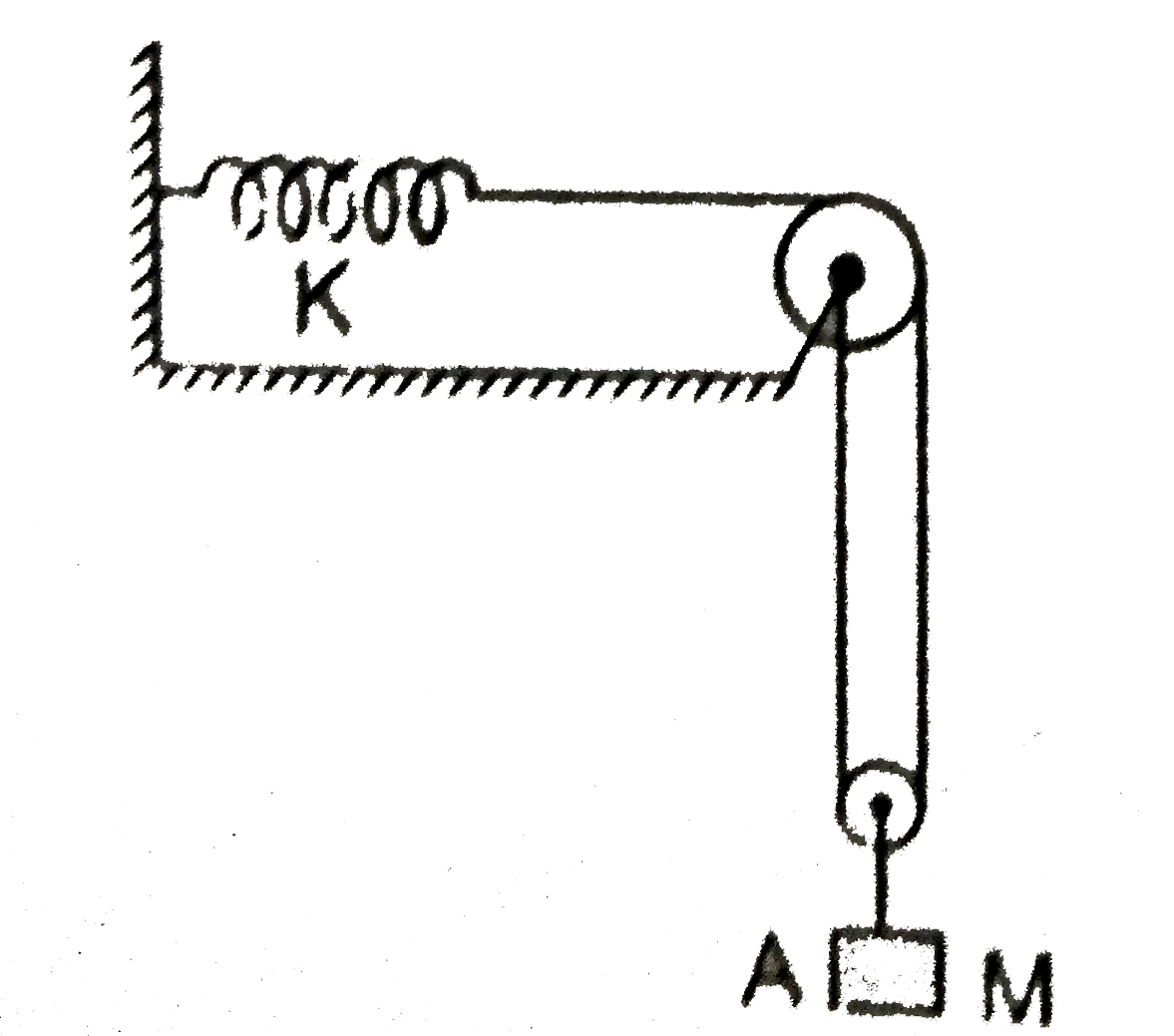 AMg/2k−x0BMg/2k+x0C2Mg/k−x0D2Mg/k+x0
AMg/2k−x0BMg/2k+x0C2Mg/k−x0D2Mg/k+x0Block A in the figure is released from rest when the extension in the spring is x0(x0<mg/k). The maximum downward displacement of the block is (there is no friction) :
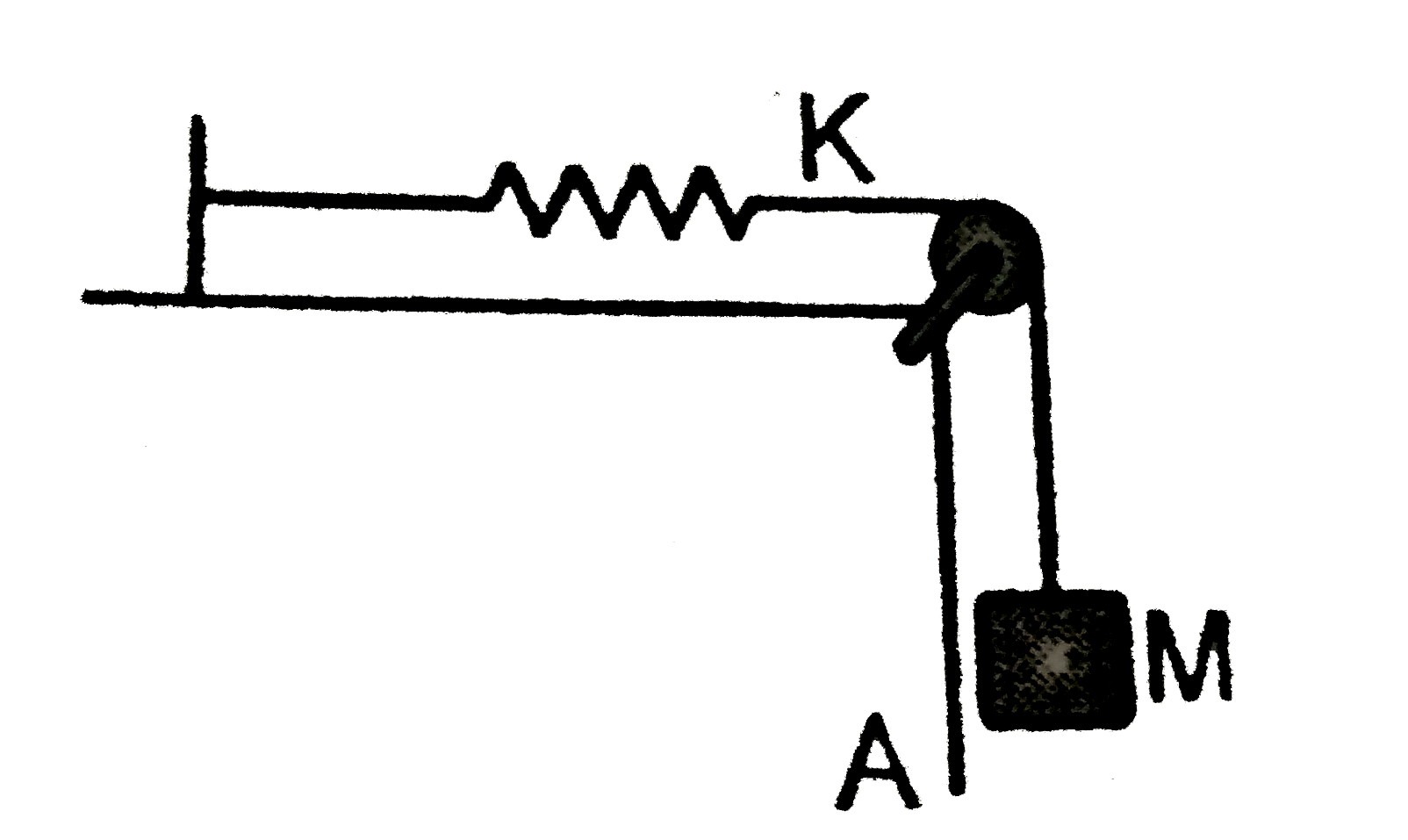 A2MgK−2x0BMg2K+x0C2MgK−x0D2MgK+x0
A2MgK−2x0BMg2K+x0C2MgK−x0D2MgK+x0In above question, speed of block A, when the extension in spring is xm2, is:
A2g√mkB2g√2mkC2g√2m3kDg√4m3k
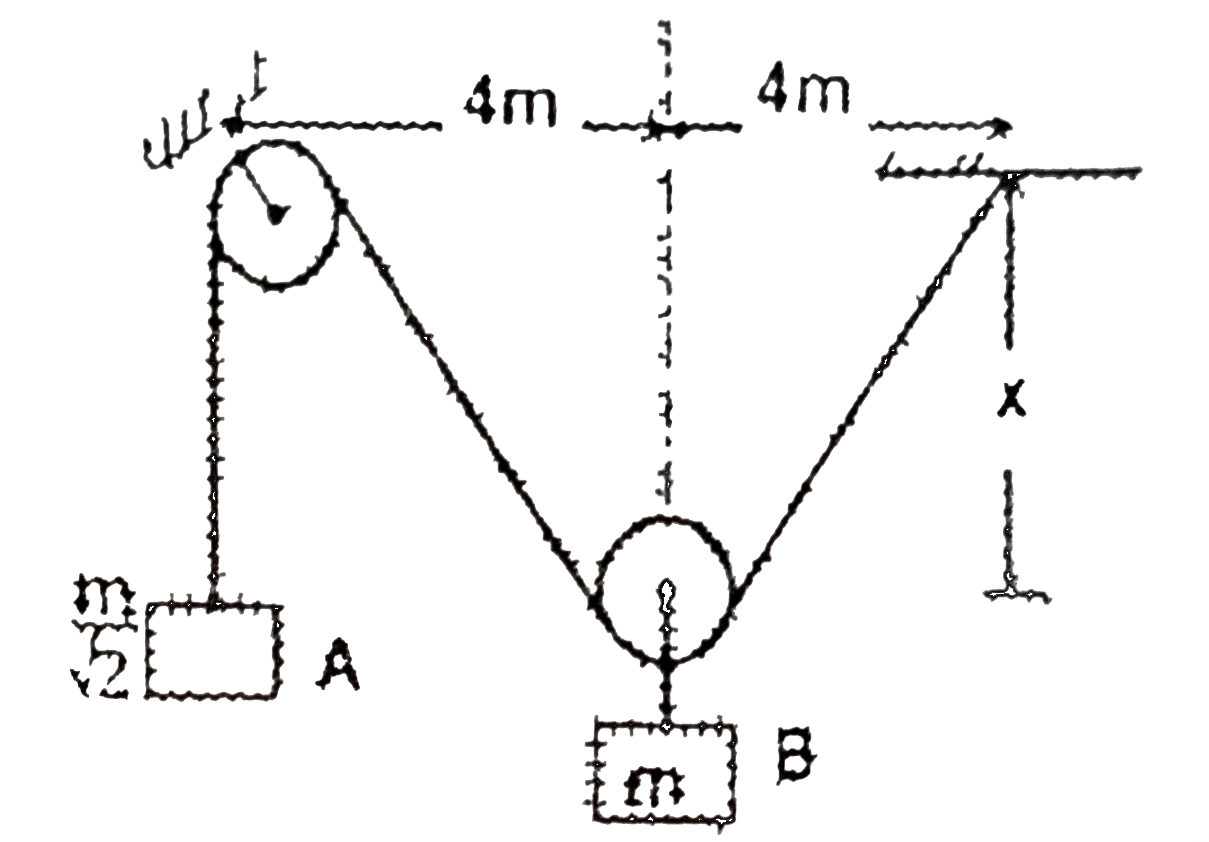
The system of mass A and B shown in the figure is released from rest with x=0, determine the velocity of mass B when x=3m. Also find the maximum displacement of mass B.
View SolutionTwo blocks each of mass m, connected by an un-stretched spring are kept at rest on a frictionless horizontal surface. A constant force F is applied on one of the blocks pulling it away from the other as shown in figure.
 (a)Find accelaration of the mass center.
(a)Find accelaration of the mass center.
(b) Find the displacement of the centre of mass as function of time t.
(c) If the extension of the spring is X0 at an instant t, find the displacements of the two blocks relative to the ground at this instant.
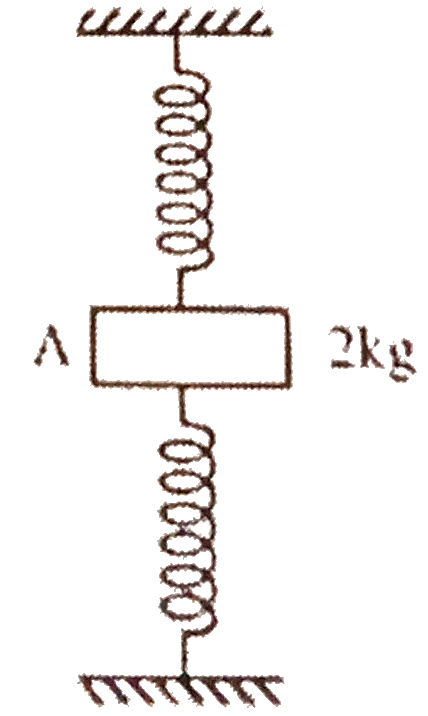
View SolutionA block A of mass 2 kg is connected with two springs, as shown . The spring constant of lower spring is system is thrice the spring costant of upper spring. The system is released from rest with both the springs unstreched. The maximum displacement of bloack is 0.1m. Find the acceleration of the block is its lowest position-
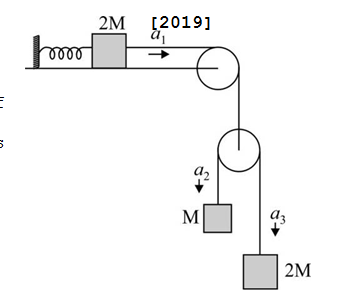
View SolutionA block of mass 2M is attached to a massless spring with spring-constant k. This block is connected to two other blocks of masses M and 2M using two massless pulleys and strings. The accelerations of the blocks are a1a2 and a3 as shown in the figure. The system is released from rest with the spring in its unstretched state. The maximum extension of the spring is x0 Which of the following option(s) is(are) correct? [g is the acceleration due to gravity. Neglect friction]
View SolutionTwo blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

If the extension of the spring is x0 at time t, then the displacement of the first block at this instant is
View Solution
