Question

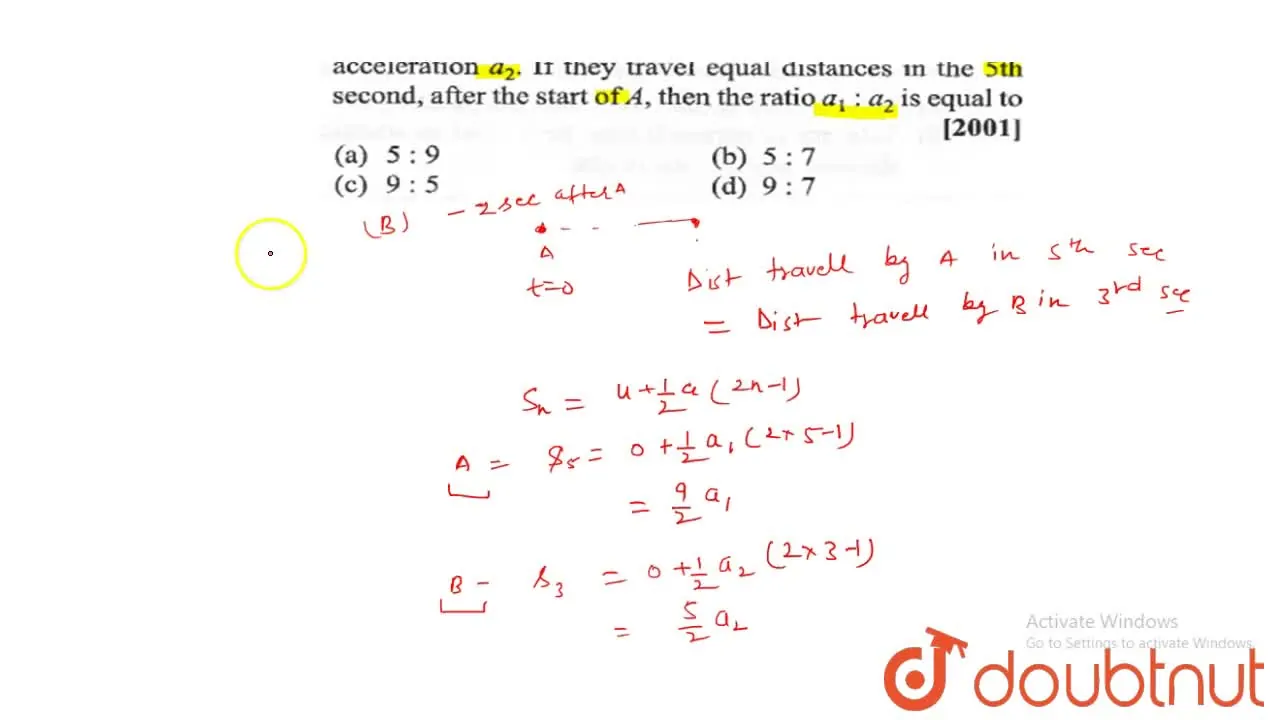
A body $A$ starts from rest with an acceleration $a_1$. After $2$ seconds, another body $B$ starts from rest with an acceleration $a_2$. If they travel equal distances in the $5th$ second, after the start of $A$, then the ratio $a_1:a_2$ is equal to :

Options

A. `5 : 9`
B. `5 : 7`
C. `9 : 5`
D. `9 : 7`

Answer

A

Explanation

To solve the problem, we need to find the ratio of accelerations $$a_1$$ and $$a_2$$ for two bodies $$A$$ and $$B$$ that travel equal distances during specific time intervals. Here’s a step-by-step solution:

Step 1: Understand the Motion of Body A
Body \( A \) starts from rest with an acceleration \( a_1 \). The distance traveled by body \( A \) in the \( n \)-th second can be calculated using the formula:
\( S_n = u + \frac{1}{2} a t^2 \)
Since \( A \) starts from rest, \( u = 0 \). The distance traveled in the \( 5^{th} \) second is given by:
\( S_5 = 0 + \frac{1}{2} a_1 (2 \cdot 5 - 1) = \frac{1}{2} a_1 (10 - 1) = \frac{9}{2} a_1 \)

Step 2: Understand the Motion of Body B
Body \( B \) starts from rest with an acceleration \( a_2 \) but starts 2 seconds after body \( A \). Therefore, when \( A \) has traveled for 5 seconds, \( B \) has only traveled for 3 seconds. The distance traveled by body \( B \) in the \( 3^{rd} \) second can be calculated using the same formula:
\( S_n = u + \frac{1}{2} a t^2 \)
For body \( B \):
\( S_3 = 0 + \frac{1}{2} a_2 (2 \cdot 3 - 1) = \frac{1}{2} a_2 (6 - 1) = \frac{5}{2} a_2 \)

Step 3: Set the Distances Equal
According to the problem, the distances traveled by both bodies in their respective time intervals are equal:
\( S_5 \text{ (for A)} = S_3 \text{ (for B)} \)
Thus, we have:
\( \frac{9}{2} a_1 = \frac{5}{2} a_2 \)

Step 4: Solve for the Ratio of Accelerations
To find the ratio \( \frac{a_1}{a_2} \), we can rearrange the equation:
\( 9 a_1 = 5 a_2 \)
Dividing both sides by \( 5 a_2 \):
\( \frac{a_1}{a_2} = \frac{5}{9} \)

Conclusion
The ratio of the accelerations \( a_1 : a_2 \) is:
\( \boxed{\frac{5}{9}} \)