Question

Find the area under the curve $y=\cos x$ over the interval
(a) $[0,\frac{\pi }{2}]$
(b) $[0,\pi ]$

Explanation

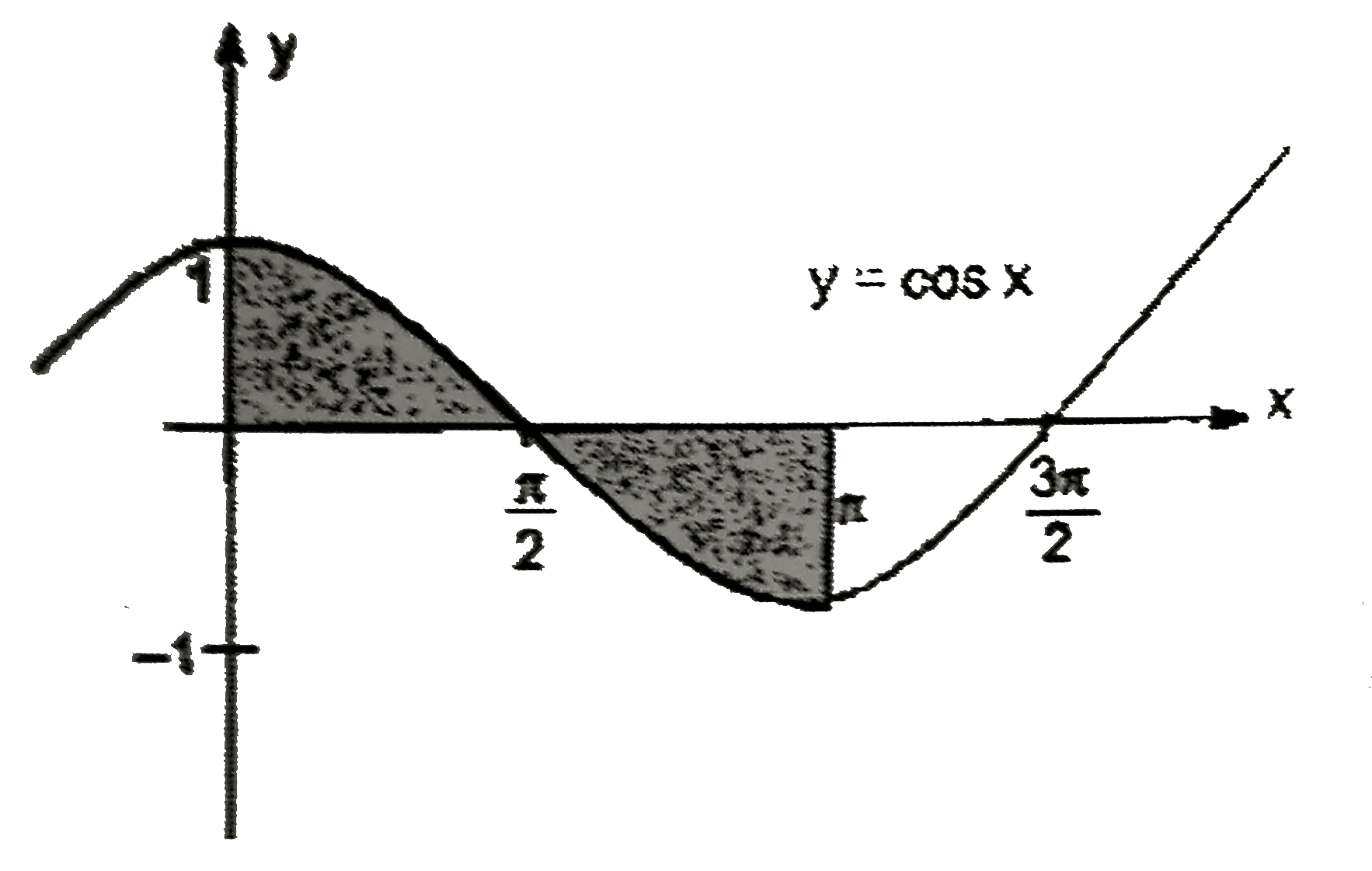
(a). Since `cosxge0` over the interval `[0,(pi)/(2)]` the area A under the curve is
`A=int_(0)^((pi)/(2))cosxdx=[sinx]_0^((pi)/(2))=sin(pi)/(2)-sin0=1`
(b). The given integral can be interpreted as the signed area between the graph of `y=cosx` and the interval `[0,pi]`. The graph in figure suggests that over the interval `[0,pi]` the portion of area above the x-axis is the same as the portion of area below the x-axis so we conjecture that the signed area is zero, this implies that the value of the integral is zero. This is confirmed by the computations.
`int_(0)^(pi)cosxdx=sinx]_(0)^(pi)=sinpi-sin0=0`