What is the period of a pendulum formed by pivoting a meter stick so that is free to rotate about a horizontal axis passing through the 75cm mark?
Let m be the mass and l be the length of the stick
l=100cm
The distance of the point of suspension from center of gravity is d=25cm
Moment of inertia about a horizontal axis through O is
l=lC+md2⇒ml212+md2
⇒T=2π√lmgd=2π
⎷ml212+md2mgd
⇒T=2π√l2+12d212gd=2π√l2+12(0.25)212×9.8×0.25=153s

Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Solved Example
15 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.1
23 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
What is the period of a pendulum formed by pivoting a meter stick so that is free to rotate about a horizontal axis passing through the 75cm mark?
View SolutionA metallic rod of mass m length R is pivoted at O. It is free to rotate about a horizontal axis passing through O . An inward magnetic field is applied as shown in the figure. Find the ratio of maximum electric field inside the metallic rod to the applied magnetic field induction .

View Solution
Knowledge Check
A disc is made to oscillate about a horizontal axis passing through mid point of its radius. Determine time period.
A2π√3R2gB2π√2R3gC2π√RgD2π√2RgA solid cube of side l is made to oscillate about a horizontal axis passing through one of its edges. Its time period will be
A2π√2√23lgB2π√23lgC2π√√32lgD2π√2√3lgA massless stick of length L is hinged at one end and a mass m attached to its other end. The stick is free to rotate in vertical plane about a fixed horizontal axis passing through frictionless hinge. The stick is held in a horizontal position. At what distance x from the hinge should a second mass M = m be attached to the stick, so that stick falls as fast as possible when released from rest
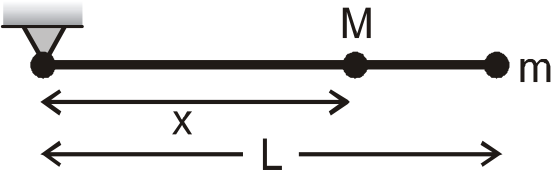 A√2LB√3LC(√2−1)LD(√3−1)L
A√2LB√3LC(√2−1)LD(√3−1)L
Torques of equal magnitude are applied to hollow cylinder and a solid sphere, both having the same mass and same radius. The cylinder is free to rotate aabout its standard axis of symmetry, and the sphere is free to rotate about an axis passing through its centre. which of the two will aquire a greater angular speed after a given time ?
View SolutionTorques of equal magnitude are applied to a hollow cylinder and a solid sphere , both having the same mass and radius . The cylinder is free to rotate about its standard axis of symmetry , and the sphere is free to rotate about an axis passing through its centre . Which of the two will acquire a greater angular speed after a given time .
View SolutionA disc is made to oscillate about a horizontal axis passing through mid point of its radius. Determine time period.
View SolutionA disc of mass M = 2m and radius R is pivoted at its centre. The disc is free to rotate in the vertical plane about its horizontal axis through its centre O. A particle of mass m is stuck on the periphery of the disc. Find the frequency of small oscillations of the system about its equilibrium position.
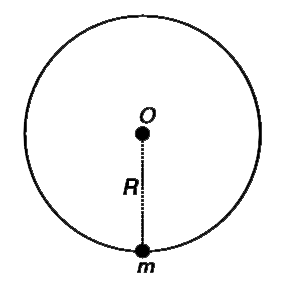
View SolutionA metre scale is suspended vertically from a horizontal axis passing through one end of it. Its time period would be
View Solution