A circular current-carrying coil has a radius R. The distance from the centre of the coil, on the axis, where B will be 1/8 of its value at the centre of the coil is
R/√3
√3R
2R√3
(2√3)R
The correct Answer is:B
(b) Baxis=μ04π×2πlR2(R2+x2)3/2
At centre, Bcentre=μ04π×2πlR
In the given problem,
μ04π×2πlR2(R2+x2)3/2=18[μ04π×2πlR]
or (R2+x2)3/2=8R2
Solving, we get x=R√3
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (multiple Currect )
5 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (assertion-reasioning )
2 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (subjective )
10 Videos
- SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - CENGAGE PHYSICSCHAPTER - SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - QUESTION BANK
12 Videos
- THERMAL PROPERTIES OF MATTERBOOK - CENGAGE PHYSICSCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Bank
40 Videos
Similar Questions
A circular current carrying coil has a radius R. The distance from the centre of the coil on the axis where the magnetic induction will be 18 th to its value at the centre of the coil, is
View SolutionA circular current carrying coil has a radius a. Find the distance from the centre of coil, on its axis, where is the magnetic induction will be 1/6th of its value at the centre of coil.
View Solution
Knowledge Check
A circular current carrying coil has a radius R. The distance from the centre of the coil on the axis where the magnetic induction will be (1/8)th of its value at the centre of the coil, is
AR/√3BR√3C2R/√3D(2R/√3)RThe radius of la circular current carrying coil is R. At what distance from the centre of the coil on its axis, the intensity of magnetic field will be 12√2 times that at the centre?
A2RB3R2CR is doubledDR2Radius of current carrying coil is 'R'. Then ratio of magnetic fields at the centre of the coil to the axial point, which is R√3 distance away from the centre of the coil :-
A1:1B1:2C1:4D8:1
Show that the magnetic field along the axis of a current carrying coil of radius r at a distance x from the centre of the coil is smaller by the fraction 3x2/2r2 than the field at the centre of the coil carrying current.
View SolutionAn electric current is flowing in a circular coil of radius r .If at a distance √Kr from the centre on the axis of the coil the magnetic fleld be 18 th of it's value at the centre,then find the value of K.
View SolutionA closely wound, circular coil with radius 2.40cm has 800 turns.
(a) What must the current in the coil be if the magnetic field at the centre of the coil is0.0580T?
(b) At what distance x from the centre of the coil, on the axis of the coil, is the magnetic field half its value at the centre?
View Solution(a) A circular current carrying coil has a radius R. Find magnetic field (a) at centre and (b) along the axis of coil distant √3R from centre. The coil is having N turns and carriers a current i.
(b) Two concentric coil A and B, having current i and 2i and radii 2R and R are placed as shown. Find magnetic field at common centre.
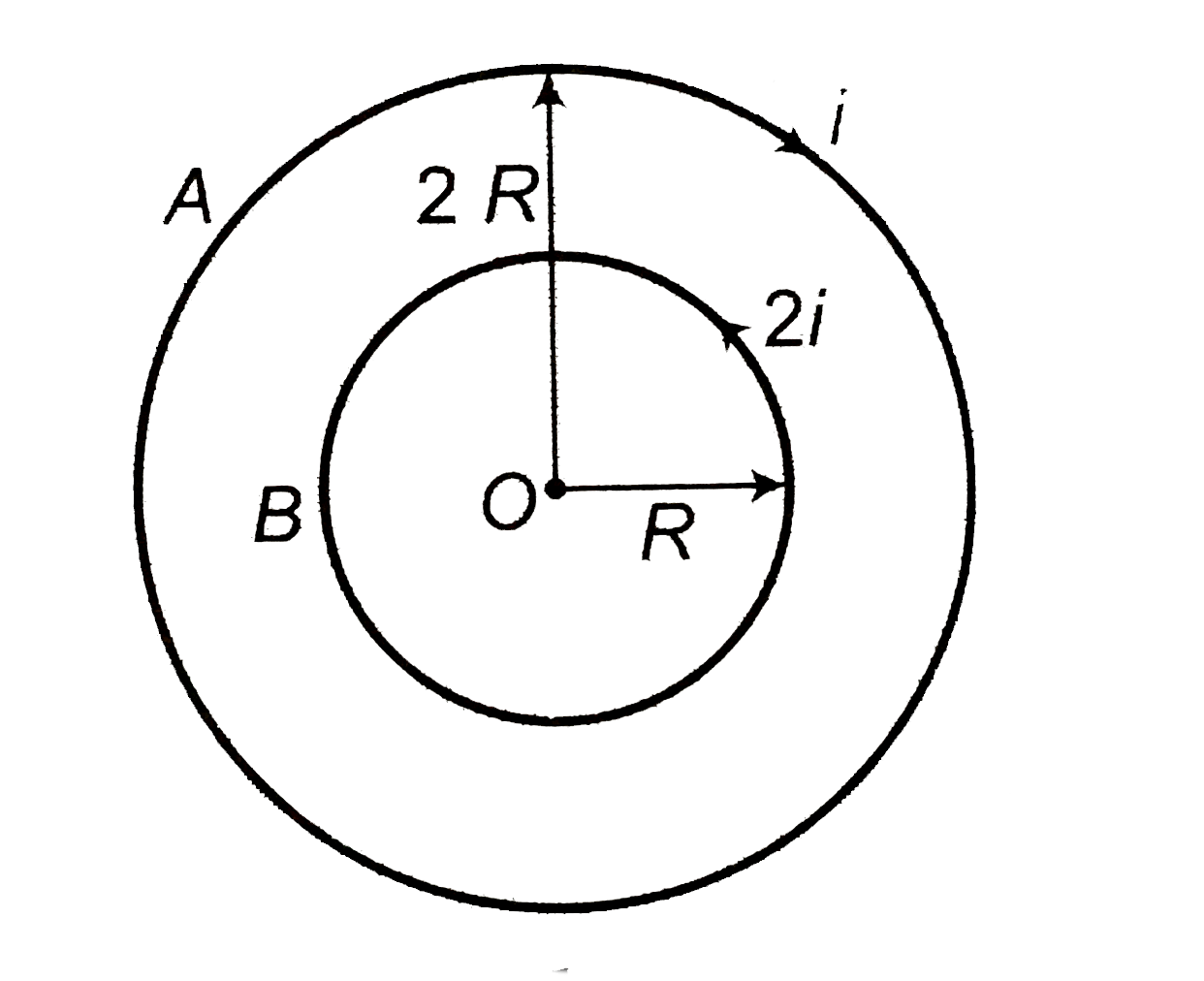
(c) In previous problem, if planes of coil are perpendicular to each other, find magnetic field at common centre.
(d) A charge q distributed uniformely over a circular ring of radius R. The ring rotates about its axis with an angular velocity ω. find the magnetic field (a) at centre and (b) at distance √3R from centre, along the axis.
View SolutionThe ratio of the magnetic field at the centre of a current carrying coil of the radius a and at distance 'a' from centre of the coil and perpendicular to the axis of coil is
View Solution
