A beam of equally charged particles after being accelerated through a voltage V enters into a magnetic field B as shown in Fig. 1.31. It is found that all the particles hit the plate between C and D. Find the ratio between the masses of the heaviest and lightest particles of the beam.

qV=12mv2⇒v=√2qVm
r=mvqB=mqB√2qVm=√2mVqB2⇒m∝r2
mheavymlight=(1+dl)2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.213videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise1.314videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Solved Example25videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank31videos
KINETIC THEORY
- BOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank50videos
Magnetism and Matter
Similar Questions
A beam of equally charged particles after being accelerated through a voltage V enters into a magnetic field 'B' as shown. It is found that all the particles hit the plate between X and Y. Find the ration between the masses of the heaviest and lightest particles of the beam.
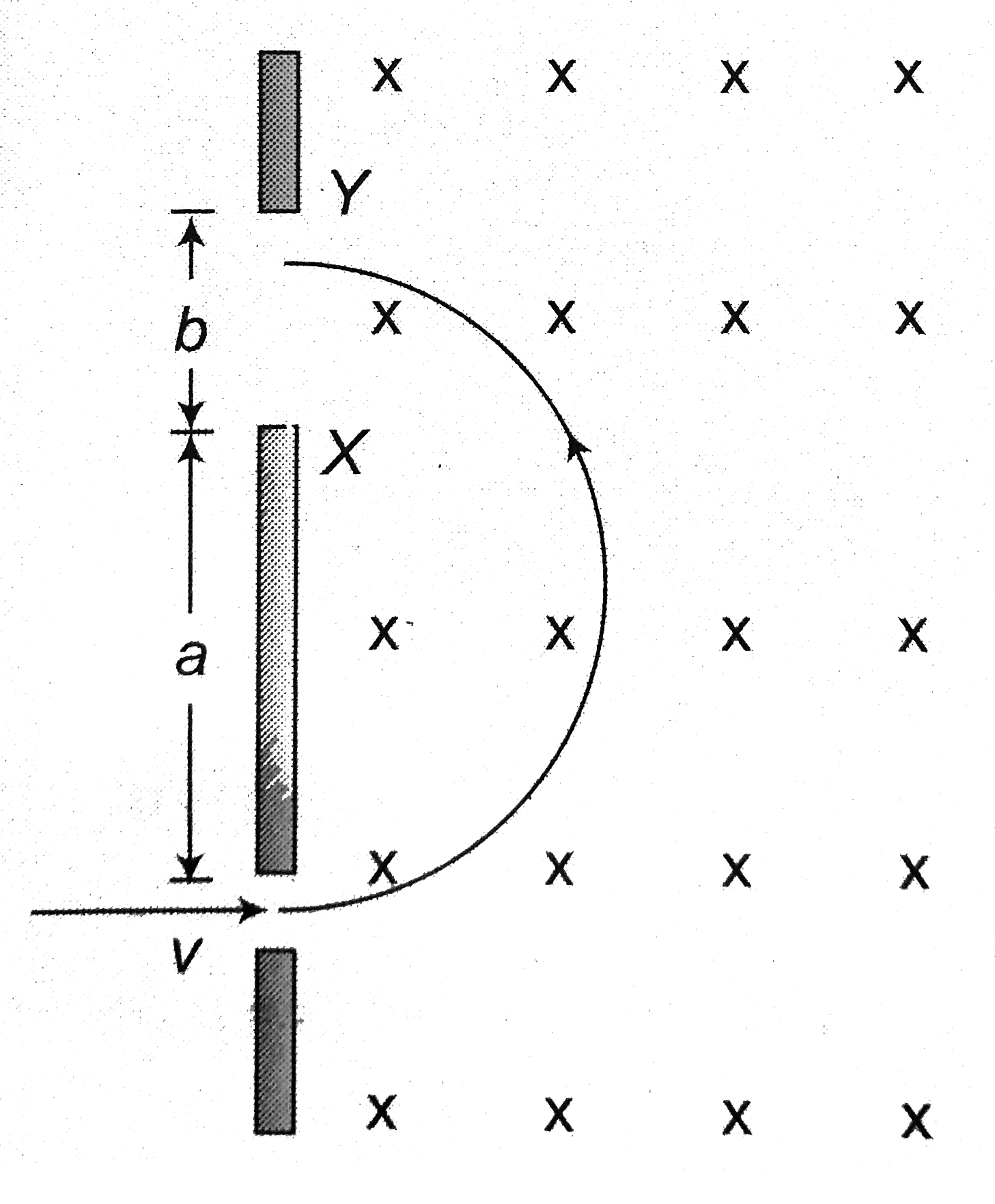 View Solution
View SolutionA positive charge particle of charge q, mass m enters into a uniform magnetic field with velocity v as shown in the figure. There is no magnetic field to the left of PQ. Find
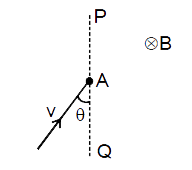
distance travelled in the magnetic fieldView Solution
Knowledge Check
- Question 1 - Select One
A charge particle after being accelerated through a potential difference V enters in a uniform magnetic field and moves in a circle of radius r. If V is doubled, the radius of the circle will become
A2rB√2rC4 rDr√2 - Question 2 - Select One
A charged particle of charge q and mass m enters a magnetic field as shown. Find radius of the circular path and the time spent inside the field. Neglect gravity.
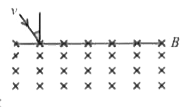 ARπθvBR(π−2θ)vCR(π+2θv)D2Rθv
ARπθvBR(π−2θ)vCR(π+2θv)D2Rθv - Question 3 - Select One
A beam of mixture of α particle and protons are accelerted through same potential difference before entering into the magnetic field of strength B. if r1=5 cm then r2 is
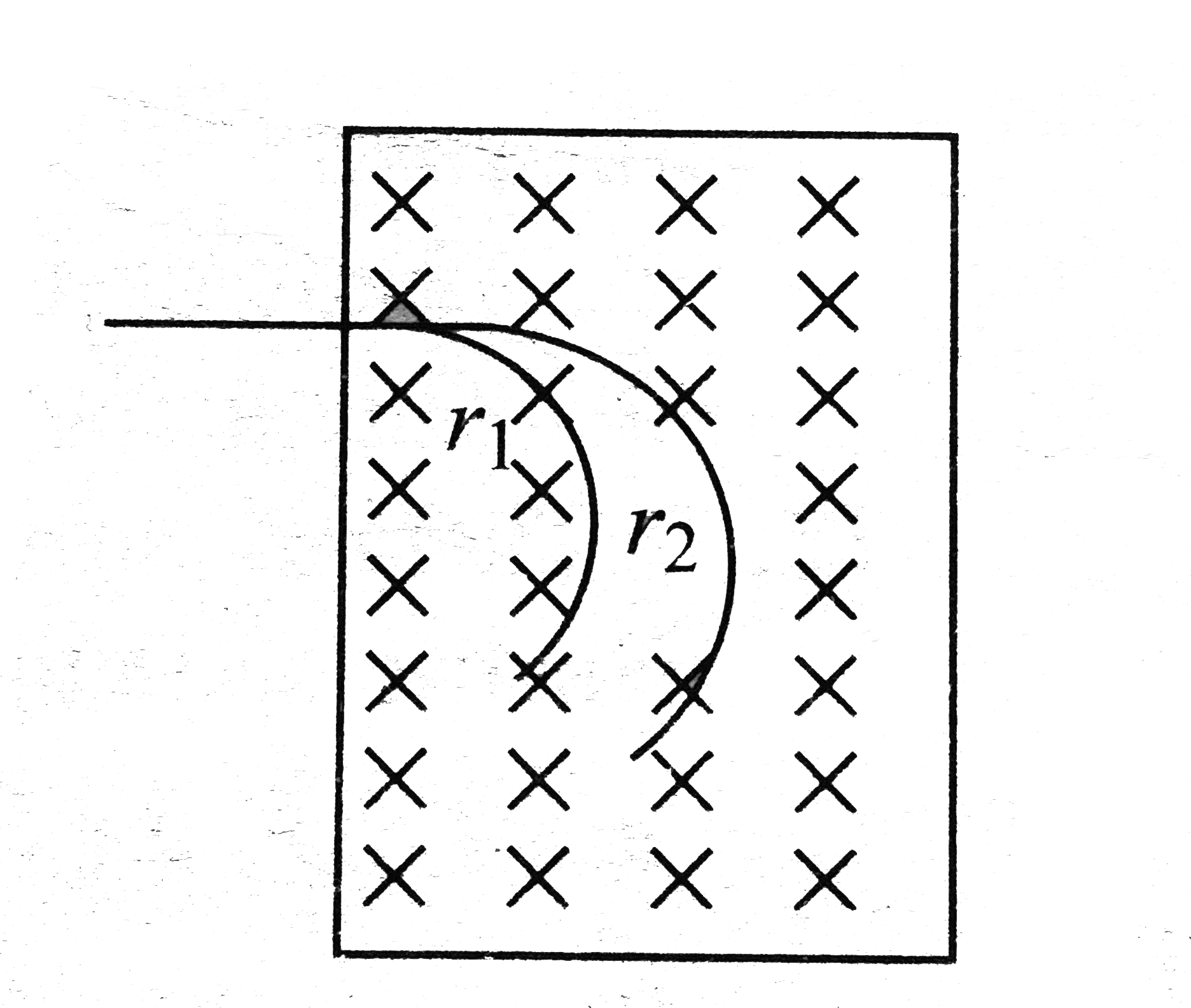 A5cmB5√2cmC10√2cmD20cm
A5cmB5√2cmC10√2cmD20cm
A positive charge particle of charge q, mass m enters into a uniform magnetic field with velocity v as shown in the figure. There is no magnetic field to the left of PQ. Find
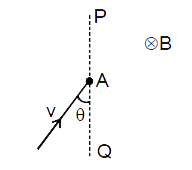
time spentView SolutionA charged particle, having charge q1 accelerated through a potential difference V enters a perpendicular magnetic field in which it experiences a force F. It V is increased to 5V, the particle will experience a force
View SolutionA charged particle, having charge q1 accelerated through a potential difference V enter a perpendicular magnetic field region in which it experiences a force F. If V is increased to 5V, the particle will experience a force
View SolutionA charged particle , having charge q accelerated through a potential difference V enters a perpendicular magnetic field in which it experiences a force F. If V is increased to 5V, the particle will experience a force.
View SolutionA proton and α particle, after accelerating through same potential difference enters into a uniform magnetic field perpendicular to their velocities, find the radius ratio of proton and α particle:-
View Solution

