A non-relativistic charge q of mass m originates at a point A lying on x-axis and moves with velocity v at an angle to the x-axis. A screen is located at a distance l from A. Find the distance r from the x-axis to the point on the screen into which the charge strikes.
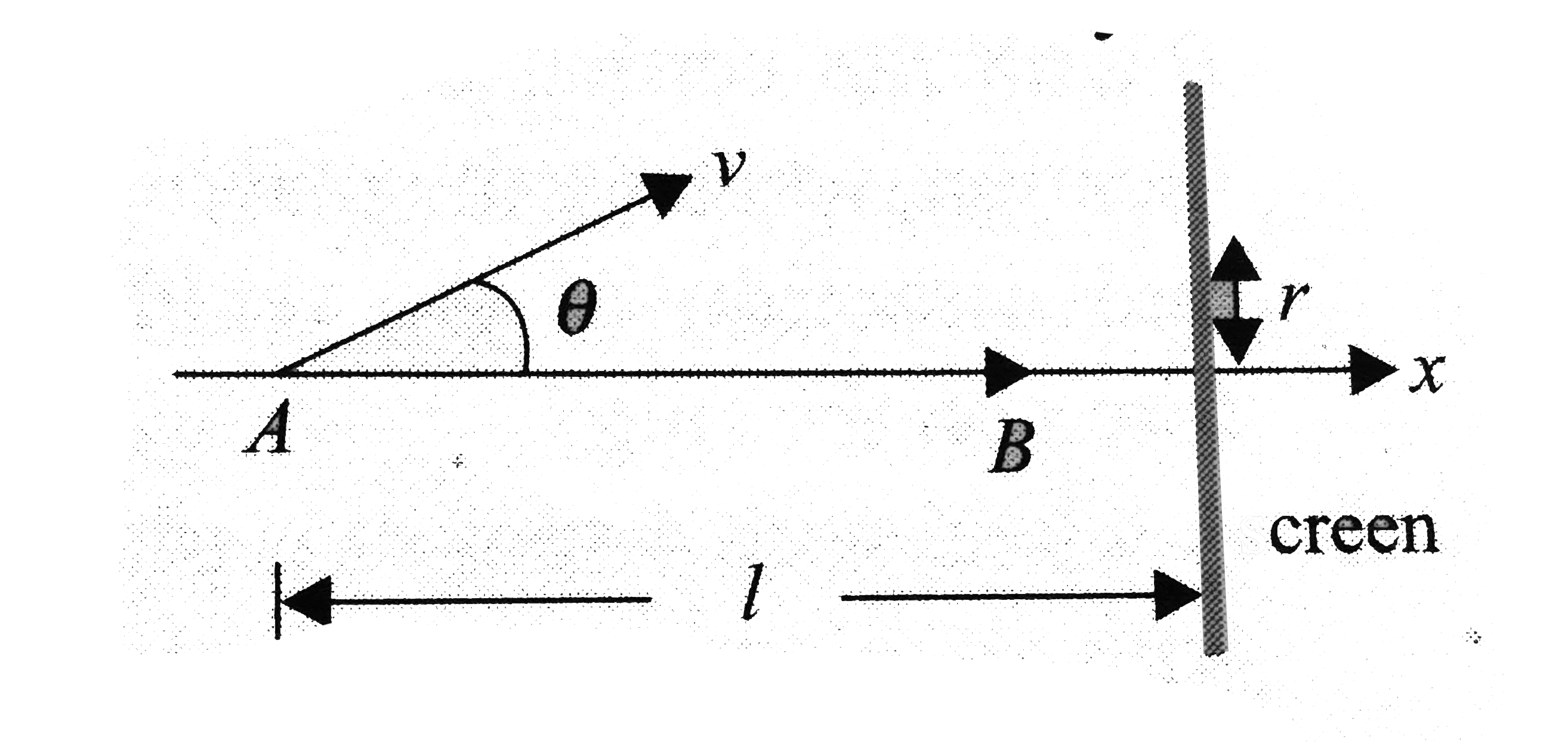
Time taken by particle to reach the screen: t=lvcosα

Radius of helical path : R=mvsinαqB
angle rotated in time t:θ=ωt=qBmt
Required distance:
r=2Rsin(θ2)=2mvsinαqBsin(qB2mlvcosα)
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.1
22 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.2
13 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple Correct Answer type
2 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
- Magnetism and MatterBOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank
50 Videos
Similar Questions
A non-relativistic electron originates at a point A lying on the axis of a striaght solenoid and moves with velocity v at an angle α to the axis. The magnetic induction of the field is equal to B. Find the distance r from the axis to the point on screen into which the electron strickes. The screeen into which the electron strikes. The screen is oriented at right angles to the axis and is located at a distance l from the point A.
View Solutionx-coordinate of a point is its distance from the x-axis.
View Solution
Knowledge Check
The distance of the point (4,3) from the X -axis is
A2B3C4D5
Find the distance of the point P(a,b,c) from the x-axis.
View SolutionFind the distance of the point P(a; b; c) from the x axis
View SolutionThe locus of a point whose distance from the x-axis is one-third of its distance from the origin is
View SolutionThe distance of the point (−3,4) from the x-axis is
View SolutionA point moves in such a way that its distance from the X-axis is twice its distance from the Y-axis. Find the locus of the point.
View SolutionFind the distance of point (8, 3) from x axis & y axis.
View SolutionThe distance of the point (3,5) from the x-axis is
View Solution
