A particle having mass $m$ and charge $q$ is released from the origin in a region in which electric field and megnetic field are given by $\to B = - B_{0} ˆ j$ and $\to E = {\to E}_{0} ˆ k$ . Find the speed of the particle as a function of its $z$ -coordinate.

Video Solution

Text Solution

Verified by Experts

In Fig shown $B_{0}$ is outward. As soon as we release the
particle at origin. Electric field will apply force $F_{e} = q E_{0}$ on the
particle. Due to this force, the charge particle will gain accelera-
tion and hence velocity along z-axis. And as it gains velocity
along z-axis, then magnetic field will apply the force $F_{b}$ on charge particle. Now both the forces are always in x-z plane, so
path of charge will be in x-z plane as shown. Let at any time its
velocity is $\to v = v_{x} ˆ i + v_{z} ˆ k$ . Let $\to a = a_{x} ˆ i + a_{z} ˆ k$ is the acceleration
at any time.

Then ${\to F}_{\neq t} = m \to a$
$\Rightarrow q E_{0} ˆ k - q (v_{x} ˆ i + v_{z} ˆ k) \times B_{0} ˆ j = m (a_{x} ˆ i + a_{z} ˆ k)$
$\Rightarrow q E_{0} ˆ k - q v_{x} B_{0} ˆ k + q v_{z} B_{0} ˆ i = m (a_{x} ˆ i + a_{z} ˆ k)$
$\Rightarrow m a_{x} = q v_{z} B_{0} and m a_{z} = q (E_{0} - v_{x} B_{0}) ... (i)$
$\Rightarrow m \frac{d v_{x}}{d t} = q v_{z} B_{0} and m \frac{d v_{z}}{d t} = q (E_{0} - v_{x} B_{0}) ... (i i)$
From Eq. (ii), $\frac{d v_{z}}{d t} = \frac{q}{m} (E_{0} - v_{x} B_{0}) \Rightarrow \frac{d^{2} v_{z}}{{d t}^{2}} = \frac{- q B_{0}}{m} \frac{d v_{x}}{d t}$
$\Rightarrow \frac{d^{2} v_{z}}{{d t}^{2}} = \frac{- q B_{0}}{m} \frac{q B_{0}}{m} v_{z} = - {(\frac{q B_{0}}{m})}^{2} v_{z}$
Solution of the above equation is : $v_{z} = v_{z 0} sin (ω t + ϕ) ... . . (i i i)$
(where $ω = \frac{q B_{0}}{m}$ )
Puting at $t = 0, v_{z} = 0, w e \geq t ϕ = 0$
Differentiating Eq. (iii),
$\frac{d v_{z}}{d t} = v_{z 0} ω cos (ω t + ϕ) \Rightarrow a_{z} = ω v_{z 0} cos ω t$
at $t = 0, a_{z} = \frac{F_{e}}{m} = \frac{q E_{0}}{m}$
$\Rightarrow \frac{q E_{0}}{m} = \frac{q B_{0}}{m} v_{z 0} {cos 0}^{\circ} \Rightarrow v_{z 0} = \frac{E_{0}}{B_{0}}$
So Eq. (iii) can be written as : $v_{z} = \frac{E_{0}}{B_{0}} sin (ω t) ... . (i v)$
Puting the value of $v_{z}$ in Eq. (ii), $m \frac{d v_{x}}{d t} = \frac{q E_{0}}{B_{0}} (sin ω t) B_{0}$
$\Rightarrow \int_{0}^{v} d v_{x} = \int_{0}^{t} \frac{q E_{0}}{m} sin ω t d t \Rightarrow v_{x} = \frac{q E_{0}}{m ω} [1 - cos ω t]$
$\Rightarrow v_{x} = \frac{q E_{0}}{m (q B_{0}) / m} [1 - cos ω t] \Rightarrow v_{x} = \frac{E_{0}}{B_{0}} [1 - cos ω t] ... . (v)$
Equations (iv) and (v) give velocity as a function of time.
From Eq.(v), $\frac{d x}{d t} = \frac{E_{0}}{B_{0}} (1 - cos ω t)$
$\Rightarrow \int_{0}^{x} d s = \frac{E_{0}}{B_{0}} \int_{0}^{t} (1 - cos ω t) d t$
$\Rightarrow x = \frac{E_{0}}{ω B_{0}} [ω t - sin ω t] ... . (v i)$
From Eq.(iv)
$\frac{d z}{d t} = \frac{E_{0}}{B_{0}} sin ω t \Rightarrow \int_{0}^{z} d z = \frac{E_{0}}{B_{0}} \int_{0}^{t} sin ω t d t$
$\Rightarrow z = \frac{E_{0}}{ω B_{0}} [1 - cos ω t] ... . (v i i)$
Equations (vi) and (vii) give the path of the verticle.
We see that at $t = 2 π$
$x = \frac{E_{0}}{B_{0}} 2 π, z = 0$

This path is known as cycloided path. This is the same kind of path as followed by a point on the rim of a purely rolling wheel, which is expalined as below.
Let a wheel is rolling purely with angular velocity $ω$ as shown.
Let at $t = 0$ , a point P on the rim is at the bottom-most position of
the wheel. After time t, the wheel turns by an angle $θ$ as shown
and point P comes at point P'. Let us find the coordinates of P'.

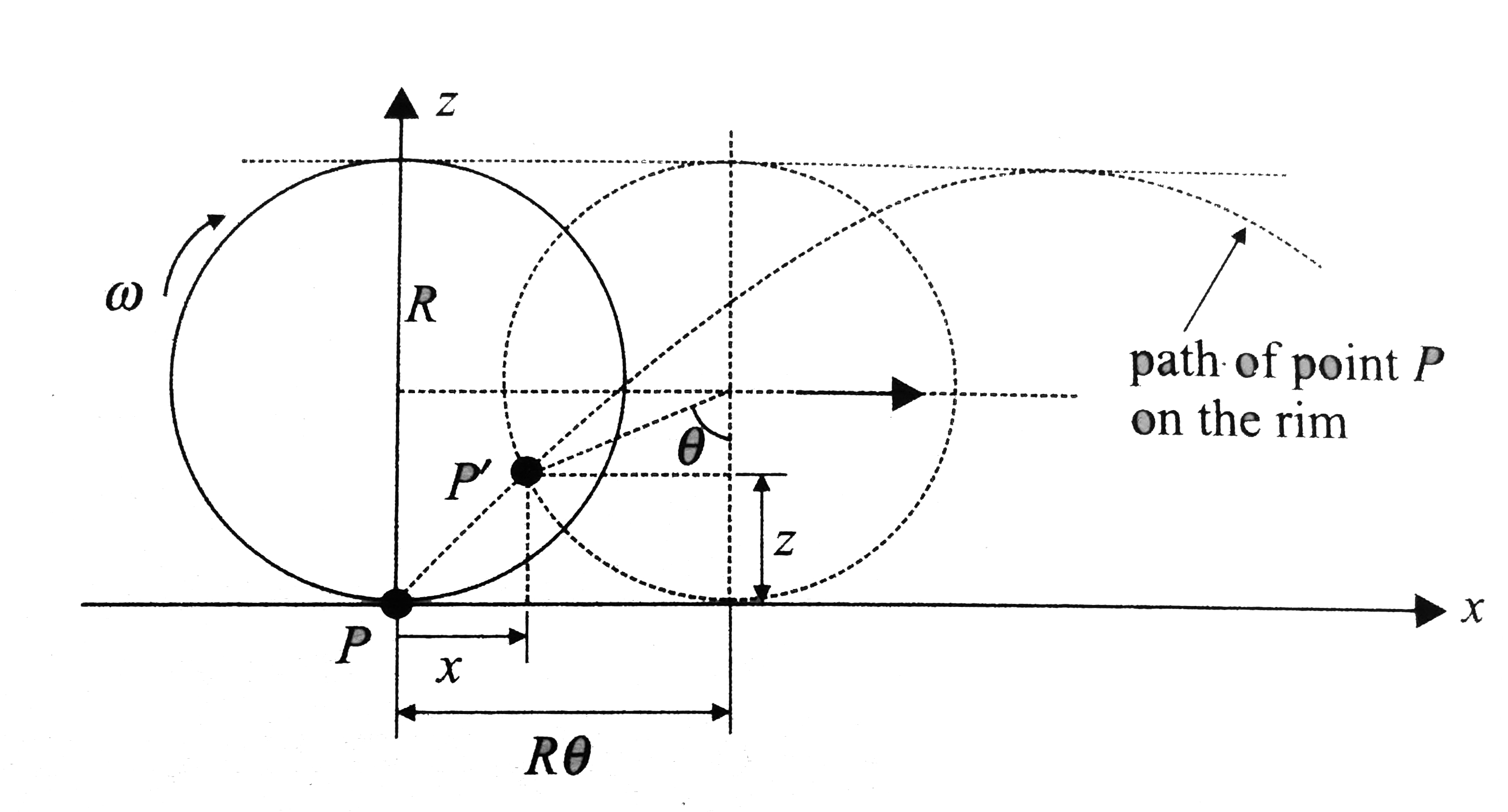
$x = R θ - R sin θ = R ω t - R sin ω t = R [ω t - sin ω t] ... (v i i i)$
$z = R - R cos θ = R [1 - cos ω t] ... . (i x)$
Equations (viii) and (ix) resemble Eqs (vi) and (vii).
Hence, the resulting path followed by charge is cycloidal.
(b) To find velocity as function of z-coordinate.
From equations (iv) and (v)
$v = \sqrt{v_{x}^{2} + v_{z}^{2}} = \frac{E_{0}}{B_{0}} \sqrt{{(1 - cos ω t)}^{2} + {(sin ω t)}^{2}}$
$= \frac{E_{0}}{B_{0}} \sqrt{{(1 - cos ω t)}^{2} + (1 - cos ω t) (1 + cos ω t)}$
$= \frac{E_{0}}{B_{0}} {\sqrt{1 - cos ω t}}^{2} = \frac{E_{0}}{B_{0}} \sqrt{\frac{z ω B_{0}}{E_{0}}} 2 [F r o m E q . (v i i)]$
$= \sqrt{\frac{E_{0}^{2}}{B_{0}^{2}} \frac{z q B_{0}}{m} \frac{B_{0}}{E_{0}} 2} = \sqrt{\frac{2 q E_{0} Z}{m}}$
Alternate Method to find velocity: Since the magnetic field does
not perform any work, therefore, whatever has been the gain in
kinetic energy it is only because of the work done by electric
field. Applying work-energy theoram, $W_{E} = Δ K$
$\Rightarrow q E_{0} z = \frac{1}{2} m v^{2} - 0 or v = \sqrt{\frac{2 q E_{0} Z}{m}}$

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Knowledge Check

A charged particle of mass m and charge q is projected on a rough horizontal XY plane. Both electric and magnetic fields are given by $\to E = - 10 ˆ k N / C$ and magnetic field $\to B = - 5 \to k$ tesla are present in the region. The particle enters into the magnetic field at $(4, 0, 0) m$ with a velocity $50 \to j m / sec$ . The particle starts into a curved path on the plane. If coefficietn of friction $μ = \frac{1}{3}$ between particle and plane, then $(q E = 2 m g, g = 10 m / s^{2})$ .
Total work done by electric force on the particle is
A $250 J$
Bzero
C $125 J$
Dnone
A charged particle of mass m and charge q is projected on a rough horizontal XY plane. Both electric and magnetic fields are given by $\to E = - 10 ˆ k N / C$ and magnetic field $\to B = - 5 \to k$ tesla are present in the region. The particle enters into the magnetic field at $(4, 0, 0) m$ with a velocity $50 \to j m / sec$ . The particle starts into a curved path on the plane. If coefficietn of friction $μ = \frac{1}{3}$ between particle and plane, then $(q E = 2 m g, g = 10 m / s^{2})$ .
The time after which particle comes to rest, is
A $5 s$
B $4 s$
C $3 s$
D $1 s$
Magnetic force on a charged particle is given by ${\to F}_{m} = q (\to v \times \to B)$ and electrostatic force ${\to F}_{e} = q \to E$ . A particle having charge q = 1C and mass 1 kg is released from rest at origin. There are electric and magnetic field given by $\to E = (10 ˆ i) N / C f or x = 1.8 m$ and $\to B = - (5 ˆ k) T$ for $1.8 m \leq x \leq 2.4 m$
A screen is placed parallel to y-z plane at $x = 3 m$ . Neglect gravity forces.
The speed with which the particle will collide the screen is
A $3 m s^{- 1}$
B $6 m / s^{- 1}$
C9 m//s^(-1)
D $12 m / s^{- 1}$

CENGAGE PHYSICS-MAGNETIC FIELD AND MAGNETIC FORCES-Solved Example

Home
Ask Doubt
Profile

A particle having mass m and charge q is released from the origin in a region in which electric field and megnetic field are given by →B=−B0ˆj and →E=→E0ˆk. Find the speed of the particle as a function of its z-coordinate.

Topper's Solved these Questions

Similar Questions

Knowledge Check

CENGAGE PHYSICS-MAGNETIC FIELD AND MAGNETIC FORCES-Solved Example

A particle having mass $m$ and charge $q$ is released from the origin in a region in which electric field and megnetic field are given by $\to B = - B_{0} ˆ j$ and $\to E = {\to E}_{0} ˆ k$ . Find the speed of the particle as a function of its $z$ -coordinate.