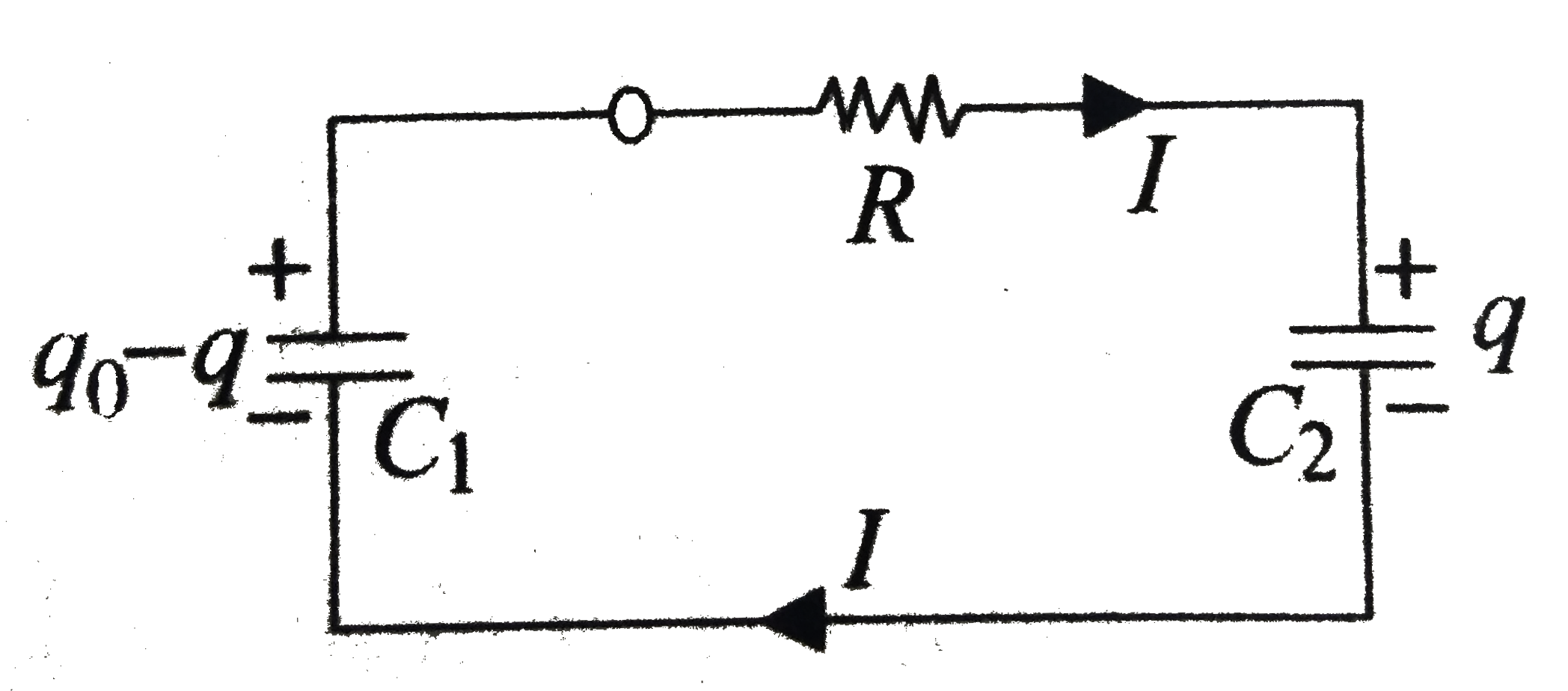
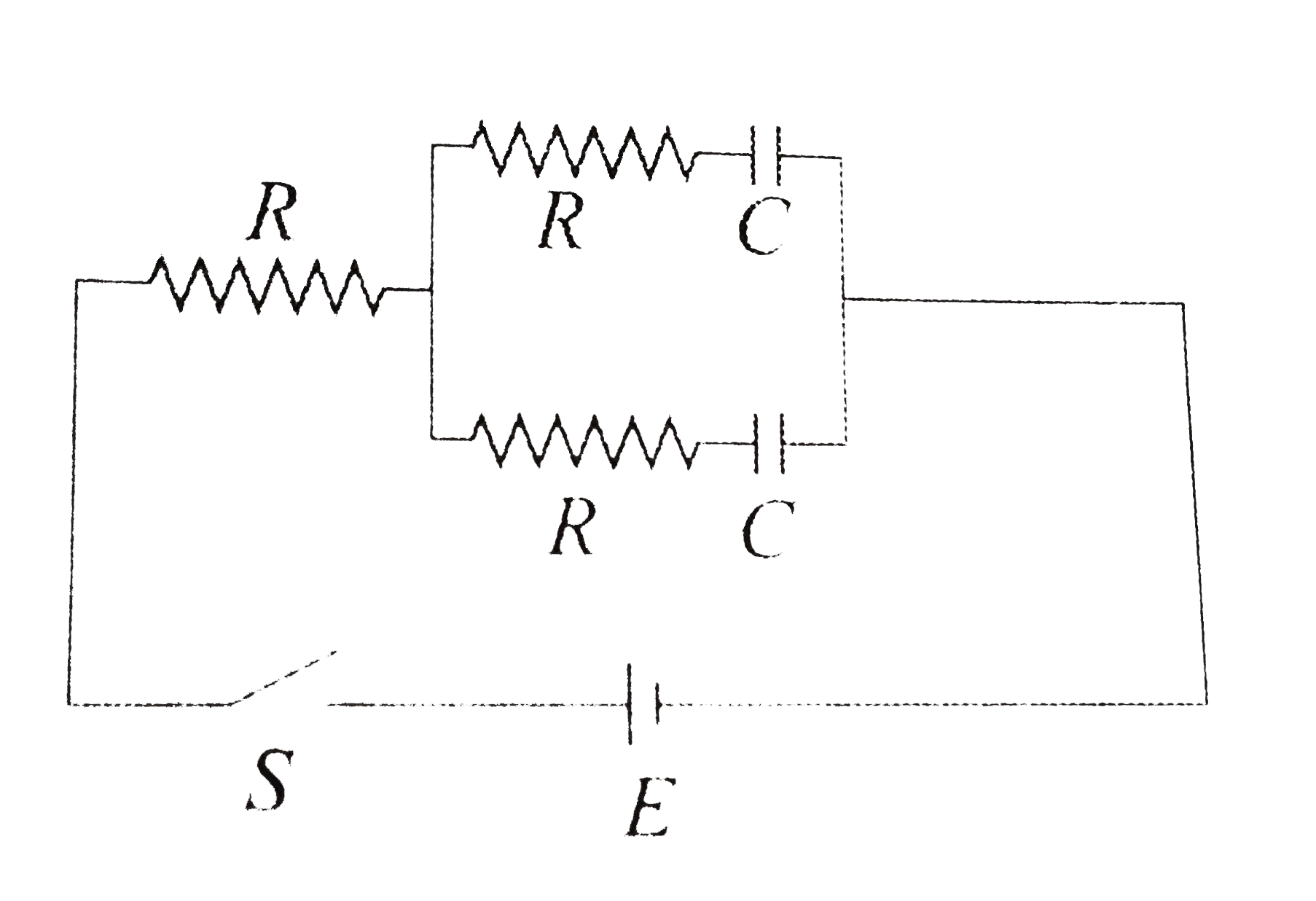
In the circuit shown, the capacitor is initially charged with charge . The switch S is closed at time t = 0 . The charge on after time t is

The correct Answer is:B
b. , apply Kirchhoff's law:
Solve to get .
Similar Questions
In the circuit shown. Initially the capacitor is uncharged. The switch S is closed at time t=0. Then
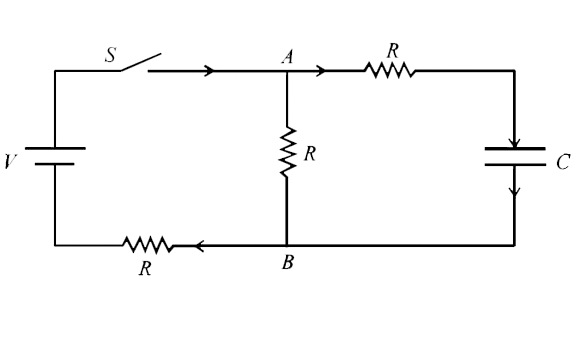
View SolutionIn the circuit shown in Figure, the battery is an ideal one, with emf V. The capacitor is initially uncharged. The switch S is closed at time t = 0 .
(a) Find the charge Q on the capacitor at time t.
(b) Find the current in AB at time t. What is its liniting value as t→∞:
 .
.
View Solution
Knowledge Check
In the circuit shown, the capacitor is initially uncharged. The switch S is closed at t = 0. Time after which voltage across capacitor and resistor are equal is:
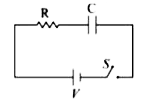 ARCln12BRCln2CRCln32DRC
ARCln12BRCln2CRCln32DRCIn the circuit shown in figure, the capacitor is charged with a cell of 5V.If the switch is closed at t=0, then at t=12s, charge on the capacitor is
 A(0.37)10μCB(0.37)210μCC(0.63)10μCD(0.63)210μC
A(0.37)10μCB(0.37)210μCC(0.63)10μCD(0.63)210μCIn the circuit shown, the capacitor initially charged with a 12V batteryy, when switch S1 is open and switch S2 is closed. The maximum value of current in the circuit when S2 is opened and S2 is closed is
 A10−6AB7.2μAC720μAD360μA
A10−6AB7.2μAC720μAD360μA
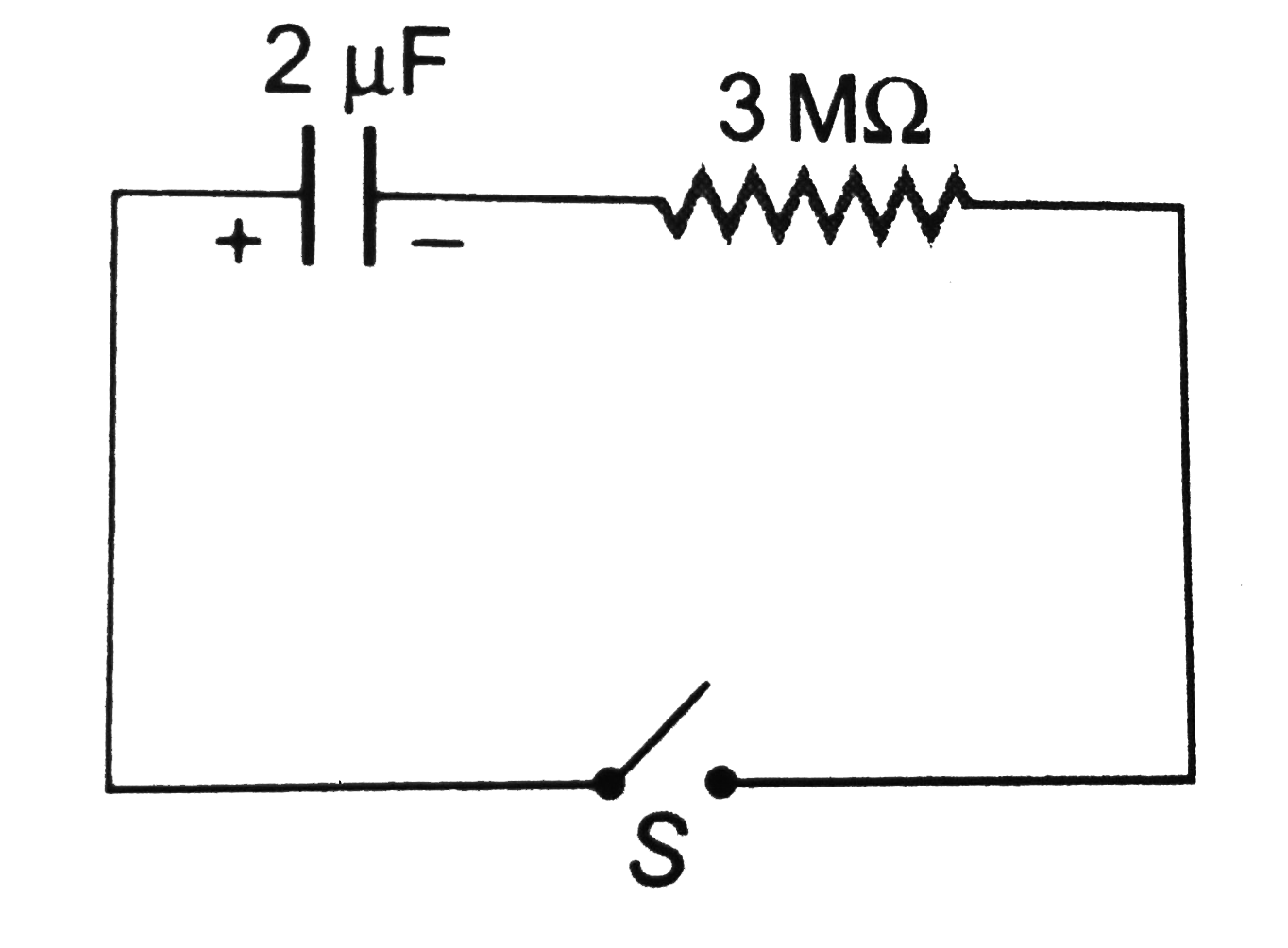
In the circuit shown in figure-3.244, the battery is an ideal one with emf V. The capacitor is initially uncharged. The switch S is closed at time t=0.
(a) Find the charge Q on the capacitor at time l
(b) Find the current in branch AB at time just after closing the switch.
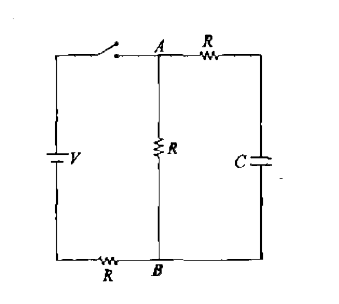
View SolutionThe plates of a capacitor of capacitance C are given the charges Q1andQ2 as shwon in figure. Now the switch is closed at t =0. Find the charges on plates after time t.
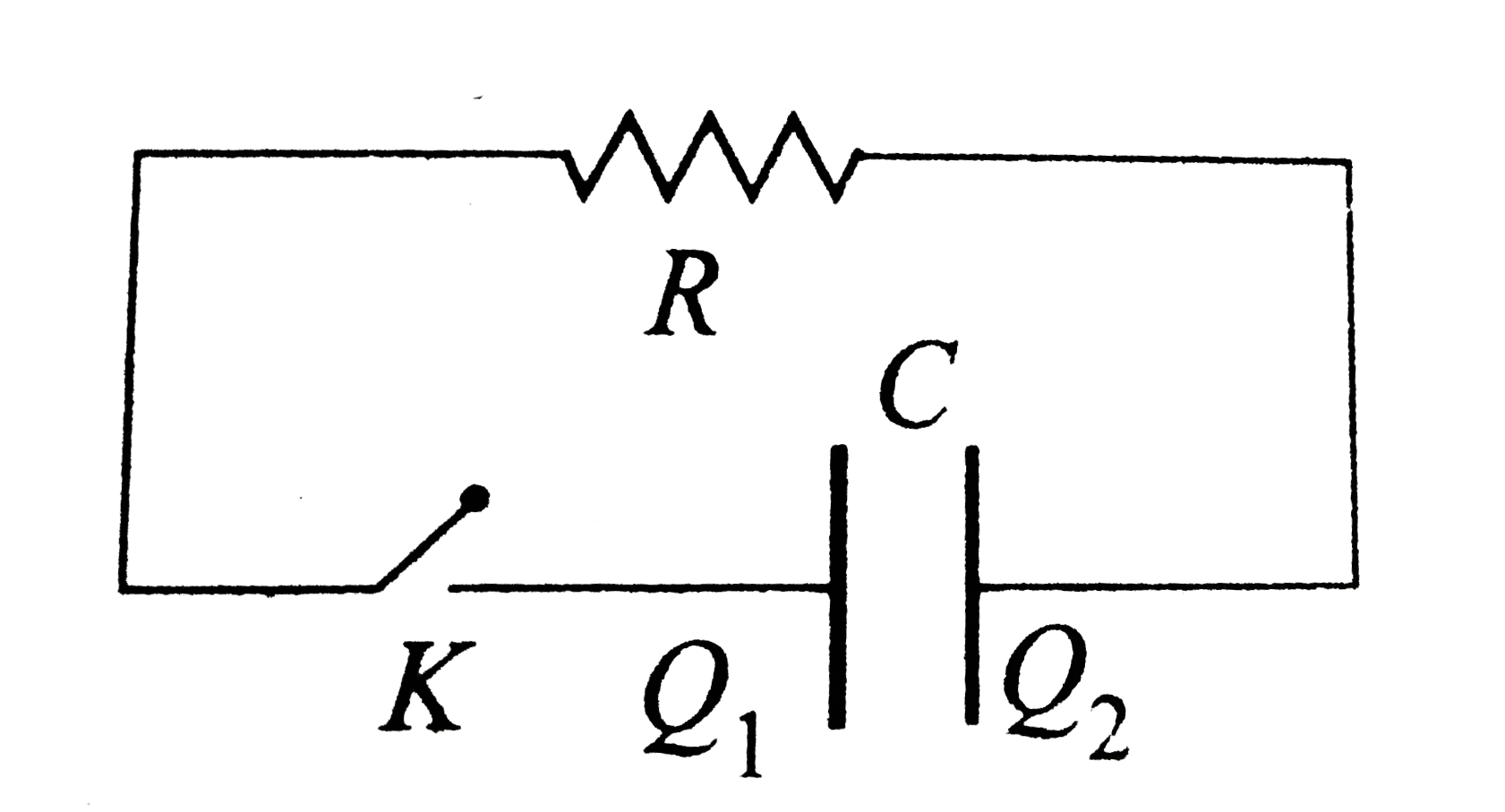
View SolutionIn given circuit, switch S is closed at t=0 . The charge on the capacitor ar steady state will be
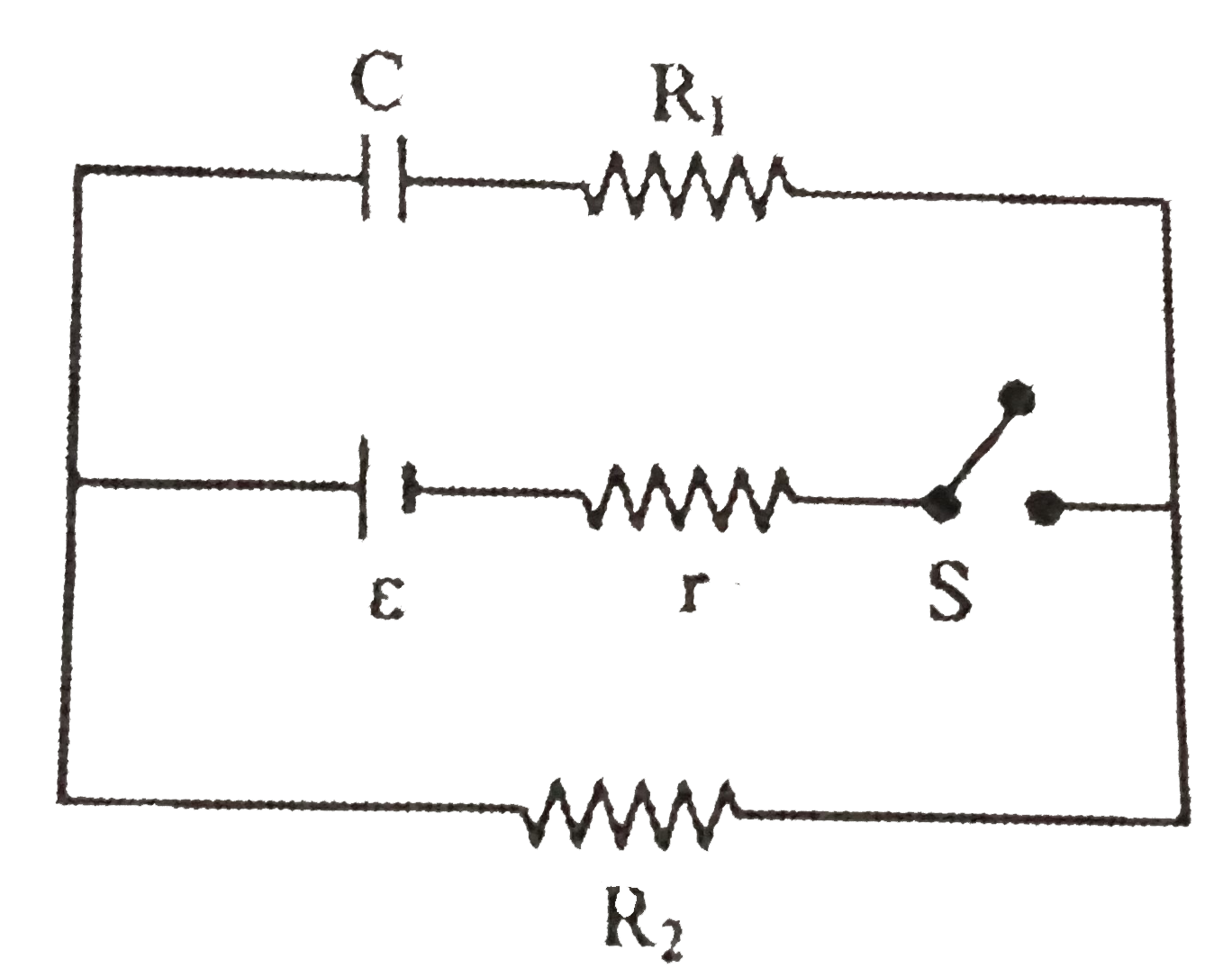
View SolutionThe switch S in the circuit diagram is closed at t = 0. The charge on capacitors at any time is
View SolutionThe switch shown in figure is closed at t=0 find the charge throuh battery till time t=LR
_2019_CBT8_E01_016_Q01.png)
View Solution
